If the sum of lengths of hypotenuse and a side of a right angled triangle is given, show that area of triangle is maximum, when the angle between them is π3.
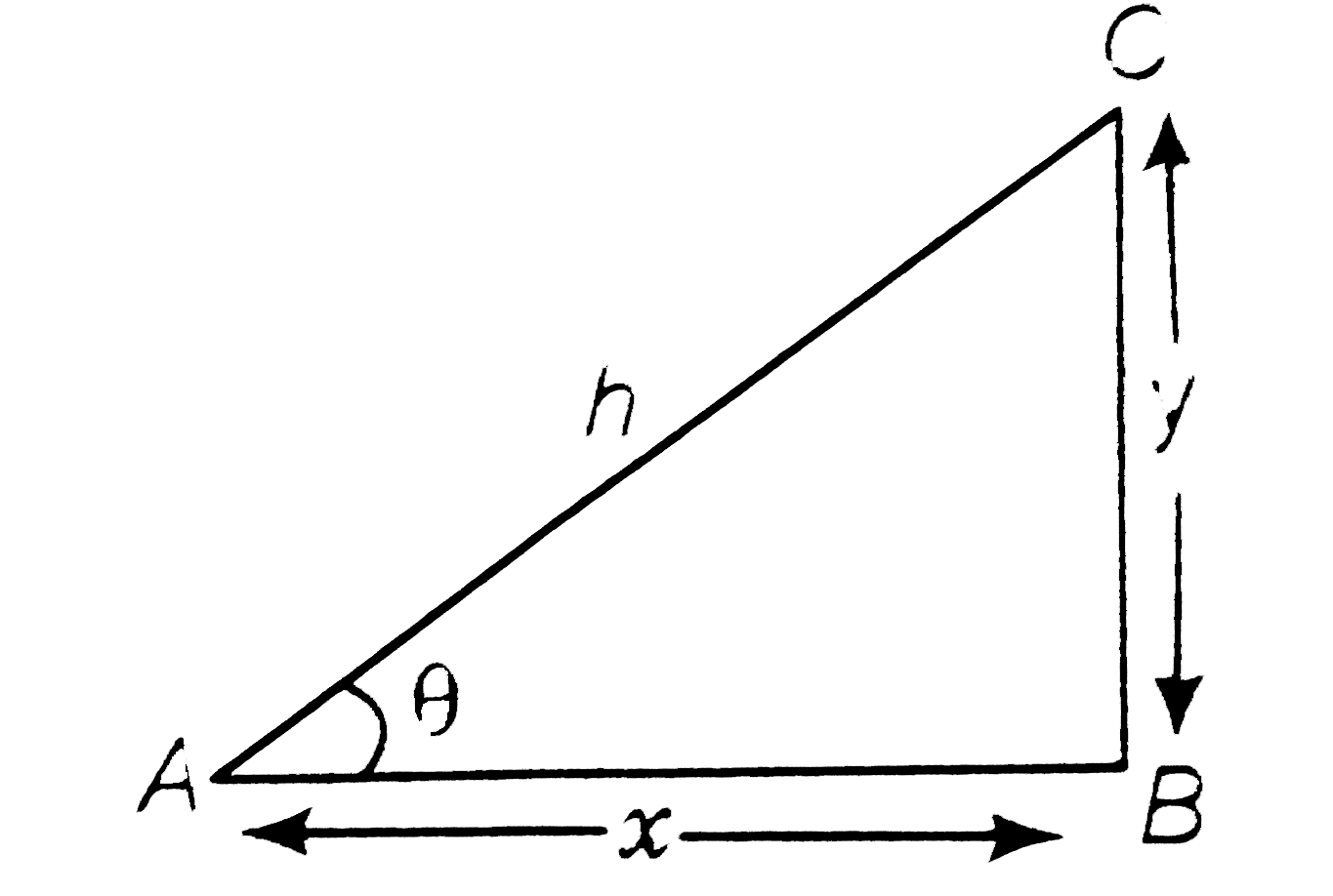
Let ABC be a triangle with AC =h, AB=x and BC=y.
Also, ∠CAB=θ
Let h+x=k
cosθ=xh
⇒x=hcosθ [Using eq.]
⇒h+hcosθ=k
⇒h(1+cosθ)=k ...............(i)
Also, area of ΔABC=12(AB.BC)
A=12.x.y
=12hcosθ.hsinθ [∴sinθ=yn]
=12h2sinθ.cosθ
=2h24sinθ.cosθ
Since, h=k1+cosθ..............(ii)
∴A=14(k1+cosθ)
A=14(k1+cosθ)2.sin2θ
⇒A=k24.sin2θ(1+cosθ)2.............(iv)
∴dA(d)θ=k24⎡⎢
⎢
⎢
⎢
⎢⎣(1+cosθ)2.cos2θ.2−sin2θ.2(1+cosθ).(0−sinθ)(1+cosθ)4⎤⎥
⎥
⎥
⎥
⎥⎦
k24{(2)(1+cosθ)[(1+cosθ).cos2θ+sin2θ(sinθ))(1+cosθ)4}
(k24).2(1+cosθ)3[(1+cosθ).cos2θ+2sin2θ.cosθ]
=k22(1+cosθ)[(1+cosθ)(1−2sin2θ)+2sin2θ.cosθ]
=k22(1+cosθ)2[1+cosθ−2sin2θ−2sin2θ.cosθ+2sin2θ.cosθ]
k22(1+cosθ)3[(1+cosθ)−2sin2θ]
=k22(1+cosθ)3[(1+cosθ)=2sin2θ]
=k22(1+cosθ)3(2cos2θ+cosθ−1)............(v)
For dA(d)θ=0,
k22(1+cosθ)3(2cos2θ+cosθ−1)=0
⇒2cos2θ+2cosθ−cosθ−1=0
⇒2cosθ(cosθ+1)−1(cosθ+1)=0
⇒(2cosθ−1)(cosθ+1)=0
⇒cosθ=12orcosθ=−1
⇒θ=π3 [positive]
or θ=2nπ±π [not possible]
∴θ=π3
Again, differentiating w.r.t θ in Eq. (v), we get
dd(θ)dAd(θ)=dd(θ)[k22(1+cosθ)3(2cos2θ+cosθ−1)]
∴d2Ad(θ)2=dd(θ)[(k2(2cosθ−1))(1+cosθ)(2(1+cosθ))3]=dd(θ)[k22.2cosθ−1(1+cosθ)2]
=k22⎡⎢
⎢
⎢
⎢⎣(1+cosθ)(1+cosθ)(−2sinθ)+2sinθ(2cosθ−1)(1+cosθ)2⎤⎥
⎥
⎥
⎥⎦
=k22[−2sinθ−2sinθ.cosθ+4sinθ.cosθ−2sinθ(1+cosθ)4]
=k22[−4sinθ−sin2θ+2sin2θ(1+cosθ)3]=k22[sin2θ−4sinθ(1+cosθ)3]
d2A(d(θ)2)atθ=π3=k22⎡⎢
⎢
⎢⎣sin2π3−4sinπ3(1+cosπ3)3⎤⎥
⎥
⎥⎦=k22⎡⎢
⎢
⎢⎣√32−4√32(1+12)3⎤⎥
⎥
⎥⎦
=k22[−3√3.82.27]=−k22√39
Which is less than zero.
Hence, area of the right angled triangle is maximum, when the angle between them is π3.
Related Playlists
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - OBJECTIVE TYPES QUESTIONS25videos
APPLICATION OF DERIVATIVES
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Fillers5videos
APPLICATION OF DERIVATIVES
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Fillers5videos
APPLICATION OF DERIVATIVES
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - Objective Type Questions22videos
APPLICATION OF INTEGRALS
Similar Practice Problems
- Question 1 - Select One
If the sum of two sides, other than hypotenuse of a right-angled triangle is 17cm and the perimeter is 30cm, then the lengths of two sides are:
A7cm, 10cmB4cm, 13cmC5cm, 12cmD6cm, 11cm
Similar Questions
If the sum of the lengths of the hypotenuse and another side of a right-angled triangle is given, show that the area of the triangle is maximum when the angle between these sides is π3.
View SolutionIf the sum of the lengths of the hypotenuse and another side of a right-angled triangle is given, show that the area of the triangle is maximum when the angle between these sides is π3.
View SolutionIn the right triangle BAC,∠A=π2anda+b=8. The area of the triangle is maximum when ∠C , is
View SolutionProve that the area of right-angled triangle of given hypotenuse is maximum when the triangle is isosceles.
View SolutionProve that the area of right-angled triangle of given hypotenuse is maximum when the triangle is isosceles.
View SolutionIf the length of sides of right angled triangle are in AP, then their ratio is
View SolutionThe hypotenuse of a right angled triangle is 45 cm long. If one of the legs of this triangle is 36 cm, then find the length of other leg.
View SolutionThe lengths of the medians through acute angles of a right-angled triangle are 3 and 4. Find the area of the triangle.
View SolutionDraw an isosceles triangle in which each of the equal sides is of length 3 cm and the angle between them is450.
View Solution

