Determine the moment of inertia Θa of a homogeneous torus with a circular cross section and mass m.
The mass moment of inertia Θa is determined fromWe can determine the geometrical relationsr = R + c sinϕ dm = ρ 2πr2c cosϕ dr from the figure. With dr = c cosϕ dϕwe obtainNote that the integrals of the odd functions over the even interval are zero. Using m = 2π2ρc2R we finally getThis result reduces to Θa = mR2 in the case of a thin ring c ≪ R.
Related Solutions
The moment of inertia of a circular ring of radius r and mass M about diameter is
View SolutionDeduce expression for moment of inertia about a vertical axis through the center of gravity and perpendicular to the cross section for uniform disc of mass 'm' and radius 'r'.
View SolutionIn designing wooden girder bridges we often have to deal with logs flattened on two opposite sides. Figure 111. shows the cross-section of such a log. Determine the moment of inertia of this cross-section about the horizontal centre line.
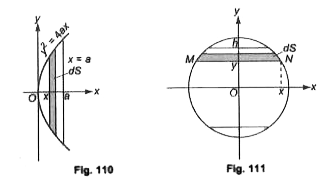
View SolutionFind the moment of inertia of a section of circular disc of radius R about an axis perpendicualr to its plane as shown in the figure. The section has mass m and subtends an angle at the centre.
View SolutionThe moment of inertia of a circular disc of mass m and radius r about an perpendicular axis passing through its centre is
View SolutionMoment of inertia of a uniform circular ring of mass and radius about a diameter of ring is………………. .
View Solutionलम्बाई L तथा द्रव्यमान M की एक छड़ को अर्द्धवृत्तीय रूप में मोड़ा जाता है (चित्रानुसार) | XY के परितः जड़त्व आघूर्ण है

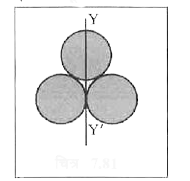
View SolutionM द्रव्यमान तथा R त्रिज्या के तीन वृत्ताकार डिस्कों को चित्रानुसार व्यवस्थित किया गया है। YY' अक्ष के परितः निकाय का जड़त्व आघूर्ण ज्ञात कीजिये।
View Solutionत्रिज्या R , द्रव्यमान M व जड़त्व आघूर्ण l का एक एकसमान गोल पिण्ड क्षैतिज से कोण पर झुके समतल पर लुढ़कता है इसका त्वरण है
View SolutionMoment of inertia of a circular ring of mass m and radius r about the normal axis passing through its centre is
View SolutionThe moment of inertia of a thin circular disc of mass M and radius R about any diameter is
View SolutionM द्रव्यमान और R त्रिज्या का एक वृत्तीय छल्ला (Ring ) दीवार में लगी खूँटी से लटकाया गया है। खूँटी के सापेक्ष इस छल्ले का जड़त्व आघूर्ण कितना होगा?
View SolutionThe moment of inertia of a thin circular disc of mass M and radius R about any diameter is
View SolutionThe moment of inertia of a uniform circular disc of mass M and of radius R about one of its diameter is
View SolutionMoment of inertia of a circular wire of mass M and radius R about its diameter is :
View SolutionA wire of mass 'm' and length 'l' is bent in the form of a circular ring. The moment of inertia of the ring about its axis is-
View SolutionMoment of inertia of a uniform circular ring of mass 2 kg and diameter 1 m about its diameter is
View SolutionA wire of mass m and length l is bent in the form of circular ring. The moment of inertia of the ring about its axis is
View SolutionA wire of length l and mass M in the form of a circular ring The moment of inertia of the ring about its axis is
View Solutionद्रव्यमान M तथा त्रिज्या R की एक वृत्तीय चकती का चकती के किनारे से गुजरने वाली तथा चकती के तल के लम्बवत् अक्ष के परितः जड़त्व आघूर्ण होता है :
View Solution