फलन f(θ)=4(sin2θ+cos4θ) पर विचार कीजिए ।
निम्नलिखित कथनो पर विचार कीजिए ।
I. f(θ)=2 का कोई हल नहीं है ।
II. f(θ)=72 का हल नहीं है ।
उपरोक्त कथनो में से कौन- सा /से कथन सही है /है ?
केवल I
केवल II
I और II दोनों
न तो I और न ही II
The correct Answer is:c
दिया है , f(θ)=4(sin2θ+cos4θ)
तथा f(θ)=4(sin2θ+cos2θ)
∴4{1−cos2θ2+(1+cos2θ2)2}
=4{1−cos2θ2+1+cos22θ+2cos2θ4}
=4{2−2cos2θ+1+cos22θ+2cos2θ4}
=4{3+cos22θ4}=3+cos22θ
f(θ) अधिकतम =3+1=4
f(θ) न्यूनतम =3+0=3
=4[sin2θ+(cos2θ)2]
=4[sin2thetea+(1−sin2θ)2]
=4[sin2θ+1+sin4θ−2sin2θ]
=4[sin4θ−sin2θ+1]
I . जब f(θ)=2
⇒4(sin2θ−sin2θ+1)=2
⇒sin6(4)θ−sin2θ+1=12
⇒sin4θ−sin2θ+12=0
⇒2sin4θ−2sin2θ+1=0
माना sin2θ=x⇒2x2−2x2−2x+1=0
अब , b2−4ac=(−2)2−4×2×1
=4−8=−4<0
⇒f(θ)=2 का कोई हल नहीं है
II. जब f(θ)=72
⇒4(sin4θ−sin2θ+1)=72
⇒sin2θ−sin2θ+1=78
⇒sin2θ−8sin2θ+1=0
माना sin2θ=x⇒8x2−8x+1=0
⇒b2−4ac=(−8)2−4×8×1
=64−32=32>0
⇒x=−(−8)±√322×8
⇒x=8±4√416=2±√24
⇒sin2θ=2±√24
ऋणात्मक लेने पर, sinθ=√2−√22=sin221∘2
⇒θ=221∘2⇒f(θ)=72 का एक हल होगा ।
धनात्मक लेने पर , sinθ=√2+√22<1
अतः दोनों कथन सही है ।
Topper's Solved these Questions
- कोणों का मापन तथा त्रिकोणमितीय अनुपात BOOK - NDA PATHFINDERCHAPTER - कोणों का मापन तथा त्रिकोणमितीय अनुपात EXERCISE - अभ्यास प्रश्नावली
40 Videos
- आव्यूह BOOK - NDA PATHFINDERCHAPTER - आव्यूह EXERCISE - अभ्यास प्रश्नावली (विगत वर्षों के प्रश्न )
21 Videos
- क्रमचय एवं संचय BOOK - NDA PATHFINDERCHAPTER - क्रमचय एवं संचय EXERCISE - विगत वर्षो के प्रश्न
23 Videos
Similar Questions
1secA−tanA−1cosA का मान है
View Solutionगुणनफल [x y z]⎡⎢⎣a h gh b fg f c⎤⎥⎦⎡⎢⎣xyz⎤⎥⎦ की कोटि क्या है ?
View Solution4 लड़को और 3 लड़कियों को कितने तरीको से बैठाया जा सकता है कि लड़के और लड़कियाँ एकान्तर रूप से बैठे ?
View Solutionसमीकरण secθ−cosecθ = 4/3 का हल है
View Solutionयदि xcosθ+ysinθ=2 और xcosθ−ysinθ=0 हो, तब निम्नलिखित में से कौन-सा एक सही है?
View Solutionनिम्नलिखित में से कौन सा कथन सही नहीं है?
View Solutionनिम्नलिखित में से कौन सा कथन सही नहीं है?
View Solutionनिम्नलिखित में से कौन सा कथन सही नहीं है?
View Solutionनिम्नलिखित यौगिक (A) पर विचार कीजिए। सही कथन का चयन कीजिए।
View Solutionदिए गए कथन में से कौन सा सही नहीं है?
View Solutionनिम्नलिखित में से कौन सा कथन RS^(Theta) और RO^(Theta) के संदर्भ में सही है?
View Solutionनिम्नलिखित में से कौन सा कथन सत्य नहीं है?
View Solutionनिम्नलिखित में से कौन सा कथन सही नहीं है?
View Solutionनिम्नलिखित में से कौन सा कथन सही नहीं है?
View Solutionनिम्नलिखित में से कौन सा कथन सत्य नहीं है?
View Solutionक्वांटम संख्या के निम्नलिखित समुच्चयों पर विचार कीजिए:
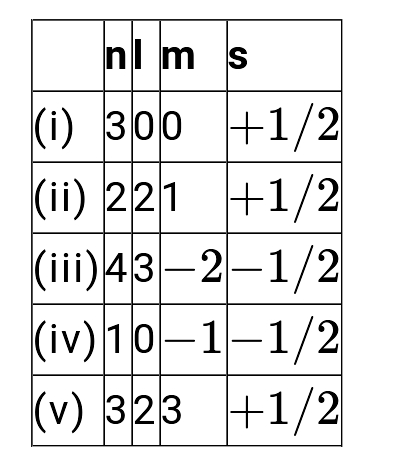
निम्नलिखित में से क्वांटम संख्या का कौन सा समुच्चय संभव नहीं है?
View Solution
