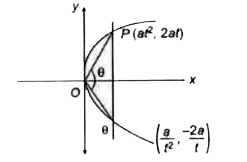
माना कि PQ परवलय की एक नाभीय जीवा है। बिन्दुओं P तथा Q पर परवलय की स्पर्श रेखाएँ एक बिन्दु पर मिलती हैं जो कि रेखा पर स्थित है।
यदि जीवा के शीर्ष पर कोण अंतरित करती है तब
The correct Answer is:D
जहाँ
Topper's Solved these Questions
- परवलयBOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - परवलयEXERCISE - मैट्रिक्स-सुमेल प्रकार
2 Videos
- परवलयBOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - परवलयEXERCISE - दृढ़कथन-कारण प्रकार
2 Videos
- परवलयBOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - परवलयEXERCISE - रिक्त स्थानों की पूर्ति कीजिये
1 Videos
- निश्चित समाकलन BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - निश्चित समाकलन EXERCISE - एकल पूर्णाक प्रश्न
7 Videos
- प्रतिलोम वृत्तीय फलन BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - प्रतिलोम वृत्तीय फलन EXERCISE - एकल पूर्णांक प्रश्न
1 Videos
Similar Questions
y2=4x पर अभिलम्ब खींचे गए, तो प्र प्रतिछेद करते है तब

View Solutionप्रत्येक प्रश्न में वक्तव्य I(कथन ) और वक्तव्य II (कारण) दिए गए हैं। प्रश्न में चार विकल्प (a), (b), (c) तथा (d) है , जिनमें से केवल एक सही है।
वक्तव्य I वक्र y=−x22+x+1 रेखा x=1 के परितः सममित (symmectric) है
क्योंकि
वक्तव्य II परवलय अपने अक्ष के परित: सममित है।
View Solutionपरवलय y2=4x के नाभिलम्ब (latusrectum) के सिरों पर खींची गयी स्पर्शी का प्रतिछेद बिंदु .............है
View Solutionदिया है कि किसी वास्तविक संख्या x के लिए [x], अधिकतम पूर्णांक को दर्शाता है यदि अन्तराल [−10,10] पर वास्तविक मानों वाला फलन f निम्न प्रकार से परिभाषित है f(x)={x−[x],if [x] is Odd,1+[x]−x, if [x] is Even तो π210∫10−10f(x)cosπxdx का मान है
View Solutionमाना f:[0,4π]→[0,π],f(x)=cos−1(cosx) के द्वारा परिभाषित है। तब x∈[0,4π] में समीकरण f(x)=10−x10 को संतुष्ट करने वाले बिंदुओं की संख्या है
View Solutionपरवलय y2=4ax की नाभि तथा उस पर स्थित चर को मिलाने वाले रेखाखण्ड के मध्य - बिंदु का पथ एक परवलय है जिसकी नियता है :
View Solutionएक परवलय की नाभि मूलबिंदु पर है तथा रेखा x=2 परवलय की नियता है , तो परवलय का शीर्ष है
View Solutionएक परवलय के नाभिलम्ब का समीकरण x+y=8 तथा उसके शीर्ष पर स्पर्शी का समीकरण x+y=12 है, तो उसके नाभिलम्ब की लम्बाई है :
View Solutionकिसी वृत्त की जीवा AB द्वारा केंद्र पर अंतरित कोण 140∘ है तो A तथा B बिन्दुओ पर खींची गयी स्पर्श रेखाओ के बीच का कोण ज्ञात कीजिए।
View Solutionकिसी वृत्त की जीवा AB द्वारा केंद्र पर अंतरित कोण 140∘ है तो A तथा B बिन्दुओ पर खींची गयी स्पर्श रेखाओ के बीच का कोण ज्ञात कीजिए।
View Solutionमाना एक ऊर्ध्वाधर मीनार AB ऐसी है कि उसका सिरा A भूमि पर है। माना AB का मध्य-बिन्दु C है और भूमि पर स्थित बिन्दु P ऐसा है कि AP=2AB यदि ∠BPC=β है, तो tanβ बराबर है
View Solutionयदि वक्रों y=10−x2 तथा y=2+x2 के बीच एक प्रतिच्छेदन बिन्दु पर न्यून कोण θ है , तो |tanθ| बराबर है
View Solutionयदि एक त्रिभुज , जिसका एक शीर्ष परवलय के शीर्ष परवलय y2+4(x−a2)=0 तथा अन्य दोनों शीर्ष y-अक्ष तथा परवलय के प्रतिच्छेदन बिंदुओं पर हैं , का क्षेत्रफल 250 वर्ग इकाई है , तो 'a' का एक मान है
View Solutionपरवलय x2=8y पर एक स्पर्श रेखा,जो X-अक्ष की धनात्मक दिशा के साथ कोण θ बनती है, का सभीकरण है
View Solutionपरवलय y2=2x की स्पर्श रेखा 18x-6y+1= 0 का स्पर्श बिन्दु है
View Solutionपरवलय y2=36x पर स्थित वे बिन्दु जिनकी कोटि भुज की तिगुनी है, हैं
View Solution
