i1/3 का मान है (1)√3+i2 (2)√3−i2 (3)1+i√32 (4)1−i√32
√3+i2
√3−i2
1+i√32
1−i√32
The correct Answer is:A
Step by step video, text & image solution for i^(1//3) का मान है (1)(sqrt3+i)/2 (2)(sqrt3-i)/2 (3)(1+isqrt3)/2 (4)(1-isqrt3)/2 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ERRORLESS HINDICHAPTER - सम्मिश्र संख्यायेंEXERCISE - Solved Multiple Choice Questions (आयोटा की पूर्णांक घातें, सम्मिश्र संख्याओं की बीजगणितीय संक्रियायें तथा समानता)30videos
सम्मिश्र संख्यायें
- BOOK - ERRORLESS HINDICHAPTER - सम्मिश्र संख्यायेंEXERCISE - Solved Multiple Choice Questions (सम्मिश्र संख्याओं का संयुग्मी, मापांक तथा कोणांक)55videos
सम्मिश्र संख्यायें
- BOOK - ERRORLESS HINDICHAPTER - समुच्चय सिद्धान्त तथा सम्बन्ध EXERCISE - JEE Advanced (Reasoning type questions)3videos
समुच्चय सिद्धान्त तथा सम्बन्ध
- BOOK - ERRORLESS HINDICHAPTER - सरल रेखा EXERCISE - JEE ADVANCE (Matrix Match type questions)4videos
सरल रेखा
Similar Questions
यदि (1+i)3(1−i)3−(1−i)3(1+i)3=x+iy
If (1+i)3(1−i)3−(1−i)3(1+i)3=x+iy01:59View Solutionसम्मिश्र संख्याएँ sinx+icos2x तथा cosx−isin2x
x के किस मान के लिये एक दूसरे की संयुग्मी हैं04:16View Solutionमाना R वास्तविक संख्याओं का समुच्चय है
कथन-1 : A={(x,y)∈R×R:y−x एक पूर्णांक है} R पर एक तुल्यता संबंध है |
कथन-2 : B={(x,y)∈R×R:x=αy किसी परिमेय संख्या α के लिए} R पर एक तुल्यता संबंध है |07:39View Solution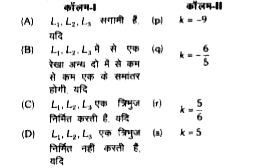
03:23View SolutionWhat is the value of (1=−+i√32)3n+(−1+i√32)3nwherei=√−1?
03:36View SolutionFind the argument of each of the following complex numbers : √3+i−1−i√3
03:34View SolutionThe sum of principal arguments of complex numbers 1+i,−1+i√3,−√3−i,√3−i,i,−3i,2,−1is
03:08View Solution4+5(−12+i√32)334+3(−12+i√32)365
04:19View Solutionদেখাও যে , (−1+i√32)19+(−1−i√32)19=−1 |
08:57View Solutionমান নির্ণয় করো: 4+5[−12+i√32]334+3[−12+i√32]365[i=√−1]
02:57View Solution|(1+isqrt3)/((1+1/(i+1))^2)| The value of
03:20View Solutionयदि z=1+i√3√3+i , तो (¯z)100 स्थित होगा
04:01View Solution4+5(−12+i√32)334+3(−12+i√32)365=
01:39View SolutionThe ascending order of the values of
z1=(1+i)4,z2=(√3+i)12,z3=(1+i√3)9+(1−i√3)903:05View SolutionThe ascending order of the values of
z1(1+i)4+(1−i)4,<br>z2=(√3+i)12+(√3−i)12,<br>z3=(1+i√3)9+(1−i√3)904:06View SolutionIf i=√−1, then 4+5(−12+i√32)334+3(−12+i√32)365 is equal to (1) 1−i√3 (2) −1+i√3 (3) i√3 (4) −i√3
01:03View Solution