यदि (1+i)(1+2i)(1+3i)….(1+ni)=a+ib, तब 2.5.10….. (1+n2) =
a2−b2
a2+b2
√a2+b2
√a2−b2
The correct Answer is:B
Step by step video, text & image solution for यदि (1+i)(1+2i)(1+3i)….(1+ni)=a+ib, तब 2.5.10….. (1+n^2) = by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- सम्मिश्र संख्यायेंBook:ERRORLESS HINDIChapter:सम्मिश्र संख्यायेंExercise:Jee Advanced (More than one correct Answers)
Explore 23 Videos
- सम्मिश्र संख्यायेंBook:ERRORLESS HINDIChapter:सम्मिश्र संख्यायेंExercise:Jee Advanced (Reasoning)
Explore 6 Videos
- सम्मिश्र संख्यायेंBook:ERRORLESS HINDIChapter:सम्मिश्र संख्यायेंExercise:Solved Multiple Choice Questions (डी मोयवर प्रमेय तथा इकाई के मूल )
Explore 59 Videos
- समुच्चय सिद्धान्त तथा सम्बन्ध Book:ERRORLESS HINDIChapter:समुच्चय सिद्धान्त तथा सम्बन्ध Exercise:JEE Advanced (Reasoning type questions)
Explore 3 Videos
- सरल रेखा Book:ERRORLESS HINDIChapter:सरल रेखा Exercise:JEE ADVANCE (Matrix Match type questions)
Explore 4 Videos
Similar Questions
माना z1 तथा z2 दो सम्मिश्र संख्याएँ इस प्रकार है कि z1≠z2 तथा |z1|=|z2| . यदि z1 धनात्मक वास्तविक भाग तथा z2 ऋणात्मक काल्पनिक भाग है, तब z1+z2z1−z2 होगा
View Solutionकथन-1: यदि n एक विषम पूर्णांक 3 से बडा है लेकिन 3 का गुणज नहीं है, तब (x+1)n−xn−1,x3+x2+x से विभाज्य है।
कथन-2: यदि n एक विषम पूर्णांक 3 से बड़ा है लेकिन 3 का गुणज नहीं है हम लेते हैं 1+ωn+ω2n=3
View Solution(cosθ+isinθ)4(sinθ+icosθ)5=
View Solutionमाना R वास्तविक संख्याओं का समुच्चय है
कथन-1 : A={(x,y)∈R×R:y−x एक पूर्णांक है} R पर एक तुल्यता संबंध है |
कथन-2 : B={(x,y)∈R×R:x=αy किसी परिमेय संख्या α के लिए} R पर एक तुल्यता संबंध है |
View Solution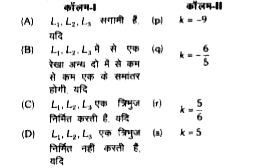
View Solution(1+i)(1+2i)(1+3i)............(1+ni)=alpha+i beta, then the value of 2.5.10......(1+n^(2))=alpha^(2)+beta^(2)
View SolutionIf (1+i)(1+2i)(1+3i)...(1+ni)=a+ib then 2xx5xx10xx...(1+n^(2))=
View SolutionIf (1+i)(1+2i)(1+3i)(1+ni)=(x+iy) , Show that 2.5.10.....(1+n2)=x2+y2.
View Solutionनिम्न को a+ib के रूप में व्यक्त कीजिये |
1+2i+3i21−2i+3i2
View SolutionIf (1+i)(1+2i)(1+3i),…(1+ni)=a+ib then the value of 2×5×10×…×(1+n2) is-
View SolutionIf (1 + i)(1 + 2i)(1 + 3i)….(1 + ni) = x + iy, then 2.5.10…(1+n2)is
View SolutionIf (1−i)(1−2i)(1−3i)…..(1−ni)=x−iy , then 2.5.10……(1+n2)=
View SolutionIf (1−i)(1−2i)(1−3i)…..(1−ni)=x−iy , then 2.5.10……(1+n2)=
View SolutionIf (1+i)(1+2i)(1+3i).......(1+ni)=x+iy, then 2.5.10.........(1+n2)=
View Solutiona+ib=(1+i)(1+2i)(1+3i) then a2+b2=
View Solution