सम्मिश्र संख्यायें z समीकरण |z- (4/z )| = 2 को संतुष्ट करती है।
यदि z का बिन्दुपथ |z−z1|=|z−z2| है, जहाँ z1 और z2 क्रमशः महत्तम और लघुत्तम मापांक की सम्मिश्र संख्यायें हैं
रेखा वास्तविक अक्ष के समान्तर है
रेखा काल्पनिक अक्ष के समान्तर है
रेखा धनात्मक प्रवणता की है
रेखा ऋणात्मक प्रवणता (ढाल) की है
The correct Answer is:B
Step by step video, text & image solution for सम्मिश्र संख्यायें z समीकरण |z- (4/z )| = 2 को संतुष्ट करती है। यदि z का बिन्दुपथ |z-z_1|=|z-z_2| है, जहाँ z_1 और z_2 क्रमशः महत्तम और लघुत्तम मापांक की सम्मिश्र संख्यायें हैं by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- सम्मिश्र संख्यायेंBook:ERRORLESS HINDIChapter:सम्मिश्र संख्यायेंExercise:Jee Advanced (Integer)
Explore 9 Videos
- सम्मिश्र संख्यायेंBook:ERRORLESS HINDIChapter:सम्मिश्र संख्यायेंExercise:Jee Advanced (Reasoning)
Explore 6 Videos
- समुच्चय सिद्धान्त तथा सम्बन्ध Book:ERRORLESS HINDIChapter:समुच्चय सिद्धान्त तथा सम्बन्ध Exercise:JEE Advanced (Reasoning type questions)
Explore 3 Videos
- सरल रेखा Book:ERRORLESS HINDIChapter:सरल रेखा Exercise:JEE ADVANCE (Matrix Match type questions)
Explore 4 Videos
Similar Questions
मान लीजिए कि z कोई सम्मिश्र संख्या है जिसके लिये |z−3−2i|≤2 सत्य है, तो |2z-6+5i| का न्यूनतम मान है
View Solutionकथन-1: यदि n एक विषम पूर्णांक 3 से बडा है लेकिन 3 का गुणज नहीं है, तब (x+1)n−xn−1,x3+x2+x से विभाज्य है।
कथन-2: यदि n एक विषम पूर्णांक 3 से बड़ा है लेकिन 3 का गुणज नहीं है हम लेते हैं 1+ωn+ω2n=3
View Solutionमाना R वास्तविक संख्याओं का समुच्चय है
कथन-1 : A={(x,y)∈R×R:y−x एक पूर्णांक है} R पर एक तुल्यता संबंध है |
कथन-2 : B={(x,y)∈R×R:x=αy किसी परिमेय संख्या α के लिए} R पर एक तुल्यता संबंध है |
View Solution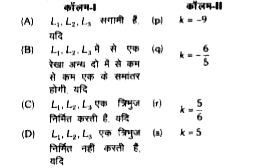
View SolutionComplex number z1 and z2 satisfy z+¯z=2|z−1| and arg (z1−z2)=π4 . Then the value of lm(z1+z2) is
View Solutionसम्मिश्र संख्या z का बिन्दुपथ ज्ञात कीजिए यदि |z+4i|+|z−4i|=10
View SolutionIf ∣∣∣z−z1z−z2∣∣∣=1, where z1andz2 are fixed complex numbers and z is a variable complex number, then z lies on a
View SolutionIf ∣∣∣z1z−z2z1z+z2∣∣∣=k,(z1,z2≠0) then
View Solutionयदि |(z−z1)/(z−z2)|=3 , जहाँ z1 और z2 स्थिर सम्मिश्र संख्यायें तथा z एक चर सम्मिश्र संख्या है. तब 'z' स्थित होगा
View SolutionThe maximum area of the triangle formed by the complex coordinates z,z1,z2 which satisfy the relations |z−z1|=|z−z2| and ∣∣∣z−z1+z22∣∣∣≤r,where r>|z1−z2| is
View SolutionLet z1andz2 be two distinct complex numbers and let z=(1−t)z1+tz2 for some real number t with 0<t<1. If a r g(w) denotes the principal argument of a nonzero complex number w , then
|z−z1|+|z−z2|=|z1−z2| (z−z1)=(z−z2)
∣∣z−z1z−(z)1z2−z1(z)2−(z)1∣∣=0
arg(z−z1)=arg(z2−z1)
View SolutionIf z1,z2,z3,z4 are the affixes of four point in the Argand plane, z is the affix of a point such that |z−z1|=|z−z2|=|z−z3|=|z−z4| , then z1,z2,z3,z4 are
View SolutionIf z1,z2,z3,z4 are the affixes of four point in the Argand plane, z is the affix of a point such that |z−z1|=|z−z2|=|z−z3|=|z−z4| , then prove that z1,z2,z3,z4 are concyclic.
View SolutionLet z1,z2,z3∈C such that |z1|=|z2|=|z3|=|z1+z2+z3|=4.
If |z1−z2|=|z1+z3|andz2≠z3, then values of |z1+z2|⋅|z1+z3| is _____.
View SolutionIf arg z−z1z2−z1 = 0 for three distinct complex numbers z,z1,z2 then the three points are
View SolutionIf z1≠−z2 and |z1+z2|=∣∣∣1z1+1z2∣∣∣ then :
View Solution
