यदि $Δ (x) = ∣ ∣ ∣ ∣ ∣ \begin{matrix} x^{n} & sin x & cos x n! & sin \frac{n π}{2} & cos \frac{n π}{2} a & a^{2} & a^{3} \end{matrix} ∣ ∣ ∣ ∣ ∣$ तो $x = 0$ पर $\frac{d^{n}}{{d x}^{n}} [Δ (x)]$ का मान है

$- 1$

$0$

$1$

a पर निर्भर

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Answer

Step by step video & text solution for यदि Delta(x)=|{:(x^(n),sinx,cosx),(n!,sin""(npi)/(2),cos""(npi)/(2)),(a,a^(2),a^(3)):}| तो x=0 पर (d^(n))/(dx^(n))[Delta(x)] का मान है by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS DETERMINANTS

Topper's Solved these Questions

BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - Solved Multiple Choice Questions (सारणिक का प्रसार , सारणिक के रूप में समीकरण का हल एवं सारणिक के गुणधर्म)
150videos
सारणिक तथा आव्यूह
BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - Solved Multiple Choice Questions ( रैखिक समीकरणों का निकाय कुछ विशेष सारणिक, सारणिक का अवकलन तथा समाकलन)
50videos
सारणिक तथा आव्यूह
BOOK - ERRORLESS HINDICHAPTER - सरल रेखा EXERCISE - JEE ADVANCE (Matrix Match type questions)
4videos
सरल रेखा

Similar Questions

$∣ ∣ ∣ ∣ ∣ \begin{matrix} 1 & a & a^{2} - b c 1 & b & b^{2} - a c 1 & c & c^{2} - a b \end{matrix} ∣ ∣ ∣ ∣ ∣ =$
10:02
View Solution
a का वह मान जिसके लिये समीकरण निकाय $a^{3} x + {(a + 1)}^{3} y + {(a + 2)}^{3} z = 0, a x (a + 1) y + (a + 2) z = 0, x + y + z = 0$ का एक अशून्य हल है
07:38
View Solution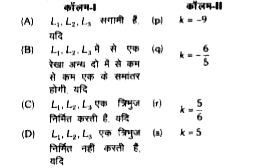
08:05
View Solution
If A(x)=det[[x^(n),sin x,cos xn!sin((n pi)/(2)),cos((n pi)/(2))a,a^(2),a^(3)]], then the value of (d^(n))/(dx^(n))[Delta(x)] at x=0 is
02:59
View Solution
If $f (x) = ∣ ∣ ∣ ∣ ∣ \begin{matrix} x^{n} & sin x & cos x n! & sin (\frac{n π}{2}) & cos (\frac{n π}{2}) a & a^{2} & a^{3} \end{matrix} ∣ ∣ ∣ ∣ ∣$ , then show that $\frac{d^{n}}{{d x}^{n}} [f (x)]$ at x=0 is 0
05:59
View Solution
If $f (x) = ∣ ∣ ∣ ∣ ∣ \begin{matrix} x^{n} & sin x & cos x n! & sin \frac{n π}{2} & cos \frac{n π}{2} a & a^{2} & a^{3} \end{matrix} ∣ ∣ ∣ ∣ ∣$ , then the value of $\frac{d^{n}}{{d x}^{n}} (f (x)) at x = 0 for n = 2 m + 1$ is
View Solution
$if = ∣ ∣ ∣ ∣ ∣ \begin{matrix} x^{n} & n! & 2 cos x & cos \frac{n π}{2} & 4 sin x & sin \frac{n π}{2} & 8 \end{matrix} ∣ ∣ ∣ ∣ ∣,$ then find the value of
$\frac{d^{n}}{{d x}^{n}} {[f (x)]}_{x = 0} (n \in z)$
04:19
View Solution
Let $f(x) ∣ ∣ ∣ ∣ ∣ ∣ \begin{matrix} π^{n} & sin π x & cos π x {(- 1)}^{n}! & - sin (\frac{n π}{2}) & - cos (\frac{n π}{2}) - 1 & \frac{1}{\sqrt{2}} & \frac{\sqrt{3}}{2} \end{matrix} ∣ ∣ ∣ ∣ ∣ ∣$
Then value or $\frac{d^{n}}{{d x}^{n}} [f(x)] at x = 1 is$
03:52
View Solution
If $f (x) = ∣ ∣ ∣ ∣ ∣ \begin{matrix} x^{n} & sin x & cos x n! & sin (n π / 2) & cos (n π / 2) a & a^{2} & a^{2} \end{matrix} ∣ ∣ ∣ ∣ ∣$ , then $\frac{d^{n}}{{d x}^{n}} {f (x)}$ at $x = 0$ is
04:20
View Solution
The solutions of the equation $1 + (sin x - cos x) \frac{sin π}{4} = 2 \frac{{cos}^{2} (5 x)}{2}$ is/are $x = \frac{n π}{3} + \frac{π}{8}, n \in Z$ $x = \frac{n π}{2} + \frac{5 π}{16}, n \in Z$ $x = \frac{n π}{3} + \frac{π}{4}, n \in Z$ $x = \frac{n π}{2} + \frac{7 π}{8}, n \in Z$
04:15
View Solution
$if = ∣ ∣ ∣ ∣ ∣ \begin{matrix} x^{n} & n! & 2 cos x & cos . \frac{n π}{2} & 4 sin x & sin . \frac{n π}{2} & 8 \end{matrix} ∣ ∣ ∣ ∣ ∣,$ then find the value of
$\frac{d^{n}}{{d x}^{n}} {[f (x)]}_{x = 0} . (n \in z) .$
07:11
View Solution
If $f (x) = ∣ ∣ \begin{matrix} x^{n} & n! & 2; cos x & cos (\frac{n π}{2}) & 4; sin x & sin (\frac{n π}{2}) & 8 \end{matrix} ∣ ∣$ then find the value of $\frac{d^{n}}{{d x}^{n}} {([f (x)])}_{x = 0} . n \in z .$
10:14
View Solution
Let $f(x) = ∣ ∣ ∣ ∣ ∣ ∣ \begin{matrix} π^{n} & sin π x & cos π x {(- 1)}^{n}! & - sin (\frac{n π}{2}) & - cos (\frac{n π}{2}) - 1 & \frac{1}{\sqrt{2}} & \frac{\sqrt{3}}{2} \end{matrix} ∣ ∣ ∣ ∣ ∣ ∣$
Then value or $\frac{d^{n}}{{d x}^{n}} [f(x)] at x = 1 is$
05:49
View Solution
If $f (x) = ∣ ∣ ∣ ∣ ∣ \begin{matrix} x^{n} & sin x & cos x n! & sin \frac{n π}{2} & cos \frac{n π}{2} a & a^{2} & a^{3} \end{matrix} ∣ ∣ ∣ ∣ ∣$ , then the value of $\frac{d^{n}}{{d x}^{n}} (f (x)) at x = 0 for n = 2 m + 1$ is
03:51
View Solution
If $f (x) = ∣ ∣ ∣ ∣ ∣ \begin{matrix} x^{n} & sin x & cos x n! & sin (\frac{n π}{2}) & cos (\frac{n π}{2}) a & a^{2} & a^{3} \end{matrix} ∣ ∣ ∣ ∣ ∣$ , then show that $\frac{d^{n}}{{d x}^{n}} [f (x)]$ at x=0 is 0
03:44
View Solution

ERRORLESS HINDI-सारणिक तथा आव्यूह -JEE ADVANCED MORE THAN ONE CORRECT ANSWERS (Matrix Match type questions)

यदि Delta(x)=|{:(x^(n),sinx,cosx),(n!,sin""(npi)/(2),cos""(npi)/(2)),(...
09:31
इस खण्ड में कुछ प्रश्न है। प्रत्येक प्रश्न में कॉलम -I में कुछ कथन (A,...
07:08
यदि |(1+x,x,x^(2)),(x,1+x,x^(2)),(x^(2),x,1+x)|=px^(5)+qx^(4)+rx^(3)+s...
08:24
इस खण्ड में कुछ प्रश्न है। प्रत्येक प्रश्न में कॉलम -I में कुछ कथन (A,...
07:08
यदि |(1+x,x,x^(2)),(x,1+x,x^(2)),(x^(2),x,1+x)|=px^(5)+qx^(4)+rx^(3)+s...
Text Solution

Home
Ask Doubt
Profile

यदि Δ(x)=∣∣ ∣ ∣∣xnsinxcosxn!sinnπ2cosnπ2aa2a3∣∣ ∣ ∣∣ तो x=0 पर dndxn[Δ(x)] का मान है

The correct Answer is:B

Topper's Solved these Questions

Similar Questions

ERRORLESS HINDI-सारणिक तथा आव्यूह -JEE ADVANCED MORE THAN ONE CORRECT ANSWERS (Matrix Match type questions)

यदि $Δ (x) = ∣ ∣ ∣ ∣ ∣ \begin{matrix} x^{n} & sin x & cos x n! & sin \frac{n π}{2} & cos \frac{n π}{2} a & a^{2} & a^{3} \end{matrix} ∣ ∣ ∣ ∣ ∣$ तो $x = 0$ पर $\frac{d^{n}}{{d x}^{n}} [Δ (x)]$ का मान है