यदि Un=∣∣ ∣ ∣∣n15n22N+12N+1n33N23N∣∣ ∣ ∣∣ तब N∑n=1Un का मान है
0
1
−1
इनमे से कोई नहीं
The correct Answer is:A
Step by step video, text & image solution for यदि U_(n)=|(n,1,5),(n^(2),2N+1,2N+1),(n^(3),3N^(2),3N)| तब sum_(n=1)^(N)U_(n) का मान है by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- सारणिक तथा आव्यूह BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - Solved Multiple Choice Questions ( आव्यूह के प्रकार, आव्यूह का बीजगणित)
130 Videos
- सारणिक तथा आव्यूह BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - EXERCISE
262 Videos
- सारणिक तथा आव्यूह BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - Solved Multiple Choice Questions (सारणिक का प्रसार , सारणिक के रूप में समीकरण का हल एवं सारणिक के गुणधर्म)
150 Videos
- सरल रेखा BOOK - ERRORLESS HINDICHAPTER - सरल रेखा EXERCISE - JEE ADVANCE (Matrix Match type questions)
4 Videos
Similar Questions
यदि M=[1223] और M2−λM−I2=0 तब λ=
View Solution∣∣ ∣ ∣∣111cos(nx)cos(n+1)xcos(n+2)xsin(nx)sin(n+1)xsin(n+2)x∣∣ ∣ ∣∣ निर्भर नहीं करता है
View Solution∣∣ ∣ ∣∣1aa2−bc1bb2−ac1cc2−ab∣∣ ∣ ∣∣=
View Solution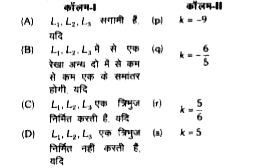
View SolutionIf U_n=|(n,1,5),(n^2, 2N+1,2N+1),(n^3,3N^2,3N+1)|, then sum_(n=1)^NU_n is equal to (A) 2sum_(n=1)^Nn (B) 2sum_(n=1)^N(n^2) (C) (1/2)sum_(n=1)^N(n^2) (D) 0
View SolutionIf Un=(√3+1)2n+(√3−1)2n , then prove that Un+1=8Un−4Un−1.
View SolutionFor Un=∫10xn(2−x)ndx;Vn=∫10xn(1−x)ndxn∈N, which of the following statement(s) is/are true? (a)Un=2nVn (b) Un=2−nVn (c)Un=22nVn (d) Vn=2−2nUn
View SolutionLet U_(1)=1,U_(2)=1 and U_(n+2)=U_(n+1)+U_(n) for n>=1. Use mathematical induction to such that: :U_(n)=(1)/(sqrt(5)){((1+sqrt(5))/(2))^(n)-((1-sqrt(5))/(2))^(n)} for all n>1
View SolutionConsider the sequence u_(n)=sum_(r=1)^(n)(r)/(2^(r)),n>=1 then the lim it_(n rarr oo)u_(n)
View Solutionlimn→∞((n+1)(n+2)(n+3).......3nn2n)1nis equal to
View SolutionIf ∑n=nn=1(n2+3n+3)(n+1)!(n+2)!=8, then n is equal to :
View SolutionIf Sn=u1+u2+u3+…+un=4n−1, find the first four terms of the sequence {un}.
View SolutionIf Sn=u1+u2+….+un=n2+2n, find the first four terms of the series.
View SolutionFor the sequence {un} if u1=14 and un+1=un2+un, find the value of 1u50.
View SolutionIf nεN and u1=xn,u2=xn−1,u3=xn−2,...,un=x, then u1'.u2'.u3...un'=
View Solutionमाना u1=1,u2=1 और un+1=un+1+un'n≥1गणितीय आगमन के प्रयोग से दिखाइए कि un=1√5[(1+√52)n−(1−√52)n],∀n>1
View Solution
