निम्नलिखित में से a और L के कौन सा (से) मान समीकरण
∫4π0et(sin6at+cos4at)dt∫t0et(sin6at+cos4at)dt=L
को संतुष्ट करता (करते) हैं?
a=2,L=e4π−1eπ−1
a=2,L=e4π+1eπ+1
a=4,L=e4π−1eπ−1
a=4,L=e4π+1eπ+1
Step by step video solution for निम्नलिखित में से a और L के कौन सा (से) मान समीकरण (int_(0)^(4pi)e^t(sin^6at +cos^4at)dt)/(int_0^te^t(sin^6 at + cos^4 at)dt)=L को संतुष्ट करता (करते) हैं? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- JEE ADVANCED पेपर - 2 (2015)BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - JEE ADVANCED पेपर - 2 (2015)EXERCISE - भाग III : गणित (खंड - 3)
4 Videos
- JEE ADVANCED पेपर - 2 (2015)BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - JEE ADVANCED पेपर - 2 (2015)EXERCISE - भाग III : गणित (खंड - 3)
4 Videos
- JEE ADVANCED पेपर - 2 (2014)BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - JEE ADVANCED पेपर - 2 (2014)EXERCISE - PART III : MATHEMATICS (खण्ड - 3 : सुमेलन सूची प्रकार (केवल एक विकल्प सही) )
4 Videos
- JEE ADVANCED पेपर - 2 (2016)BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - JEE ADVANCED पेपर - 2 (2016)EXERCISE - भाग- III गणित (खंड-3)
4 Videos
Similar Questions
माना कि बॉक्स I में n1 लाल गेंद और n2 काली गेंद हैं । माना कि बॉक्स II में n3 लाल गेंद और n4 काली गेंद हैं ।
बॉक्स I और बॉक्स II में से, यादृच्चया (at random) एक बॉक्स को चुना गया और इस चुने हुए बॉक्स से, यादृच्चया एक गेंद निकाली गयी । यह गेंद लाल रंग की पाई गयी । यदि इस लाल गेंद के बॉक्स II से निकाले जाने की प्रायिकता 13 है, तब निम्नलिखित में से n1,n2,n3 और n4 के सही संभव मान है (हैं)
View Solutionमाना कि बॉक्स I में n1 लाल गेंद और n2 काली गेंद हैं । माना कि बॉक्स II में n3 लाल गेंद और n4 काली गेंद हैं ।
बॉक्स I और बॉक्स II में से, यादृच्चया (at random) एक बॉक्स को चुना गया और इस चुने हुए बॉक्स से, यादृच्चया एक गेंद निकाली गयी । यह गेंद लाल रंग की पाई गयी । यदि इस लाल गेंद के बॉक्स II से निकाले जाने की प्रायिकता 13 है, तब निम्नलिखित में से n1,n2,n3 और n4 के सही संभव मान है (हैं)
View Solutionइस खंड में 4 बहुविकल्पीय प्रश्न है। प्रत्येक प्रश्न में दो सुमेलन सूचियां है। सूचियों के लिए कूट के विकल्प और है जिनमें से केवल एक सही है।
माना की zk=cos(2kπ10)+isin(2kπ10),k=1,2,…,9
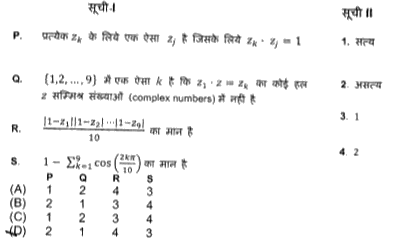
View Solutionअनुच्छेद
फुटबॉल दलों T1 और T2 को एक दूसरे के विरुद्ध दो खेल (games) खेलने हैं। यह मान लिया गया है कि दोनों खेलों के परिणाम
एक दूसरे पर निर्भर नहीं करते। दल T1 के दल T2 के विरुद्ध एक खेल में जीतने, बराबर होने और हारने की प्रायिकता क्रमशः 12,16
और 13 हैं। प्रत्येक दल जीतने पर 3 अंक, बराबरी पर 1 अंक और हारने पर 0 अंक अर्जित करता है। माना कि दो खेलों के पश्चात दल
T1 और दल T2 के द्वारा अर्जित कुल अंक क्रमशः X और Y हैं।
P(X>Y) का मान है
View SolutionThe option(s) with the values of a and L that satisfy the following equation is (are) ∫4π0et(sin6at+cos4at)dt∫π0et(sin6at+cos4at)dt=L
View SolutionThe value of int_(0)^(sin^(2)) sin^(-1)sqrt(t)dt+int_(0)^(cos^(2)x)cos^(-1)sqrt(t)dt , is
View SolutionThe options(s) with the values of a and L that satisfy the following equation is(are)
∫4π0et(sin6at+cos4at)dt∫π0et(sin6at+cos4at)dt=L?
View Solutionनिम्नलिखित में से a और L के कौन सा (से) मान समीकरण ∫4π0et(sin6at+cos4at)dt∫π0et(sin6at+cos4at)dt=L को संतुष्ट करता (करते) हैं
View Solution∫sin2x0sin−1√tdt+∫cos2x0cos−1√tdt=
View Solutionint_(0)^(sin^(2)x) sin^(-1) sqrt(t) dt + int_(0)^(cos^(2)x) cos^(-1) sqrt(t) dt=
View SolutionThe option(s) with the values of a and L that satisfy the following equation is (are) ∫4π0et(sin6at+cos4at)dt∫π0et(sin6at+cos4at)dt=L
View SolutionWhich of the following (a) value equations of a and L (int_0^(4pi)e^(t)(sin^(6)at+cos^(4)at)dt)/(int_0^(pi)e^(t)(sin^(6)at+cos^(4)at)dt)=L Satisfies (do)
View Solution∫π40 sin2tsin4t+cos4t dt is..........
View SolutionThe option(s) with the values of a and L that satisfy the following equation is (are)
∫4π0et(sin6at+cos4at)dt∫π0et(sin6at+cos4at)dt=L
View SolutionIf 1sint1+tdt=α, then that value of the integral :
∫4π4π−2sint24π+2−tdt in terms of α is given by :
View SolutionThe option(s) with the values of a and L that satisfy the following equation is (are) ∫4π0et(sin6at+cos4at)dt∫π0et(sin6at+cos4at)dt=L
View Solution
