फलन के परिप्रेक्ष्य में निम्नलिखित पर विचार कीजिए
पर सतत है । पर अवकलनीय है ।
उपरोक्त कथनों में से कौन-सा/से कथन सही है/हैं ?
केवल I
केवल II
I और II दोनों
न तो I और न ही II
The correct Answer is:C
दिया गया फलन
. बाईं पक्ष सीमा x=3 पर,
दाईं पक्ष सीमा x=3 पर, f(3+0)
यहाँ बाईं पक्ष सीमा = दाईं पक्ष सीमा
इस प्रकार फलन पर सतत है ।
अतः फलन पर अवकलनीय है ।
Topper's Solved these Questions
Similar Questions
यदि limx→0kxcosecx=limx→0xcoseckx है, तो k का मान है
View Solution∣∣ ∣ ∣∣−a2abacab−b2bcacbc−c2∣∣ ∣ ∣∣ किसके बराबर है ?
View Solutionसिद्ध कीजिए की फलन f(x)=5x-3,x=0 पर सतत है।
View Solutionसिद्ध कीजिए कि फलन f(x)=|x|,x=0 पर अवकलनीय नहीं है यद्यपि यह x=0 पर संतत है|
View Solutionनिम्नलिखित में से कौन सा कथन सही नहीं है?
View Solutionनिम्नलिखित में से कौन सा कथन सही नहीं है?
View SolutionX और Y अणुओं के संबंध में निम्नलिखित कथनों में से कौन सा गलत है?
View Solutionनिम्नलिखित में से कौन सा कथन सही नहीं है?
View Solutionऊष्मागतिकी के प्रथम नियम के आधार पर, निम्नलिखित में से कौन सा सही है?
View Solutionनिम्नलिखित कथनों में से कौन सा सही है?
View Solutionनिम्नलिखित में से कौन सा कथन सही है?
View Solutionनिम्नलिखित में से कौन सा कथन सही नहीं है?
View Solutionनिम्नलिखित में से कौन सा कथन सही है?
View Solutionनिम्नलिखित में से कौन सा कथन सही नहीं है?
View Solutionक्वांटम संख्या के निम्नलिखित समुच्चयों पर विचार कीजिए:
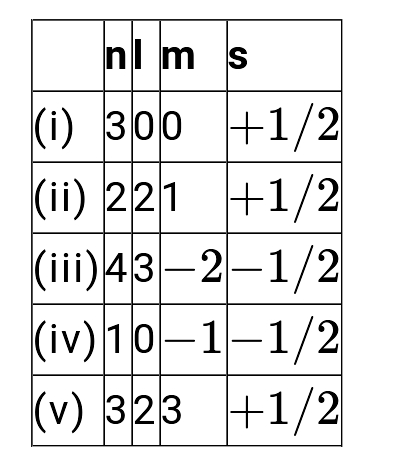
निम्नलिखित में से क्वांटम संख्या का कौन सा समुच्चय संभव नहीं है?
View Solutionनिम्नलिखित में से कौन -सा कथन सही नहीं है ?
View Solution
