All the florets are disc florets in
launea
vernonia
sunflower
tridax
The correct Answer is:b
Step by step video & image solution for All the florets are disc florets in by Biology experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- INFIORESCENCEBook:DINESH PUBLICATION ENGLISHChapter:INFIORESCENCEExercise:check your grasp
Explore 10 Videos
- HUMAN REPRODUCTIONBook:DINESH PUBLICATION ENGLISHChapter:HUMAN REPRODUCTIONExercise:MCQ
Explore 770 Videos
- INTRODUCTION Book:DINESH PUBLICATION ENGLISHChapter:INTRODUCTION Exercise:CYG
Explore 10 Videos
Similar Questions
Floret is
View SolutionRay florets have:
View SolutionHensen's disc occurs in
View SolutionWork energy theorem sys work done by all the force is equal to change in kinetic energy" In the diagram, a disc of mass M and radius Ris rolling without slipping on a rough fixed inclined AB=l,V= velocity of disc at B.
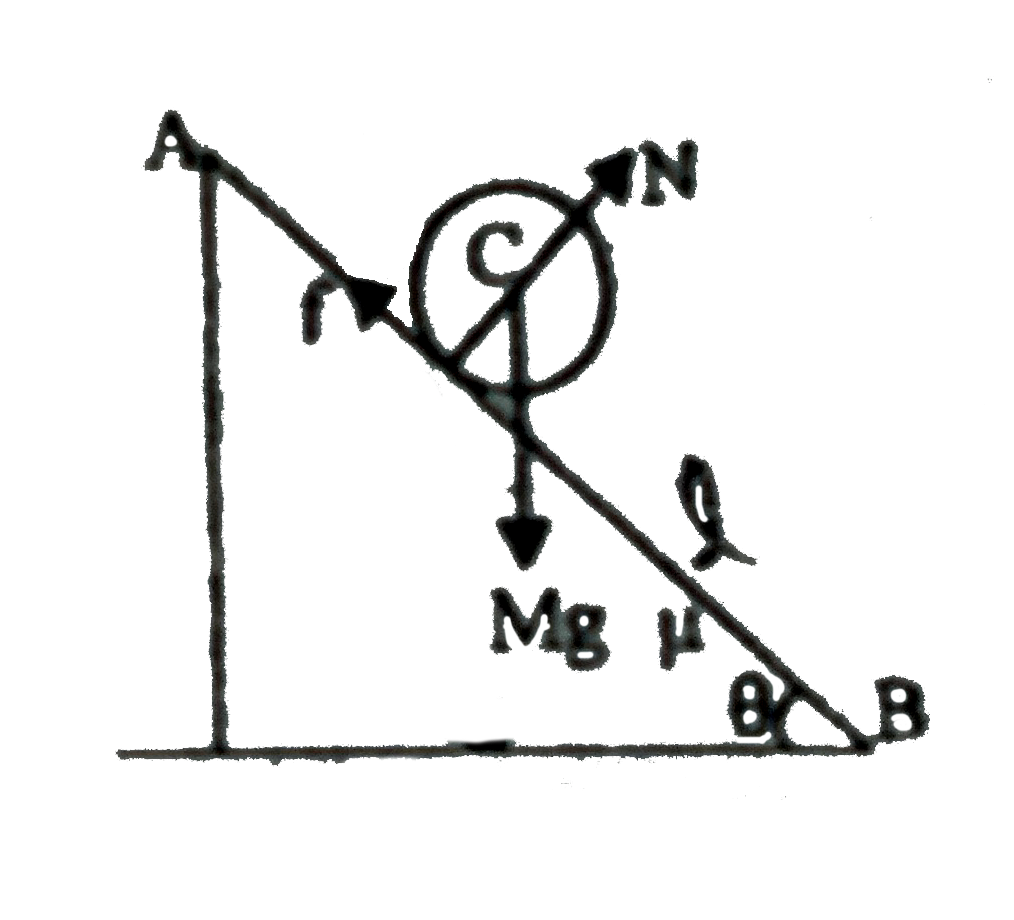
Choose the correct option(s)
View SolutionWork energy theorem sys"work done by all the force is equal to change in kinetic energy" In the diagram, a disc of mass M and radius Ris rolling without slipping on a rough fixed inclined AB=l,V= velocity of disc at B.
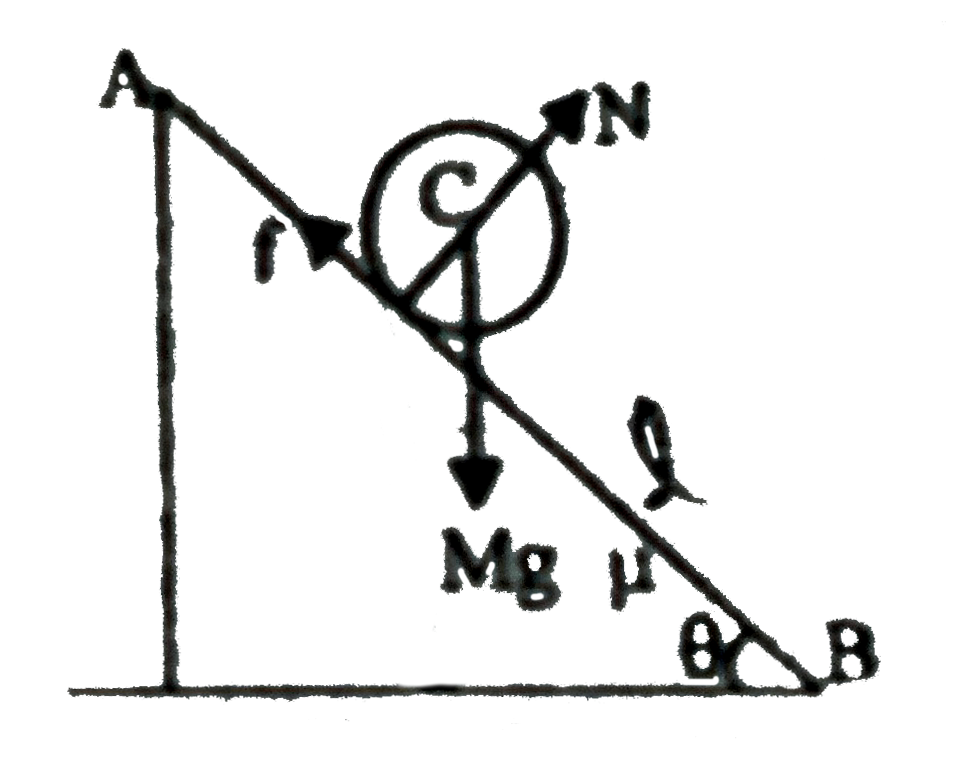
Choose the correct option(s)
View SolutionInter-vertebral disc occurs in :
View SolutionAssertion : In head inflorescence , florets are arranged centrifugally .
Reason : There are always two types of florets in head.
View SolutionOn a disc of radius R a concentric circle of radius R/2 is drawn. The disc is free to rotate about a frictionless fixed axis through its center and perpendicular to plane of the disc. All three forces ( in plane of the disc ) shown in figure are exerted tangent to their respective circular periphery. The magnitude of the net torque ( about centre of disc ) acting on the disc is :
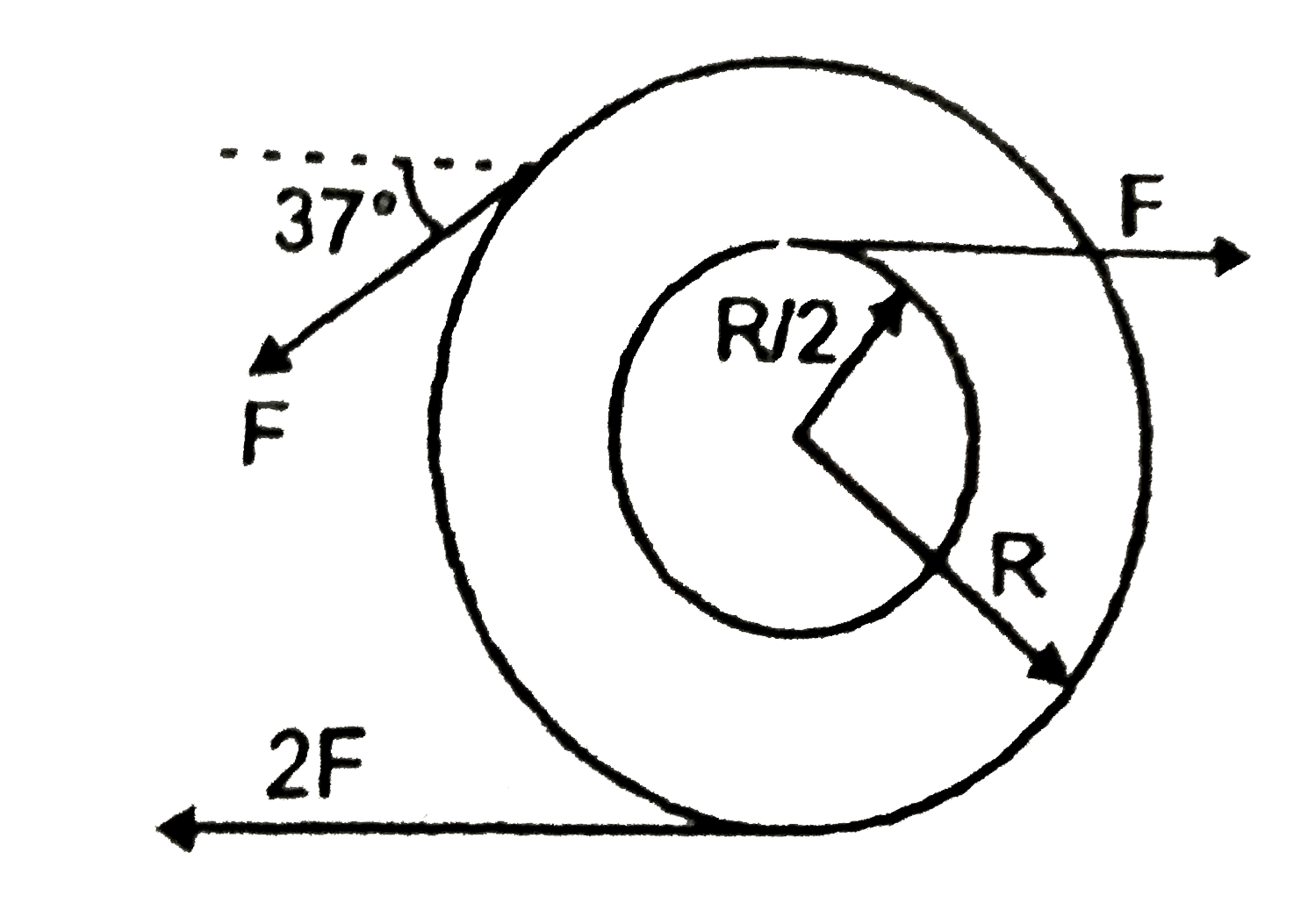
View SolutionOn a disc of radius R a concentric circle of radius R/2 is drawn. The disc is free to rotate about a frictionless fixed axis through its center and perpendicular to plane of the disc. All three forces ( in plane of the disc ) shown in figure are exerted tangent to their respective circular periphery. The magnitude of the net torque ( about centre of disc ) acting on the disc is :

View SolutionConsider three bodies : a ring, a disc and a sphere. All the bodies have same mass and radius. All rotate about their axis through their respective centre of mass and perpendicular to the plane. What is the ratio of moment of inertia ?
View Solution
