Question

Explain the principle of carrying capacity by using population Verhulst-Pearl logistic growth curve.

Explanation

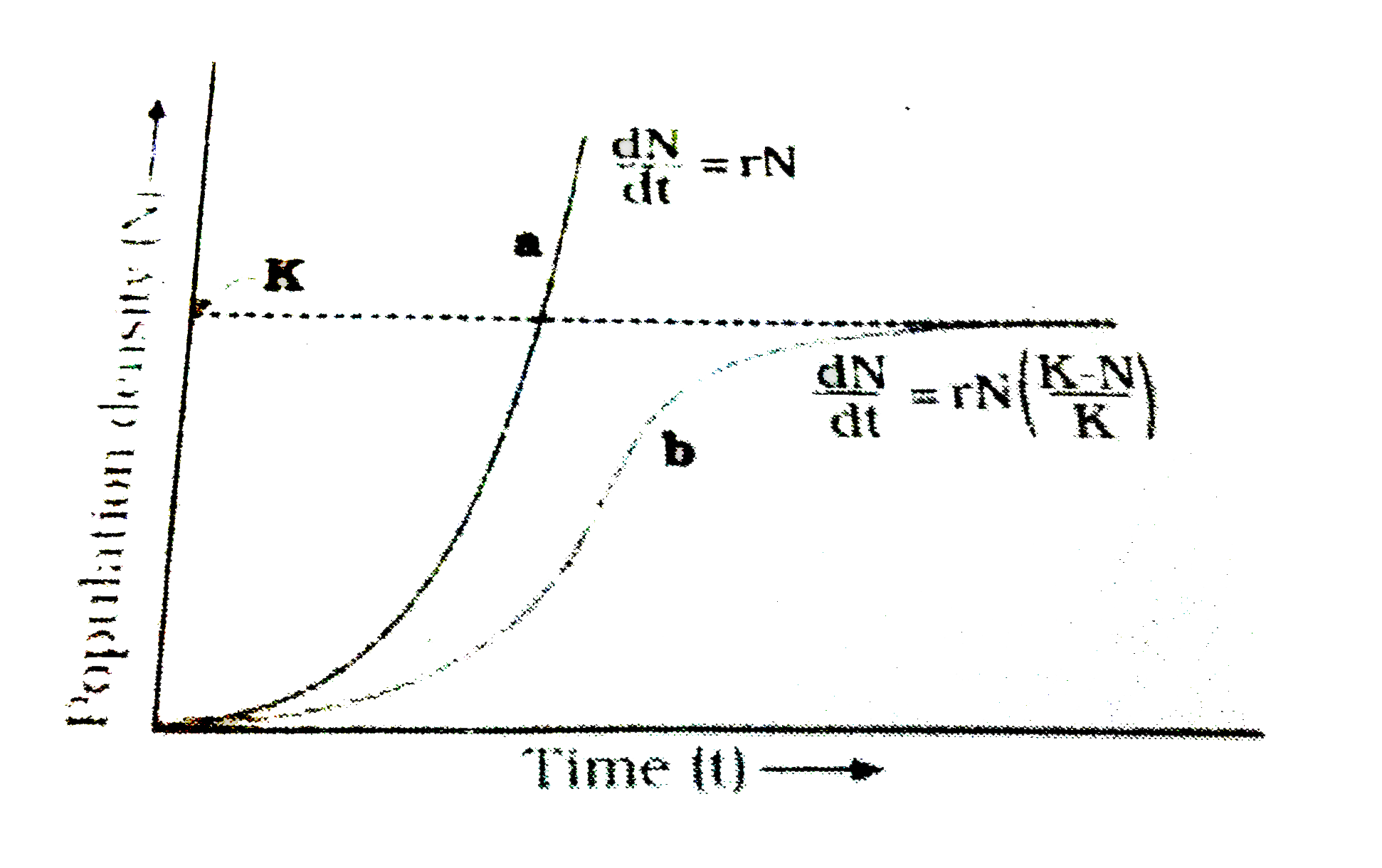
Given habitat has enough resource to support a maximum possible number beyond which no-further growth is possible. This is carrying capacity. A population growing in region with limited resources shows initially a log phase, followed by acceleration and decleration and at last an asymptote when the population reaches the carrying capacity. A plot of populatoin density (N) in relation to time (t) results in a sigmoid curve and is called the Verhulst-Pearl logistic growth curve, given by
`"the equation " (dN)/(dt) = rN [(k-N)/(K)]`
Where N= population density
r = intrinsic rate of population increase
k = carrying capacity.