A certain metal when irradiated to light (v=3.2×1016Hz) emits photoelectrons with twice kinetic energy as did photoelectrons when the same metal is irradiation by light (v=2.0×1016Hz). The v0 Threshold frequency ) of the metal is
1.2×1014Hz
8×1015 Hz
1.2×1016 Hz
4×1012 Hz
The correct Answer is:B
To solve the problem, we need to find the threshold frequency v0 of the metal based on the given information about the kinetic energies of the emitted photoelectrons when the metal is irradiated with two different frequencies of light.
1. Understanding the Photoelectric Effect:
The kinetic energy (KE) of emitted photoelectrons can be expressed using the equation:
KE=hν−hv0
where h is Planck's constant, ν is the frequency of the incident light, and v0 is the threshold frequency.
2. Setting Up the Equations:
For the first frequency ν1=3.2×1016Hz:
KE1=hν1−hv0
For the second frequency ν2=2.0×1016Hz:
KE2=hν2−hv0
3. Relating the Kinetic Energies:
According to the problem, the kinetic energy of the photoelectrons emitted at frequency ν1 is twice that of those emitted at frequency ν2:
KE1=2×KE2
4. Substituting the Kinetic Energy Equations:
Substitute the expressions for KE1 and KE2:
hν1−hv0=2(hν2−hv0)
5. Simplifying the Equation:
Expanding the equation gives:
hν1−hv0=2hν2−2hv0
Rearranging terms leads to:
hν1+hv0=2hν2
6. Isolating the Threshold Frequency:
Dividing through by h (assuming h≠0):
ν1+v0=2ν2
Rearranging gives:
v0=2ν2−ν1
7. Substituting the Values:
Now substitute ν1=3.2×1016Hz and ν2=2.0×1016Hz:
v0=2(2.0×1016)−3.2×1016
v0=4.0×1016−3.2×1016
v0=0.8×1016Hz=8.0×1015Hz
8. Final Result:
Thus, the threshold frequency v0 of the metal is:
8.0×1015Hz
The kinetic energy (KE) of emitted photoelectrons can be expressed using the equation:
KE=hν−hv0
where h is Planck's constant, ν is the frequency of the incident light, and v0 is the threshold frequency.
For the first frequency ν1=3.2×1016Hz:
KE1=hν1−hv0
For the second frequency ν2=2.0×1016Hz:
KE2=hν2−hv0
According to the problem, the kinetic energy of the photoelectrons emitted at frequency ν1 is twice that of those emitted at frequency ν2:
KE1=2×KE2
Substitute the expressions for KE1 and KE2:
hν1−hv0=2(hν2−hv0)
Expanding the equation gives:
hν1−hv0=2hν2−2hv0
Rearranging terms leads to:
hν1+hv0=2hν2
Dividing through by h (assuming h≠0):
ν1+v0=2ν2
Rearranging gives:
v0=2ν2−ν1
Now substitute ν1=3.2×1016Hz and ν2=2.0×1016Hz:
v0=2(2.0×1016)−3.2×1016
v0=4.0×1016−3.2×1016
v0=0.8×1016Hz=8.0×1015Hz
Thus, the threshold frequency v0 of the metal is:
8.0×1015Hz
Topper's Solved these Questions
- STRUCTURE OF ATOM BOOK - NCERT FINGERTPS ENGLISHCHAPTER - STRUCTURE OF ATOM EXERCISE - HIGHER ORDER THINKING SKILLS
11 Videos
- STRUCTURE OF ATOM BOOK - NCERT FINGERTPS ENGLISHCHAPTER - STRUCTURE OF ATOM EXERCISE - NCERT EXEMPLAR PROBLEMS
16 Videos
- STATES OF MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - STATES OF MATTER EXERCISE - Assertion And Reason
15 Videos
- THE P-BLOCK ELEMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THE P-BLOCK ELEMENTSEXERCISE - Assertion And Reason
15 Videos
Similar Questions
When a certain metal was irradiated with a light of 8.1×1016 Hz frequency the photoelectron emitted had 1.5 times the kinetic energy as did the photoelectrons emitted when the same metal was irradiated with light 5.8×1016 Hz frequency if the same metal is irradiated with light of 3.846 nm wave length what will be the energy of the photoelectron emitted ?
View SolutionWhat a certain was metal was irradiatiobn with light of frequency 1.6×1016Hz the photoelectron emitted but the kinetic energy as the photoelectron emitted when the same metal was irradiation with light of frequency 1.0×1016Hz .Calculate the threslold frequency (v0) for the metal
View SolutionWhen a certain metal was irradiated with light of frequency 3.2×1016s−1 the photoelectrons emitted had three twice the KE as did photoelectrons emitted when the same metal was irradited with light of frequency2.0×1016s−1 .Calculate the thereshold frequency of the metal
View SolutionWhen a certain metal was irradiated with light of frequency 4.0×1019s−1 the photoelectrons emitted had three times the kinetic energy as the kinetic energy of photoelectrons emitted when the metal was irradited with light of frequency 2.0×1016s−1 .Calculate the critical frequency (v0) of the metal
View SolutionThe maximum kinetic energy of the photoelectrons is found to be 6.63×10−19J, when the metal is irradiated with a radiation of frequency 2×1015Hz. The threshold frequency of the metal is about:
View SolutionIf a light with frequency 4×1016Hz emitted photoelectrons with double the maximum kinetic as are emitted by the light of frequency 2.5×1016Hz from the same metal surface, then what is the threshold frequency (v0) of the metal?
View SolutionIf a metal is irradiated with light of frequency 3×1019sec−1, electron is emitted with kinetic energy of 6.625×10−15J. The threshold frequency of the metal is
View SolutionIn an experiment of photo-electric effect, the graph of maximum kinetic energy Ek of the emitted photoelectrons versus the frequency v of the incident light is a straight line AB as shown in figure below :
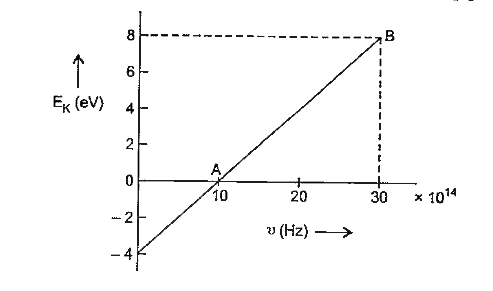
Threshold frequency of the metal.
View SolutionCalculate the maximum kinetic energy of photoelectrons emitted when a light of frequency 2×1016 Hz is irradiated on a metal surface with threshold frequency (v0) equal to 8.68×1015 Hz.
View SolutionPhotoelectric emission is observed from a metallic surface for frequencies v1 and v2 of the incident light rays (v1>v2). If the maximum values of kinetic energy of the photoelectrons emitted in the two cases are in the ratio of 1:k , then the threshold frequency of the metallic surface is
View Solution
