A discharge tube of 2L capacity containing hydrogen gas was evacuated till the pressure inside is 1×10−5atm. If the tube is maintained at a temperature of 27∘C, calculate the number of hydrogen molecules still present in the tube.
To calculate the number of hydrogen molecules present in the discharge tube, we can follow these steps:
Step 1: Convert the temperature to Kelvin
The temperature given is 27°C. To convert this to Kelvin, we use the formula:
T(K)=T(°C)+273
T=27+273=300K
Step 2: Use the Ideal Gas Law to find the number of moles
The Ideal Gas Law is given by the equation:
PV=nRT
Where:
- P = pressure in atm
- V = volume in liters
- n = number of moles
- R = universal gas constant (0.0821 L·atm/(K·mol))
- T = temperature in Kelvin
Given:
- P=1×10−5atm
- V=2L
- R=0.0821L⋅atm/(K⋅mol)
- T=300K
We can rearrange the Ideal Gas Law to solve for n:
n=PVRT
Substituting the values:
n=(1×10−5atm)(2L)(0.0821L⋅atm/(K⋅mol))(300K)
Calculating the denominator:
0.0821×300=24.63
Now, substituting back:
n=2×10−524.63
n≈8.12×10−7moles
Step 3: Convert moles to molecules
To find the number of molecules, we use Avogadro's number:
N=n×NA
Where NA=6.022×1023molecules/mol.
Substituting the values:
N=(8.12×10−7moles)×(6.022×1023molecules/mol)
Calculating:
N≈4.89×1017molecules
Final Answer
The number of hydrogen molecules still present in the tube is approximately:
N≈4.89×1017molecules
---
The temperature given is 27°C. To convert this to Kelvin, we use the formula:
T(K)=T(°C)+273
T=27+273=300K
The Ideal Gas Law is given by the equation:
PV=nRT
Where:
- P = pressure in atm
- V = volume in liters
- n = number of moles
- R = universal gas constant (0.0821 L·atm/(K·mol))
- T = temperature in Kelvin
- P=1×10−5atm
- V=2L
- R=0.0821L⋅atm/(K⋅mol)
- T=300K
n=PVRT
n=(1×10−5atm)(2L)(0.0821L⋅atm/(K⋅mol))(300K)
0.0821×300=24.63
n=2×10−524.63
n≈8.12×10−7moles
To find the number of molecules, we use Avogadro's number:
N=n×NA
Where NA=6.022×1023molecules/mol.
N=(8.12×10−7moles)×(6.022×1023molecules/mol)
N≈4.89×1017molecules
The number of hydrogen molecules still present in the tube is approximately:
N≈4.89×1017molecules
Topper's Solved these Questions
- STATES OF MATTER : GASES AND LIQUIDSBOOK - MODERN PUBLICATIONCHAPTER - STATES OF MATTER : GASES AND LIQUIDSEXERCISE - Practice Problems
54 Videos
- STATES OF MATTER : GASES AND LIQUIDSBOOK - MODERN PUBLICATIONCHAPTER - STATES OF MATTER : GASES AND LIQUIDSEXERCISE - Advanced Level (PROBLEMS)
14 Videos
- SOME BASIC CONCEPTS OF CHEMISTRY BOOK - MODERN PUBLICATIONCHAPTER - SOME BASIC CONCEPTS OF CHEMISTRY EXERCISE - COMPETITION FILE (INTEGER TYPE AND NUMERICAL VALUE TYPE QUESTIONS)
10 Videos
- STRUCTURE OF ATOMBOOK - MODERN PUBLICATIONCHAPTER - STRUCTURE OF ATOMEXERCISE - Unit Practice Test
13 Videos
Similar Questions
Knowledge Check
Two gas bulbs are connected by a thin tube. Calaculate the partial pressure of helium after the connective value is opened at a constant temperature of 27∘C
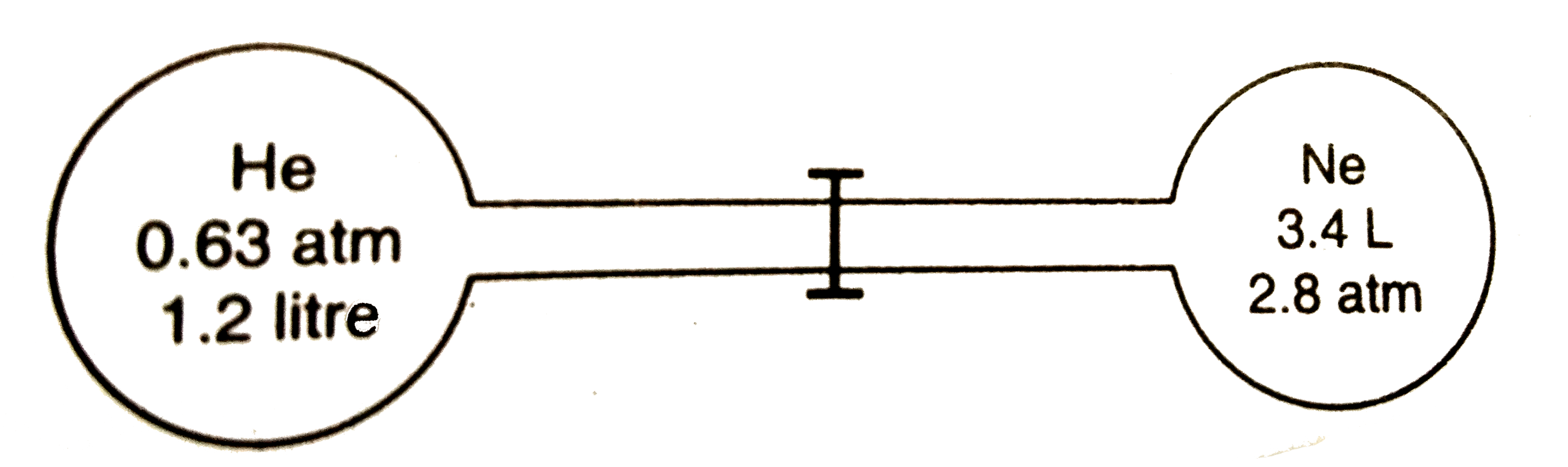 A1 atmB0.328atmC1.64atmD0.166atm
A1 atmB0.328atmC1.64atmD0.166atmAn electron tube was sealed off during manufacture at a pressure of 1.2×10−7 mm of mercury at 27∘C. Its volume is 100 cm3. The number of molecules that remain in the tube is
A6.02×1014B8.2×6.02×1023C24.6×106D8.2×30×6.02×1023An electronic vacuum tube was sealed off during an experiment at a pressure of 8.2×10−10 atm at 27∘C The volume of the tube was 30dm3. The number of gas molecules remaining in the tube are
A6.02×1014B49.4×1023C24.6×106D8.2×30×6.02×1023
10 g of O2 were introduced into an evacuated vessel of 5 litre capacity maintained at 27∘C. Calculate the pressure of the gas in atmosphere in the container.
View SolutionA vessel of capacity 400cm3 contains hydrogen gas at 1 atm pressure at 7∘C. In order to expel 28.57cm3 of the gas at the same pressure, to what temperature the vessel should be heated ?
View Solution5 L of methane gas at 2 atm pressure is compressed to 1.6 L at contant temperature. Calculate the final pressure.
View SolutionAn electron tube was sealed off during manufacture at a pressure of 1.2×10−7 mm of mercury at 27∘C. Its volume is 100 cm3. The number of molecules that remain in the tube is
View SolutionA container contains 1 mole of a gas at 1 atm pressure and 27° C, while its volume is 24.6 litres. If its pressure is 10 atm and temperature 327° C, then new volume is
View Solution
