A metal crystalizes into two cubic phases, face centred cubic (FCC) and body centred cubc (BCC), whose unit cell lengths are 3.5 and 3.0A∘, respectively. Calculate the ratio of densities of FCC and BCC.Solution in Malayalam
1.259
12.59
0.259
0.0259
The correct Answer is:A
Density, d=z×Formula mass of substanceN0×a3
Since for FCC, z = 4 and for BCC, z = 2
dFCC=4×Formula mass of metalN0×(3.5)3anddBCC=2×Formula mass of metalN0×(3)3
Dividing dFCCanddBCC,dFCCdBCC=42×(3)3(3.5)3=2×0.6297=1.259
Topper's Solved these Questions
- SOLID STATEBOOK - BRILLIANT PUBLICATIONCHAPTER - SOLID STATEEXERCISE - LEVEL-II (ASSERTION - REASON TYPE)
20 Videos
- SOLID STATEBOOK - BRILLIANT PUBLICATIONCHAPTER - SOLID STATEEXERCISE - QUESTIONS
14 Videos
- SOLID STATEBOOK - BRILLIANT PUBLICATIONCHAPTER - SOLID STATEEXERCISE - LEVEL-I
50 Videos
- REDOX REACTION & ELECTROCHEMISTRYBOOK - BRILLIANT PUBLICATIONCHAPTER - REDOX REACTION & ELECTROCHEMISTRYEXERCISE - QUESTION (ELECTROCHEMISTRY) (LEVEL -II) (ASSERTION-REASON)
3 Videos
- SOLUTIONSBOOK - BRILLIANT PUBLICATIONCHAPTER - SOLUTIONSEXERCISE - Level-III (Linked Comprehension Type)
12 Videos
Similar Questions
Assertion : In any ionic solid [MX] with Schottky defects, the number of positive and negative ions are same.
Reason : Equal number of cation and anion vacancies are present.
View SolutionA compound of elements X and Y crystallizes in cubic structure where X atoms occupy the corners of the cube and Y atoms occupy the centre of the cube. What is the formula of the compound?
View SolutionThe radius of a di val ent cation M2+ is 94 pm and of divalent anion X2− is 146 pm. Thus, M2X2− has
View SolutionAssertion: After the electrolysis of aqueous CuSO4 solution pH decreases.
Reason: Sulphuric acid is formed.
View Solution1 mole of A and 1 mole B are mixed together to form an ideal solution at 25∘C. Solution is placed in a vessel fitted with piston. If pressure on the solution is reduced isothermally.
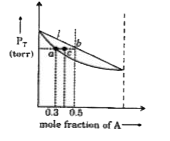
Correct relationship between P∘AandP∘B is
View SolutionA metal crystallizes into two cubic phases, face-centred cubic and body-centred cubic, which have unit cell lengths 3.5 and 3.0A, respectively. Calculate the ration of densities of fcc and bcc.
View SolutionA metal crystallizes into two cubic phases, face-centred cubic and body-centred cubic, which have unit cell lengths 3.5 and 3.0A, respectively. Calculate the ration of densities of fcc and bcc.
View SolutionA metal crystallizes into two cubic phases, face-centred cubic and body-centred cubic, which have unit cell lengths 3.5 and 3.0A, respectively. Calculate the ration of densities of fcc and bcc.
View SolutionA metal crystallises into two cubic phases , face-centred cubic (FCC) and body-centred cubic (BCC) whose unit cell lengths are 3.5 and 3.0 Å respectively. Calculate the ratio of the densities of FCC and BCC
View SolutionA metal crystallizes into two cubic phases , face-centred cubic (fcc) and body -centred cubic (bcc) whose unit cell lengths are 3.5 and 3.0 Å respectively. Calculate the ratio of the densities of fcc and bcc.
View SolutionA Metal crystallises into two cubic faces namely face centered (fcc) and body centered (bcc). Whose unit cell edge length are 3.5Å respectively .Find the ratio of the densities of fcc and bcc.
View SolutionA metal crystallizes into two cubic systems - face centred cubic (fcc) and simple cubic (SC), whose unit cell lengths are 4.5Å and 1.501Å respectively. Calculate the ratio of densities of face centred cubic and Simple cubic.
View SolutionA metal crystallizes into two cubic phases, face-centred cubic and body-centred cubic, which have unit cell lengths 3.5 and 3.0A, respectively. Calculate the ration of densities of fcc and bcc.
View Solutionएक धातु फलक केंद्रित घन (face centred cubic , fcc ) तथा अन्तः केंद्रित घन (body centred cubic, bcc ) , दोनों रूपों में क्रिस्टलीकृत होती है , जिनमें इकाई सेल की भुजा की लम्बाई क्रमश :4.5 तथा 4.0Å है। fcc तथा bcc के घनत्वों के अनुपात की गणना कीजिए।
View SolutionA metal crystallise into two cubic phases FCC and BCC whose unit cell lengths are 2.5 and 2.0 A^0 respectively, the ratio of densities of BCC and FCC
View Solution
