An element with density 3 g cm−3 forms a bcc lattice with edge length of 3×10−8 cm. The molar mass of the element is : (NA=6×1023mol−1)
48.6g mol−1
24.3g mol−1
60g mol−1
56g mol−1
To find the molar mass of the element given its density and lattice structure, we can follow these steps:
1. Identify the Given Data:
- Density (d) = 3 g/cm³
- Edge length (a) = 3 × 10^(-8) cm
- Avogadro's number (N_A) = 6 × 10^(23) mol^(-1)
- For BCC (Body-Centered Cubic) structure, the number of atoms per unit cell (z) = 2.
2. Calculate the Volume of the Unit Cell:
Volume(V)=a3=(3×10−8 cm)3=27×10−24 cm3=2.7×10−23 cm3
3. Use the Density Formula:
The formula for density in terms of molar mass (M) is given by:
d=z⋅MV⋅NA
Rearranging this to find M gives:
M=d⋅V⋅NAz
4. Substitute the Values:
- Substitute the values of density, volume, Avogadro's number, and z into the equation:
M=3 g/cm3⋅2.7×10−23 cm3⋅6×1023 mol−12
5. Calculate M:
- First calculate the numerator:
3⋅2.7×10−23⋅6=48.6×10−23 g mol−1
- Now divide by 2:
M=48.6×10−232=24.3×10−23 g mol−1
- Convert to grams per mole:
M=24.3 g/mol
Final Answer:
The molar mass of the element is 24.3 g/mol.
---
- Density (d) = 3 g/cm³
- Edge length (a) = 3 × 10^(-8) cm
- Avogadro's number (N_A) = 6 × 10^(23) mol^(-1)
- For BCC (Body-Centered Cubic) structure, the number of atoms per unit cell (z) = 2.
Volume(V)=a3=(3×10−8 cm)3=27×10−24 cm3=2.7×10−23 cm3
The formula for density in terms of molar mass (M) is given by:
d=z⋅MV⋅NA
Rearranging this to find M gives:
M=d⋅V⋅NAz
- Substitute the values of density, volume, Avogadro's number, and z into the equation:
M=3 g/cm3⋅2.7×10−23 cm3⋅6×1023 mol−12
- First calculate the numerator:
3⋅2.7×10−23⋅6=48.6×10−23 g mol−1
- Now divide by 2:
M=48.6×10−232=24.3×10−23 g mol−1
- Convert to grams per mole:
M=24.3 g/mol
The molar mass of the element is 24.3 g/mol.
Topper's Solved these Questions
- QUESTION PAPER 2022 TERM 1 SET 2BOOK - XII BOARDS PREVIOUS YEARCHAPTER - QUESTION PAPER 2022 TERM 1 SET 2EXERCISE - SECTION -C
6 Videos
- QUESTION PAPER 2022 TERM 1 SET 2BOOK - XII BOARDS PREVIOUS YEARCHAPTER - QUESTION PAPER 2022 TERM 1 SET 2EXERCISE - SECTION -C
6 Videos
- QUESTION PAPER 2022 TERM 1 SET 1BOOK - XII BOARDS PREVIOUS YEARCHAPTER - QUESTION PAPER 2022 TERM 1 SET 1EXERCISE - SECTION-C
6 Videos
- QUESTION PAPER 2022 TERM 2 SET 1BOOK - XII BOARDS PREVIOUS YEARCHAPTER - QUESTION PAPER 2022 TERM 2 SET 1EXERCISE - SECTION - C
7 Videos
Similar Questions
An element with density 11.2gcm−3 forms a fcc lattice with edge length of 4×10−8 cm. Calculate the atomic mass of the element. Give NA=6.022×1023 atoms mol−1.
View SolutionAn element with density 11.2g cm−3 forms a fcc lattice with edge length of 4×10−8 cm. Calculate the atomic mass of the element.
View Solution
Knowledge Check
An element with density 2.8 gcm−3 forms a f.c.c unit cell with edge length 4×10−8 cm. calculate the molar mass of the element. Given: (NA=6.022×1023)mol−1
A26.97B22C25D21
An element with density 11.2gcm−3 forms a fcc lattice with edge length of 4×10−8 cm. Calculate the atomic mass of the element. [Given:NA=6.022×1023atoms mol−1]
View Solution(i) (a) Following is the schematic alignment of magnetic moments :
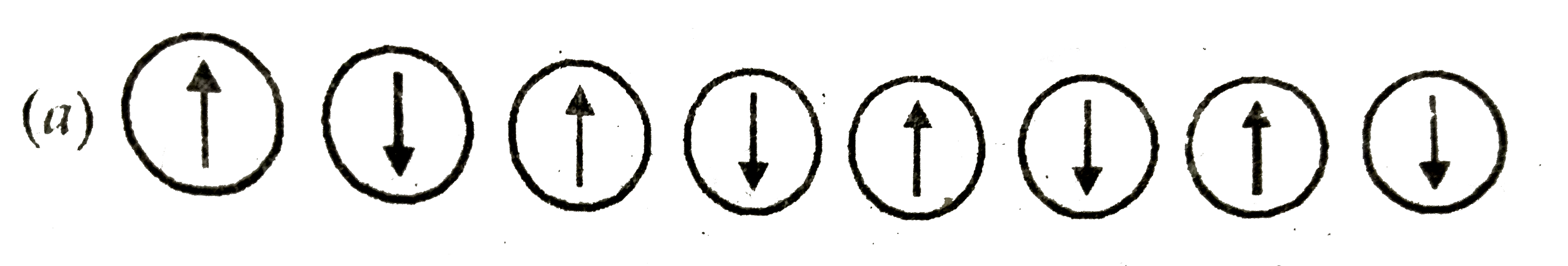
What type of magnetism is shown by this substance ?
(b) What type of stoichiometric defect is shown by (i) KCI (ii) AgCl ?
(ii) An element with density 11.2gcm−3 forms a fcc lattice with length of 4×d10−8 cm. Calculate the atomic mass of the element. (NA=6.02×1023mol−1).
View SolutionAn element with density 2.8gcm−3 forms a fcc unit cell with edge length 4×10−8cm. Calculate the molar mass of the element.
(Given : NA=6.022×1023mol−3 )
View SolutionAn element with density 11.2gcm−3 forms a f. c. c. lattice with edge length of 4×10−8 cm. Calculate the atomic mass of the element. (Given : NA=6.022×1023mol−1
View SolutionAn element with density 2.8 cm3 forms a f.c.c unit cell with edge length 4×10−8 cm. calculate the molar mass of the element. Given: (NA=6.022×1023)mol−1
View SolutionAn element with density 11.2gcm−3 forms a f. c. c. lattice with edge length of 4×10−8 cm. Calculate the atomic mass of the element. (Given : NA=6.022×1023mol−1
View SolutionAn element with density 11.2gcm−3 forms a f. c. c. lattice with edge length of 4×10−8 cm. Calculate the atomic mass of the element. (Given : NA=6.022×1023mol−1
View Solution
