An organic compound (A) (C10H20) on reductive ozonolysis gives 2− methyl butanal. Based on this information, answer the following question.
The total number of stereoisomers possible for (A) is :
8
7
6
4
The correct Answer is:C
To solve the problem, we need to determine the total number of stereoisomers possible for the organic compound (A) with the formula C10H20, which on reductive ozonolysis gives 2-methylbutanal. Here’s a step-by-step breakdown:
Step 1: Identify the structure of the product
The product of the ozonolysis is 2-methylbutanal. The structure of 2-methylbutanal can be represented as follows:
CH3|CH3−CH−CHO|CH2−CH3
Step 2: Determine the structure of compound (A)
Since ozonolysis cleaves double bonds, compound (A) must have a structure that can yield two moles of 2-methylbutanal. The simplest structure that can achieve this is a compound with a double bond that, when cleaved, results in two identical aldehyde products.
A possible structure for compound (A) could be:
CH3∣CH3−CH=CH−CH2−CH3
This structure has a double bond between the second and third carbon atoms.
Step 3: Identify chiral centers in compound (A)
Next, we need to identify if there are any chiral centers in compound (A). A chiral center is typically a carbon atom that is bonded to four different groups.
In the structure of compound (A):
- The second carbon (C2) has the following groups: \( CH3, CH, CH2, H \) (which are different).
- The third carbon (C3) also has the following groups: \( CH3, CH2, CH, H \) (which are different).
Thus, both C2 and C3 are chiral centers.
Step 4: Calculate the number of stereoisomers
The formula to calculate the number of stereoisomers based on the number of chiral centers (n) is given by:
\(
\text{Number of stereoisomers} = 2^n
\)
Here, \( n = 2 \) (since we have two chiral centers).
Thus, the number of stereoisomers is:
\(
2^2 = 4
\)
Step 5: Consider geometric isomerism
Since compound (A) has a double bond, it can also exhibit geometric (cis/trans) isomerism. Each geometric isomer can also have the stereoisomers calculated previously.
For each of the 4 stereoisomers, there are 2 possible geometric isomers (cis and trans). Therefore, the total number of stereoisomers becomes:
\(
4 \text{ (from chiral centers)} \times 2 \text{ (from geometric isomerism)} = 8
\)
Final Answer
The total number of stereoisomers possible for compound (A) is 8.
---
The product of the ozonolysis is 2-methylbutanal. The structure of 2-methylbutanal can be represented as follows:
Since ozonolysis cleaves double bonds, compound (A) must have a structure that can yield two moles of 2-methylbutanal. The simplest structure that can achieve this is a compound with a double bond that, when cleaved, results in two identical aldehyde products.
CH3∣CH3−CH=CH−CH2−CH3
This structure has a double bond between the second and third carbon atoms.
Next, we need to identify if there are any chiral centers in compound (A). A chiral center is typically a carbon atom that is bonded to four different groups.
- The second carbon (C2) has the following groups: \( CH3, CH, CH2, H \) (which are different).
- The third carbon (C3) also has the following groups: \( CH3, CH2, CH, H \) (which are different).
The formula to calculate the number of stereoisomers based on the number of chiral centers (n) is given by:
\(
\text{Number of stereoisomers} = 2^n
\)
Here, \( n = 2 \) (since we have two chiral centers).
\(
2^2 = 4
\)
Since compound (A) has a double bond, it can also exhibit geometric (cis/trans) isomerism. Each geometric isomer can also have the stereoisomers calculated previously.
\(
4 \text{ (from chiral centers)} \times 2 \text{ (from geometric isomerism)} = 8
\)
The total number of stereoisomers possible for compound (A) is 8.
Topper's Solved these Questions
- ISOMERISMBook:CENGAGE CHEMISTRY ENGLISHChapter:ISOMERISMExercise:Multiple choice questions (Exercise)
Explore 38 Videos
- ISOMERISMBook:CENGAGE CHEMISTRY ENGLISHChapter:ISOMERISMExercise:Single correct answer type
Explore 13 Videos
- ISOMERISMBook:CENGAGE CHEMISTRY ENGLISHChapter:ISOMERISMExercise:Exercise
Explore 19 Videos
- IONIC EQUILIBRIUMBook:CENGAGE CHEMISTRY ENGLISHChapter:IONIC EQUILIBRIUMExercise:Archives Subjective
Explore 28 Videos
- NCERT BASED EXERCISEBook:CENGAGE CHEMISTRY ENGLISHChapter:NCERT BASED EXERCISEExercise:Chemical Equilibrium
Explore 73 Videos
Similar Questions
An organic compound (A) (C10H20) on reductive ozonolysis gives 2− methyl butanal. Based on this information, answer the following question.
1. Compound (A) is
View SolutionAn organic compound (A) (C10H20)on reductive ozonolysis gives 2− methyl butanal. Based on this information, answer the following question.
If the stereochemistry about the double bond in (A) is cis, the total number of stereoisomers for (A) is :
View Solution
Knowledge Check
The total number of stereoisomer possible for 2, 3-dichloro butane :
A2B3C4D5An organic compound C4H6 on reductive ozonolysis gives HCHO,CO2andCO3CHO. Find structure of compound.
AH2C=CH−CH=CH2BCH3−CH=C=CH2C DH3C−C≡C−CH3
DH3C−C≡C−CH3
An organic compound (A) (C10H20)on reductive ozonolysis gives 2− methyl butanal. Based on this information, answer the following question.
If the stereochemistry about the double bound in (A) is trans, the total number of stereoisomers for (A) is:
View SolutionAn organic compound (A) (C10H20) on reductive ozonolysis gives 2− methyl butanal. Based on this information, answer the following question.
If the stereochemistry about the double bond in (A) is trans, the total number of enantiomers for (A) is :
View SolutionAn organic compound (A) (C10H20) on reductive ozonolysis gives 2− methyl butanal. Based on this information, answer the following question.
If the stereochemistry about the double bound in (A) is cis, the total number of enantiomers for (A) is :
View SolutionThe total number of stereoisomer possible for 2,3-dichloro butane :
View SolutionConsider the compound (A) given below:
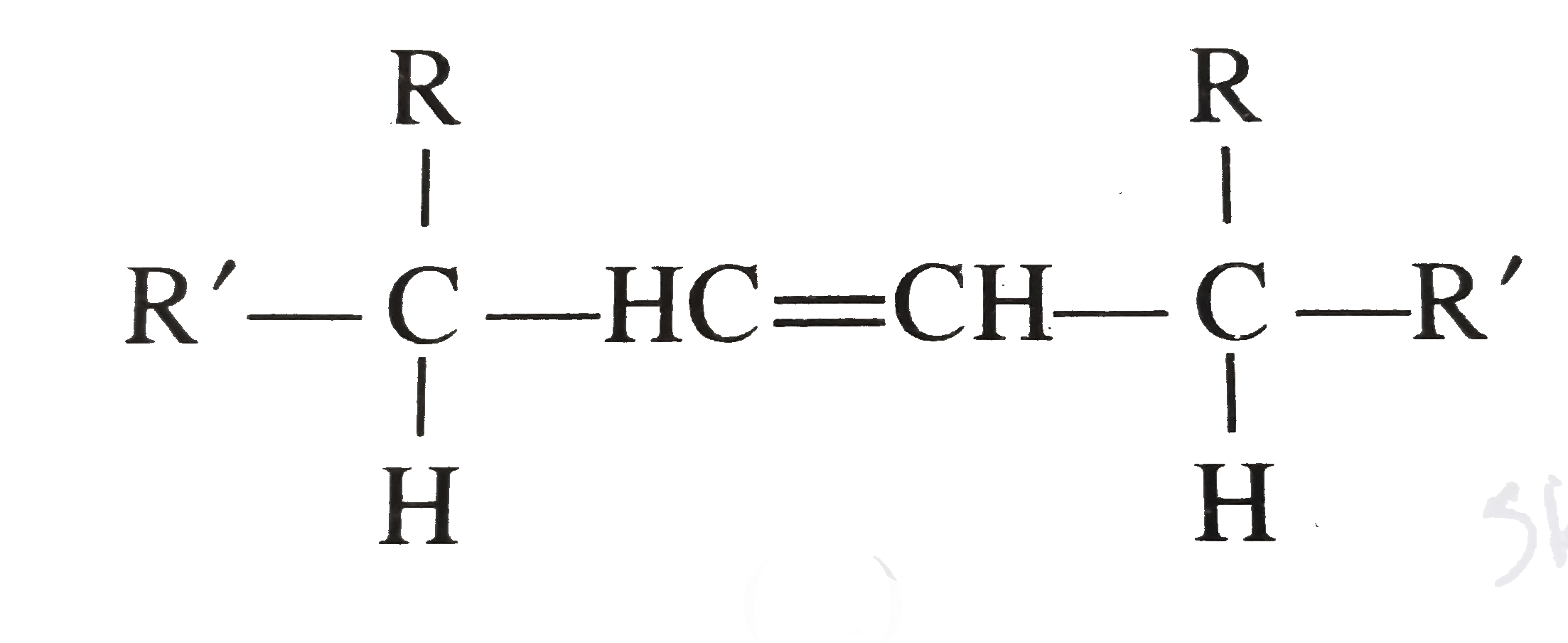
Give the total number of stereoisomers possible for (A).
View SolutionTotal number of stereoisomers possible for the following compound is.
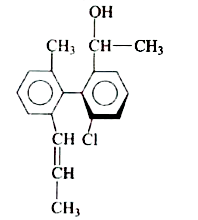
View Solution