Calculate the percentage efficiency of packing in case of body centered cubic crystal.
Packing efficiency : In bcc arrangement, the sphere are touching along the leading diagonal of the cube as shown in the figure .
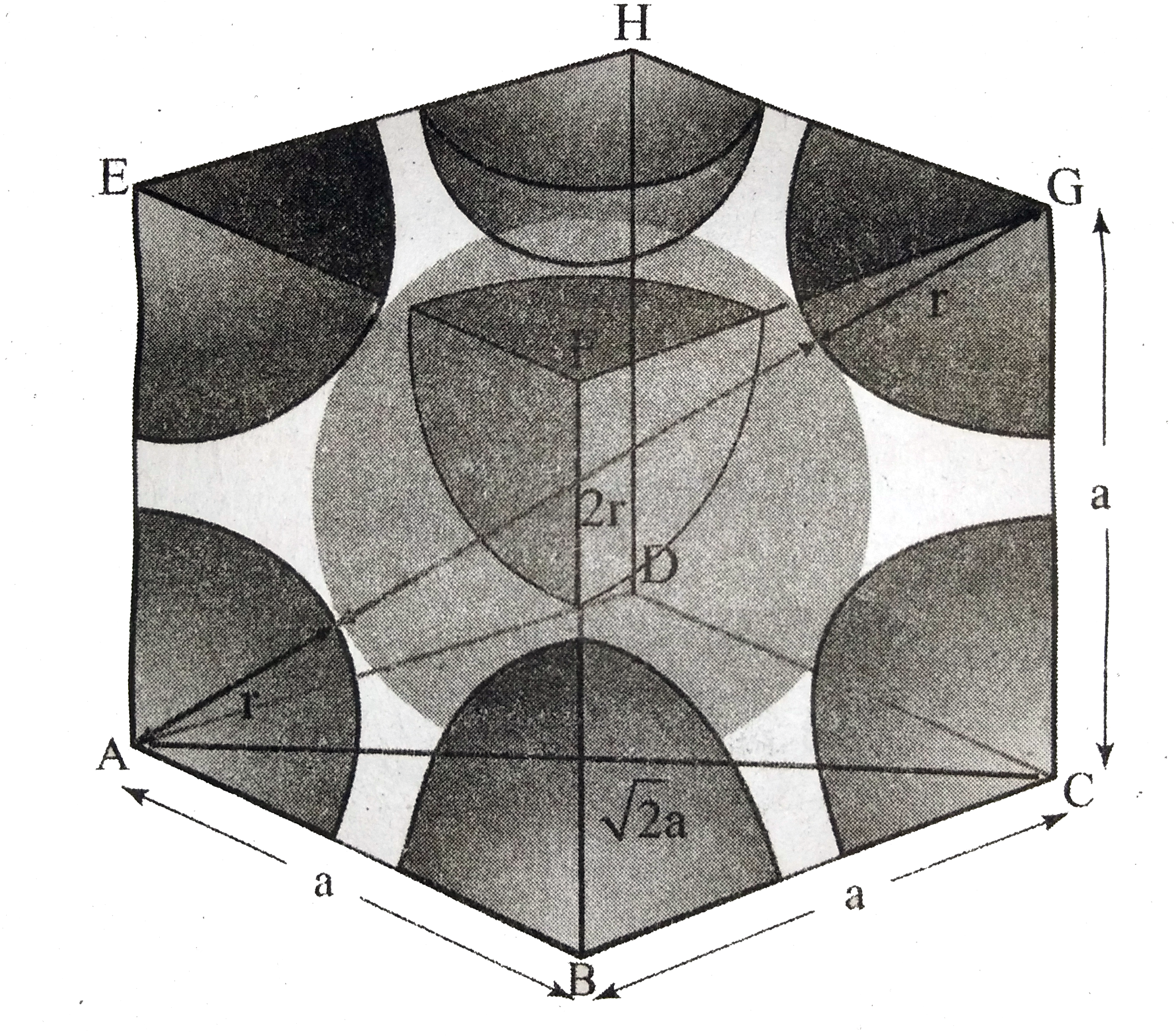
In ΔABC
AC2=AB2+BC2
AC=√AB2+BC2
AC=√a2+a2=√2a2=√2a.
In ΔACG
AG2=√AC2+CG2
AG=√(√2a)2+a2
AG=√3a
i.e., √3a = 4r
r=√34a
∴ Volume of the spher e with radius .r.
=43πr3
=43π(√34a)3
=√316πa3
Number of spheres belong to unit cell in bcc arragement in equal to two and hence the total volume of all spheres.
=2×√3πa316=√3πa38
Dividing (2) by (3)
Packing fraction =(√3πa38)(a3)×100
=√3π8×100
=√3π×12.5
=1.732×3.14×12.5
=68%
i.e., 68% of the available volume is occupied . The available space is used more efficiently than in simple cubic paking .
Topper's Solved these Questions
- SOLID STATE BOOK - PREMIERS PUBLISHERSCHAPTER - SOLID STATE EXERCISE - OTHER IMPORTANT QUESTION &ANSWER ( CHOOSE THE CORRECT ANSWER )
26 Videos
- SOLID STATE BOOK - PREMIERS PUBLISHERSCHAPTER - SOLID STATE EXERCISE - OTHER IMPORTANT QUESTION &ANSWER ( ANSWER THE FOLLOWING :)
59 Videos
- SOLID STATE BOOK - PREMIERS PUBLISHERSCHAPTER - SOLID STATE EXERCISE - EVALUATION TEXTBOOK QUESTIONS & ANSWER (CHOOSE THE CORRECT ANSWERS)
24 Videos
- P-BLOCK ELEMENT IIBOOK - PREMIERS PUBLISHERSCHAPTER - P-BLOCK ELEMENT IIEXERCISE - OTHER IMPORTANT QUESTIONS & ANSWERS (ANSWER THE FOLLOWING QUESTIONS)
244 Videos
- SURFACE CHEMISTRYBOOK - PREMIERS PUBLISHERSCHAPTER - SURFACE CHEMISTRYEXERCISE - OTHER IMPORTANT QUESTIONS & ANSWERS (II. Answer the following questions:)
57 Videos
Similar Questions
Knowledge Check
Calculate the efficiency of packing in case of metal crystal for simple cubic (with the assumption that atoms are touching each other.
A0.524B0.624C0.544D0.724The packing efficiency for a body centred cubic structure is
A0.42B0.53C0.68D0.82
Calculate the efficiency of packing in case of a metal crystal for
a. Simple cubic
b. Body-centred cubic
c. Face-centred cubic (with the assumptions that atoms are touching each other).
View SolutionCalculate the efficiency of packing in case of a metal crystal for
a. Simple cubic
b. Body-centred cubic
c. Face-centred cubic (with the assumptions that atoms are touching each other).
View SolutionCalculate the efficiency of packing in case of a metal crystal for
(i) simple cubic
(ii) body-centred cubic
(iii) face-centred cubic (with the assumptions that atoms are touching each other).
View SolutionCalculate the percentage of packing efficiency in simple cubic unit cell.
View SolutionCalculate the percentage of packing efficiency in simple cubic unit cell.
View SolutionCalculate packing efficinecy in the crystal of body -centred cubic (bcc) structure.
View Solution
