Calculate the weight of copper sulphate formed when 128 g of copper are added to conc. H2SO4. What is the volume of SO2 liberated at S.T.P.?
(Cu= 64, H = 1, S = 32, O = 16)
To solve the problem, we will follow these steps:
Step 1: Write the balanced chemical equation
The reaction between copper (Cu) and concentrated sulfuric acid (H₂SO₄) can be represented by the balanced chemical equation:
Cu+H2SO4→CuSO4+H2O+SO2
Step 2: Calculate the molar mass of copper sulfate (CuSO₄)
The molar mass of CuSO₄ can be calculated as follows:
- Atomic mass of Cu = 64 g/mol
- Atomic mass of S = 32 g/mol
- Atomic mass of O = 16 g/mol
Thus, the molar mass of CuSO₄ is:
Molar mass of CuSO4=64+32+(16×4)=64+32+64=160 g/mol
Step 3: Determine the amount of CuSO₄ produced from 128 g of Cu
From the balanced equation, we see that 64 g of Cu produces 160 g of CuSO₄. Therefore, we can set up a proportion to find out how much CuSO₄ is produced from 128 g of Cu:
If 64 g Cu→160 g CuSO4
Then 128 g Cu→(16064×128) g CuSO4
Calculating this gives:
16064=2.5
So, 128 g Cu→2.5×128=320 g CuSO4
Step 4: Calculate the volume of SO₂ liberated at STP
From the balanced equation, we see that 1 mole of Cu produces 1 mole of SO₂. Therefore, 2 moles of Cu will produce 2 moles of SO₂.
At STP (Standard Temperature and Pressure), 1 mole of any gas occupies 22.4 liters. Since we have 128 g of Cu (which is 2 moles of Cu), we can find the volume of SO₂ produced:
Volume of SO2=2 moles×22.4 L/mole=44.8 L
Final Results
- The weight of copper sulfate formed is 320 g.
- The volume of SO₂ liberated at STP is 44.8 L.
The reaction between copper (Cu) and concentrated sulfuric acid (H₂SO₄) can be represented by the balanced chemical equation:
Cu+H2SO4→CuSO4+H2O+SO2
The molar mass of CuSO₄ can be calculated as follows:
- Atomic mass of Cu = 64 g/mol
- Atomic mass of S = 32 g/mol
- Atomic mass of O = 16 g/mol
Molar mass of CuSO4=64+32+(16×4)=64+32+64=160 g/mol
From the balanced equation, we see that 64 g of Cu produces 160 g of CuSO₄. Therefore, we can set up a proportion to find out how much CuSO₄ is produced from 128 g of Cu:
If 64 g Cu→160 g CuSO4
Then 128 g Cu→(16064×128) g CuSO4
16064=2.5
So, 128 g Cu→2.5×128=320 g CuSO4
From the balanced equation, we see that 1 mole of Cu produces 1 mole of SO₂. Therefore, 2 moles of Cu will produce 2 moles of SO₂.
Volume of SO2=2 moles×22.4 L/mole=44.8 L
- The weight of copper sulfate formed is 320 g.
- The volume of SO₂ liberated at STP is 44.8 L.
Topper's Solved these Questions
- MOLE CONCEPT AND STOICHIOMETRYBOOK - ICSECHAPTER - MOLE CONCEPT AND STOICHIOMETRYEXERCISE - WORKSHEET-7
5 Videos
- MOLE CONCEPT AND STOICHIOMETRYBOOK - ICSECHAPTER - MOLE CONCEPT AND STOICHIOMETRYEXERCISE - Additional Questions for practice
59 Videos
- MOLE CONCEPT AND STOICHIOMETRYBOOK - ICSECHAPTER - MOLE CONCEPT AND STOICHIOMETRYEXERCISE - WORKSHEET-5
7 Videos
- MOLE CONCEPT AND STOICHIOMETRYBOOK - ICSECHAPTER - MOLE CONCEPT AND STOICHIOMETRYEXERCISE - ILLUSTRATIVE ASSIGNMENTS
28 Videos
- ORGANIC CHEMISTRY BOOK - ICSECHAPTER - ORGANIC CHEMISTRY EXERCISE - QUESTIONS FOR PRACTICE PAPER ON EXAMINATION PATTERN (ANSWER THE QUESTIONS)
40 Videos
Similar Questions
Calculate the volume of 320 g of SO2 at S.T.P. (Atomic mass : S = 32 and O = 16).
View SolutionOn adding KMnO4 to cold conc. H2SO4, it gives
View SolutionCalculate
mass of copper sulphate formed when 2.56 g of copper oxide reacts completely as illustrated by the equation below :
[Cu = 64 , S = 32 , O = 16, H = 1]
View SolutionWhat mass of CO will be formed when 9 gm anhydrous oxalic acid is dehydrated with conc H2SO4?
View SolutionCalculate the volume of 320 g of SO2 at STP. (Atomic mass S=32 and O=16)
View SolutionCalculate the volume occupied by 8 g of sulphur dioxide at S. T.P. [S = 32, O = 16)
View SolutionGive reason why?
Conc. H2SO4 is not a drying agent for H2S?
View Solution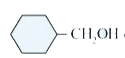 on dehydration with conc. H2SO4 forms predominantly :
on dehydration with conc. H2SO4 forms predominantly :
View SolutionCopper sulphate is prepared by blowing a current of air through copper scrap and dilute H2SO4. Dilute HNO3 is also added:
View SolutionState how addition of I ] copper ii] NaCl to - hot conc . H2SO4 , serves as a test for the latter.
View Solution
