Consider the follwoing two elerctronic transition possibilites in a hydrogen atom as pictured below :

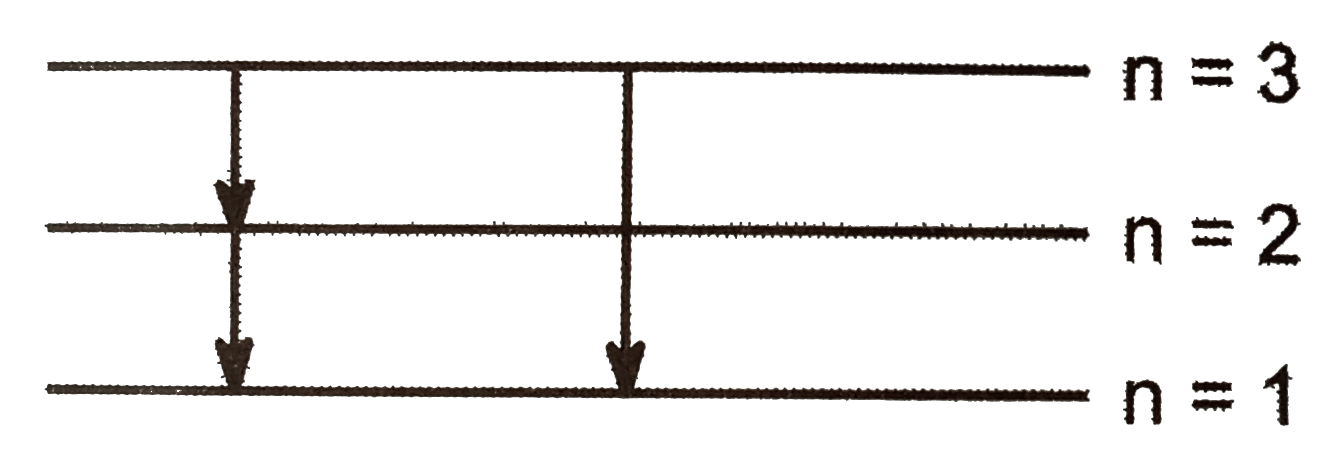
(1) The electron drops from third Bohr's orbit to second Bohr's obit followed with the next transition from second to first Bohr's orbit .
(2) The electron drops from third Bohr's orbit to first Bohr's orbit directly .
Show that :
(a) The sum of the enrgies for the transitions $n = 3$ to $n = 2$ and $n = 2$ to $n = 1$ is equal to the energy of transiton for $n = 3$ to $n = 1$ .
(b) Are wavelengths and frequencies of the emitted spectrum also additive in the same way as their energies are ?

Video Solution

Text Solution

Verified by Experts

Applying, $Δ E = R_{H} [\frac{1}{n_{1}^{2}} - \frac{1}{n_{2}^{2}}]$
For n=3 to n=2,
$Δ E_{3 \to 2} = R_{H} [\frac{1}{2^{2}} - \frac{1}{3^{2}}] = R_{H} \times \frac{5}{36}$ . . .(i)
For n=2 to n=1,
$Δ E_{2 \to 1} = R_{H} [\frac{1}{1^{2}} - \frac{1}{2^{2}}] = R_{H} \times \frac{3}{4}$ . . .. (ii)
For n=3 to n=1,
$Δ E_{3 \to 1} = R_{H} [\frac{1}{1^{2}} - \frac{1}{3^{2}}] = R_{H} \times \frac{8}{9}$ . . .(iii)
Adding equations (i) and (ii).
$R_{H} (\frac{5}{36} + \frac{3}{4}) = R_{H} (\frac{5 + 27}{36}) = R_{H} \times \frac{8}{9}$
thus, $Δ E_{3 \to 1} = Δ E_{3 \to 2} + Δ E_{2 \to 1}$ .

The radius of second Bohr's orbit is
A $0.053 n m$
B $\frac{0.053}{4} n m$
C $0.053 \times 4 n m$
D $0.053 \times 20 n m$
For hydrogen atom, radius of first Bohr's orbit is a for $L i^{2 +}$ the radius of first Bohr's orbit is
A27a
B3a
C $\frac{a}{27}$
D $\frac{a}{3}$
If the speed of electron in the first bohr orbit of hydrogen atom is x then the speed of the electron in the third Bohr orbit of hydrogen is
A $\frac{x^{2}}{9}$
B $\frac{x}{3}$
C $3 x$
D $9 x$