Dry air was passed successively through solution of 5g of a solute in 180g of water and then through pure water. The loss in weight of solution was 2.50g and that of pure solvent 0.04g. The molecualr weight of the solute is:
31.25
3.125
312.5
None
The correct Answer is:A
To find the molecular weight of the solute, we can follow these steps:
1. Identify the Given Data:
- Mass of solute (W_solute) = 5 g
- Mass of solvent (W_solvent) = 180 g
- Loss in weight of solution (ΔW_solution) = 2.50 g
- Loss in weight of pure solvent (ΔW_solvent) = 0.04 g
2. Calculate the Total Loss in Weight:
The total loss in weight when dry air passes through both the solution and the pure solvent is:
ΔWtotal=ΔWsolution+ΔWsolvent=2.50g+0.04g=2.54g
3. Calculate the Relative Lowering of Vapor Pressure:
The relative lowering of vapor pressure (R) can be calculated using the formula:
R=ΔWsolventΔWtotal=0.042.54
4. Calculate the Moles of Solute:
Let the molecular weight of the solute be M g/mol. The number of moles of solute (n_solute) is given by:
nsolute=WsoluteM=5M
5. Calculate the Moles of Solvent:
The molecular weight of water is approximately 18 g/mol. Therefore, the number of moles of solvent (n_solvent) is:
nsolvent=Wsolvent18=18018=10moles
6. Calculate the Mole Fraction of Solute:
The mole fraction of the solute (X_solute) is given by:
Xsolute=nsolutensolute+nsolvent=5M5M+10
7. Set Up the Equation:
From the relative lowering of vapor pressure, we know:
R=Xsolute=0.042.54
Therefore, we can set up the equation:
5M5M+10=0.042.54
8. Cross-Multiply and Solve for M:
Cross-multiplying gives:
5⋅2.54=0.04⋅(5+10M)
Simplifying this:
12.7=0.04⋅5+0.04⋅10M
12.7=0.2+0.4M
12.5=0.4M
M=12.50.4=31.25g/mol
Final Answer:
The molecular weight of the solute is 31.25 g/mol.
- Mass of solute (W_solute) = 5 g
- Mass of solvent (W_solvent) = 180 g
- Loss in weight of solution (ΔW_solution) = 2.50 g
- Loss in weight of pure solvent (ΔW_solvent) = 0.04 g
The total loss in weight when dry air passes through both the solution and the pure solvent is:
ΔWtotal=ΔWsolution+ΔWsolvent=2.50g+0.04g=2.54g
The relative lowering of vapor pressure (R) can be calculated using the formula:
R=ΔWsolventΔWtotal=0.042.54
Let the molecular weight of the solute be M g/mol. The number of moles of solute (n_solute) is given by:
nsolute=WsoluteM=5M
The molecular weight of water is approximately 18 g/mol. Therefore, the number of moles of solvent (n_solvent) is:
nsolvent=Wsolvent18=18018=10moles
The mole fraction of the solute (X_solute) is given by:
Xsolute=nsolutensolute+nsolvent=5M5M+10
From the relative lowering of vapor pressure, we know:
R=Xsolute=0.042.54
Therefore, we can set up the equation:
5M5M+10=0.042.54
Cross-multiplying gives:
5⋅2.54=0.04⋅(5+10M)
Simplifying this:
12.7=0.04⋅5+0.04⋅10M
12.7=0.2+0.4M
12.5=0.4M
M=12.50.4=31.25g/mol
The molecular weight of the solute is 31.25 g/mol.
Topper's Solved these Questions
- SOLUTIONSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLUTIONSEXERCISE - Exercise (Assertion-Reasoning)
18 Videos
- SOLUTIONSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLUTIONSEXERCISE - Exercise (Interger)
8 Videos
- SOLUTIONSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLUTIONSEXERCISE - Exercises (Multiple Correct)
25 Videos
- SOLID STATEBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLID STATEEXERCISE - Ex 1.2 (Objective)
9 Videos
- SURFACE CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SURFACE CHEMISTRYEXERCISE - Archives Subjective
2 Videos
Similar Questions
Lowering in vapour pressure is determined by Ostwald and Walker dynamic methed. It is based on the prinicipal , that when air is allowed to pass through a solvent or solution, it takes up solventvapour with it to get itself saturated at that temperature
I and II are weighted separately before and after passing dry air. Loss in mass of each set, gives the lowing of vapour pressure. The temperature of air, the solution and the solvent is kept constant.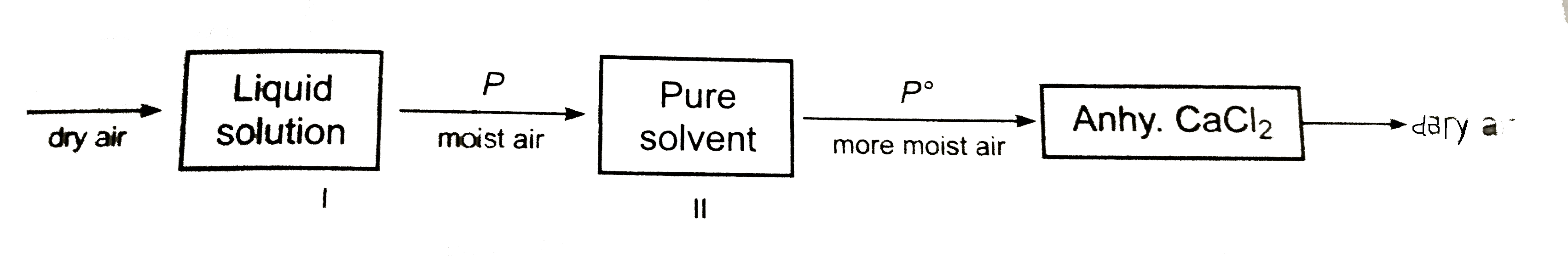
Dry air was passed thorough 9.24 g of solute in 108 g of water and then through pure water. The loss in mass of solution was 3.2 g and that of pure water 0.08 g . The molecular mass (g/mol) of solute is nearly :
(a)50
(b)62
(c)70
(d)80
View SolutionA solution is prepared by dissolved 4 g of a solute in 18 g of water. Calculate the mass percent of the solute.
View SolutionA solution is prepared by dissolved 2 g of a solute in 36 g of water. Calculate the mass percent of the solute.
View SolutionSolute potential of pure water and its solution are :
View SolutionVapour pressure of a solution of 5gof non-electrolyte in 100gwater at a particular temperature is2985N/m2.The vapour pressure of pure water is3000N/m2.The molecular weight of the solute is
View SolutionThe vapour pressure of benzene at 90∘C is 1020 torr. A solution of 5g of a solute in 58.5g benzene has vapour pressure 990 torr. The molecular weight of the solute is?
View SolutionA solution is prepared by adding 5 g of a substance x to 18 g of water. Calculate the mass percent of the solute.
View SolutionA solution is prepared by adding 5 g of a substance x to 18 g of water. Calculate the mass percent of the solute.
View SolutionThree grams of salt of molecular weight 30 is dissolved in 250g of water. The molality of the solution is….
View SolutionA solution is prepared by adding 2 g of a substance A to 18 g of water. Calculate the mass per cent of the solute.
View Solution
