Energy required to ionise 2 mole of gaseous ion present in its ground state is :
eV
eV
eV
eV
The correct Answer is:B
To find the energy required to ionize 2 moles of gaseous He+ ions in their ground state, we can follow these steps:
Step 1: Understand the Ionization Energy Formula
The ionization energy (IE) for a hydrogen-like atom or ion is given by the formula:
\(
IE = \frac{13.6 \, \text{eV} \cdot Z^2}{n^2}
\)
where:
- \( Z \) is the atomic number,
- \( n \) is the principal quantum number (for ground state, \( n = 1 \)).
Step 2: Identify the Values for Helium Ion
For the helium ion He+:
- \( Z = 2 \) (since helium has an atomic number of 2),
- \( n = 1 \) (ground state).
Step 3: Substitute the Values into the Formula
Substituting the values into the ionization energy formula:
\(
IE = \frac{13.6 \, \text{eV} \cdot (2)^2}{(1)^2} = \frac{13.6 \, \text{eV} \cdot 4}{1} = 54.4 \, \text{eV}
\)
Step 4: Calculate the Energy for 2 Moles
Now, we need to calculate the energy required for 2 moles of He+ ions. We know that 1 mole contains Avogadro's number (\( N_A \)) of ions:
\(
\text{Energy for 2 moles} = 54.4 \, \text{eV} \times 2 \times N_A
\)
\(
= 108.8 \, N_A \, \text{eV}
\)
Final Answer
The energy required to ionize 2 moles of gaseous He+ ions in their ground state is:
\(
\text{Energy} = 108.8 \, N_A \, \text{eV}
\)
The ionization energy (IE) for a hydrogen-like atom or ion is given by the formula:
\(
IE = \frac{13.6 \, \text{eV} \cdot Z^2}{n^2}
\)
where:
- \( Z \) is the atomic number,
- \( n \) is the principal quantum number (for ground state, \( n = 1 \)).
For the helium ion He+:
- \( Z = 2 \) (since helium has an atomic number of 2),
- \( n = 1 \) (ground state).
Substituting the values into the ionization energy formula:
\(
IE = \frac{13.6 \, \text{eV} \cdot (2)^2}{(1)^2} = \frac{13.6 \, \text{eV} \cdot 4}{1} = 54.4 \, \text{eV}
\)
Now, we need to calculate the energy required for 2 moles of He+ ions. We know that 1 mole contains Avogadro's number (\( N_A \)) of ions:
\(
\text{Energy for 2 moles} = 54.4 \, \text{eV} \times 2 \times N_A
\)
\(
= 108.8 \, N_A \, \text{eV}
\)
The energy required to ionize 2 moles of gaseous He+ ions in their ground state is:
\(
\text{Energy} = 108.8 \, N_A \, \text{eV}
\)
Topper's Solved these Questions
- ATOMIC STUCTUREBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - ATOMIC STUCTUREEXERCISE - level 2
30 Videos
- ATOMIC STUCTUREBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - ATOMIC STUCTUREEXERCISE - Level 3 (Passage 1)
3 Videos
- CHEMICAL EQUILIBRIUMBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Match the column
1 Videos
Similar Questions
Energy required to ionise 1 mole of gaseousHe+ion present in its ground state is
View SolutionThe energy required to remove an electron from an isolated gaseous atom in its ground state is called ionisation enthalpy.From the following,select the element having maximum value of 2ndI.E. a) Be b)C c)N d)O
View Solution
Knowledge Check
the energy required to excite an electron in hydrogen atom to its first excited state is
A8.5eVB10.2eVC12.7eVD13.6eVIf the ionization energy for the hydrogen atom is 13.6 eV , the energy required to excite it from the ground state to the next higher state is nearly
A3.4 eVB10.2 eVC17.2 eVD13.6 eV
The lattice enthalpy of an ionic compound is the enthalpy when one mole of an ionic compoud present in its gaseous state, dissociates into its ions. It is impossible to determine it directly by experiment. Suggest and explain in indirect method to measure lattice enthalpy of NaCl(s).
View SolutionThe ionisation energy of Li is 500 KJ/mole. The amount of energy required to convert 70 mg of Li atoms in gaseous state into Li+ ion (in KJ) is
View SolutionWhat is the energy of the electron in He+ ion in the ground state ?
View SolutionHow many unpaired electrons ae in a Fe2+ ion in the ground state?
View SolutionIonisation energy is the amount of energy required to remove the outermost e− from a gaseous atom. It's unit is kJ/mole or kcal/mole.
Successive ionisation energy - It is the amount of energy required to remove electron successively from a gaseous ion. These are termed as IE2,IE3,IE4 etc. the difference in the values of IE1,IE2 and IE3 helps to determine electronic configuration of the element.
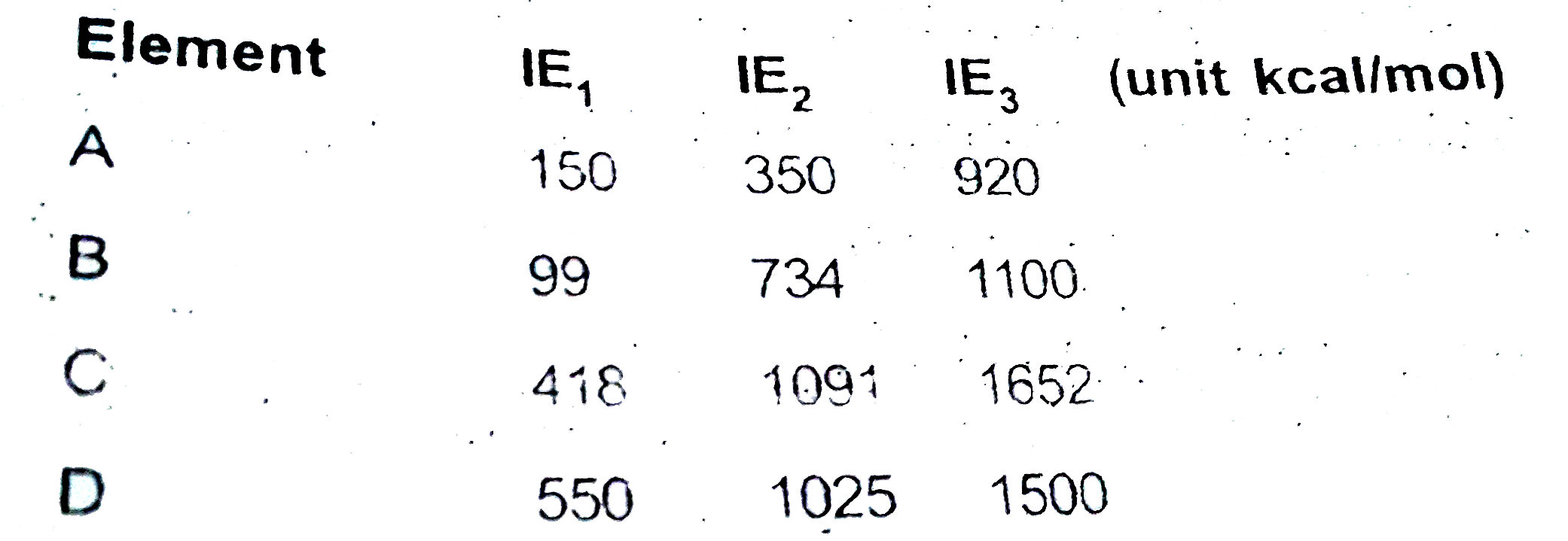
Which element is a noble gas ?
View SolutionIonisation energy is the amount of energy required to remove the outermost e− from a gaseous atom. It's unit is kJ/mole or kcal/mole.
Successive ionisation energy - It is the amount of energy required to remove electron successively from a gaseous ion. These are termed as IE2,IE3,IE4 etc. the difference in the values of IE1,IE2 and IE3 helps to determine electronic configuration of the element.

Which of the following is a non-metal ?
View Solution
