How many moles of CO2 will be formed when a mixture containing
10 moles each of Li2CO3 and Na2CO3 are heated ?
To solve the question of how many moles of CO2 will be formed when a mixture containing 10 moles each of Li2CO3 and Na2CO3 are heated, we can follow these steps:
Step 1: Understand the stability of the carbonates
- Lithium carbonate (Li2CO3) is less stable when heated and will decompose.
- Sodium carbonate (Na2CO3) is stable and will not decompose upon heating.
Step 2: Write the decomposition reaction for lithium carbonate
- The decomposition reaction for lithium carbonate is:
\(
\text{Li}_2\text{CO}_3 \rightarrow \text{Li}_2\text{O} + \text{CO}_2
\)
- This reaction shows that 1 mole of Li2CO3 produces 1 mole of CO2.
Step 3: Calculate the amount of CO2 produced from Li2CO3
- Since we have 10 moles of Li2CO3, we can use the stoichiometry of the reaction:
\(
10 \text{ moles of Li}_2\text{CO}_3 \rightarrow 10 \text{ moles of CO}_2
\)
- Therefore, from 10 moles of Li2CO3, we will get 10 moles of CO2.
Step 4: Determine the contribution from Na2CO3
- Since Na2CO3 does not decompose upon heating, it does not produce any CO2.
- Therefore, the contribution from Na2CO3 is 0 moles of CO2.
Step 5: Combine the results
- Total moles of CO2 produced = moles from Li2CO3 + moles from Na2CO3
- Total moles of CO2 = 10 moles + 0 moles = 10 moles.
Final Answer
- The total number of moles of CO2 formed when the mixture is heated is 10 moles.
---
- Lithium carbonate (Li2CO3) is less stable when heated and will decompose.
- Sodium carbonate (Na2CO3) is stable and will not decompose upon heating.
- The decomposition reaction for lithium carbonate is:
\(
\text{Li}_2\text{CO}_3 \rightarrow \text{Li}_2\text{O} + \text{CO}_2
\)
- This reaction shows that 1 mole of Li2CO3 produces 1 mole of CO2.
- Since we have 10 moles of Li2CO3, we can use the stoichiometry of the reaction:
\(
10 \text{ moles of Li}_2\text{CO}_3 \rightarrow 10 \text{ moles of CO}_2
\)
- Therefore, from 10 moles of Li2CO3, we will get 10 moles of CO2.
- Since Na2CO3 does not decompose upon heating, it does not produce any CO2.
- Therefore, the contribution from Na2CO3 is 0 moles of CO2.
- Total moles of CO2 produced = moles from Li2CO3 + moles from Na2CO3
- Total moles of CO2 = 10 moles + 0 moles = 10 moles.
- The total number of moles of CO2 formed when the mixture is heated is 10 moles.
Topper's Solved these Questions
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - S-BLOCK GROUP 1 - ALKALI METALSEXERCISE - Ex 4.1 (Subjective)24videos
S-BLOCK GROUP 1 - ALKALI METALS
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - S-BLOCK GROUP 1 - ALKALI METALSEXERCISE - Ex 4.1 (True/False)1videos
S-BLOCK GROUP 1 - ALKALI METALS
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - S-BLOCK GROUP 1 - ALKALI METALSEXERCISE - Archives Subjective8videos
S-BLOCK GROUP 1 - ALKALI METALS
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - REDOX REACTIONSEXERCISE - Archives (Integers)1videos
REDOX REACTIONS
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - S-BLOCK GROUP 2 - ALKALINE EARTH METALSEXERCISE - Ex 5.1 Objective2videos
S-BLOCK GROUP 2 - ALKALINE EARTH METALS
Similar Questions
On heating a mixture containing 1 mole each of Li2CO3andK2CO3, x mole of CO2 is//are formed. Find the value of x.
View SolutionOn heating 8 moles each of Li2CO3 and K2CO3, how many moles of CO2 evolved ?
View SolutionOne mole of CO2 contains:
View SolutionHow are 0.50molNa2CO3 and 0.50MNa2CO3 different?
View SolutionThe equilibrium constant for the reaction CO(g)+H2O(g)⇔CO2(g)+H2(g)is5 how many moles of CO2 must be added to 1 litre container alrady containing 3 moles each of CO and H2O to make 2 M equilibrium conentration of CO?
View SolutionNa2CO3.10H2O is
View SolutionHow many litres of CO2 gas at STP will react with 2.5 moles of KOH exactly to form K2CO3?
View SolutionHow many litres of CO2 at STP wil be formed when 100mL of 0.1MH2SO4 reacts with excess of Na2CO3?
View SolutionHow many moles of CO2 will released when following compound treated with heat?
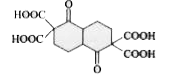 View Solution
View SolutionHow many moles of CO2 is produced when one mole of benzene undergoes combustion ?
View Solution

