How many optically active stereoisomers are possible for lactic acid ?
1
2
4
3
The correct Answer is:b
To determine how many optically active stereoisomers are possible for lactic acid, we can follow these steps:
Step 1: Understand the Structure of Lactic Acid
Lactic acid has the molecular formula C3H6O3. The structure can be represented as:
- A central carbon atom (C) connected to:
- A hydroxyl group (OH)
- A methyl group (CH3)
- A hydrogen atom (H)
- A carboxyl group (COOH)
Step 2: Identify Chiral Centers
A chiral center is a carbon atom that is bonded to four different groups. In lactic acid, we analyze the carbon atoms:
- The first carbon (attached to CH3) has three hydrogens and is not chiral.
- The second carbon (attached to OH, H, CH3, and COOH) has four different groups and is chiral.
- The third carbon (part of the carboxyl group) is also not chiral since it is part of a double bond.
Thus, there is only one chiral center in lactic acid.
Step 3: Calculate the Number of Optically Active Stereoisomers
The formula to calculate the number of optically active stereoisomers is given by:
Number of stereoisomers=2n
where n is the number of chiral centers.
Since we have identified that there is 1 chiral center in lactic acid:
Number of stereoisomers=21=2
Conclusion
Therefore, lactic acid can have 2 optically active stereoisomers.
Final Answer
The number of optically active stereoisomers possible for lactic acid is 2.
---
Lactic acid has the molecular formula C3H6O3. The structure can be represented as:
- A central carbon atom (C) connected to:
- A hydroxyl group (OH)
- A methyl group (CH3)
- A hydrogen atom (H)
- A carboxyl group (COOH)
A chiral center is a carbon atom that is bonded to four different groups. In lactic acid, we analyze the carbon atoms:
- The first carbon (attached to CH3) has three hydrogens and is not chiral.
- The second carbon (attached to OH, H, CH3, and COOH) has four different groups and is chiral.
- The third carbon (part of the carboxyl group) is also not chiral since it is part of a double bond.
The formula to calculate the number of optically active stereoisomers is given by:
Number of stereoisomers=2n
where n is the number of chiral centers.
Number of stereoisomers=21=2
Therefore, lactic acid can have 2 optically active stereoisomers.
The number of optically active stereoisomers possible for lactic acid is 2.
Topper's Solved these Questions
- ORGANIC CHEMISTRY SOME BASIC PRINCIPLES AND TECHNIQUES BOOK - DISHA PUBLICATIONCHAPTER - ORGANIC CHEMISTRY SOME BASIC PRINCIPLES AND TECHNIQUES EXERCISE - Exercise - 1 : Concept Builder (Topicwise) TOPIC 3 : Concepts of Reaction Mechanism in Organic Compounds and Purification
31 Videos
- ORGANIC CHEMISTRY SOME BASIC PRINCIPLES AND TECHNIQUES BOOK - DISHA PUBLICATIONCHAPTER - ORGANIC CHEMISTRY SOME BASIC PRINCIPLES AND TECHNIQUES EXERCISE - Exercise - 2 : Concept Applicator
30 Videos
- ORGANIC CHEMISTRY SOME BASIC PRINCIPLES AND TECHNIQUES BOOK - DISHA PUBLICATIONCHAPTER - ORGANIC CHEMISTRY SOME BASIC PRINCIPLES AND TECHNIQUES EXERCISE - Exercise - 1 : Concept Builder Builder (Topicwise) TOPIC 1 : Classification and Nomenclature of Organic Compounds)
22 Videos
- JEE MAIN 2019BOOK - DISHA PUBLICATIONCHAPTER - JEE MAIN 2019EXERCISE - MCQs
30 Videos
- POLYMERS BOOK - DISHA PUBLICATIONCHAPTER - POLYMERS EXERCISE - EXERCISE -2 : CONCEPT APPLICATOR
30 Videos
Similar Questions
Knowledge Check
How many optically active isomers are possible for
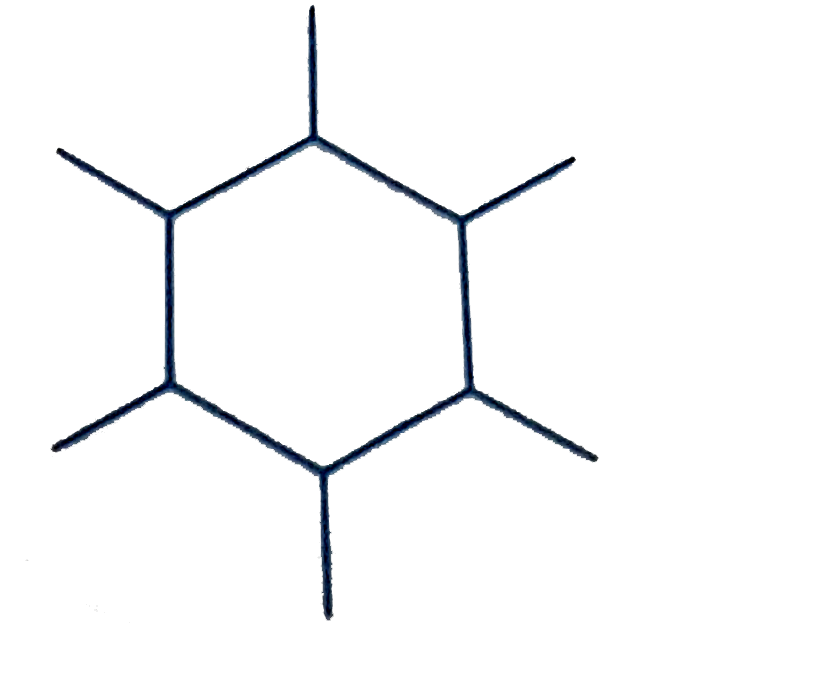 A2B4C6D8
A2B4C6D8How many optically active stereoisomers are possible for butane-2, 3-diol ?
A1B2C3D4How many optically active stereoisomers are possible for butane-2, 3-diol ?
A1B2C3D4
How many optically active stereoisomers are possible for butane-2, 3-diol ?
View SolutionHow many optically active stereoisomers are possible for butane-2,3-diol
View SolutionHow many optically active stereoisomers are possible for tri-sec-buty1methane?
View SolutionHow many stereoisomers are possible for
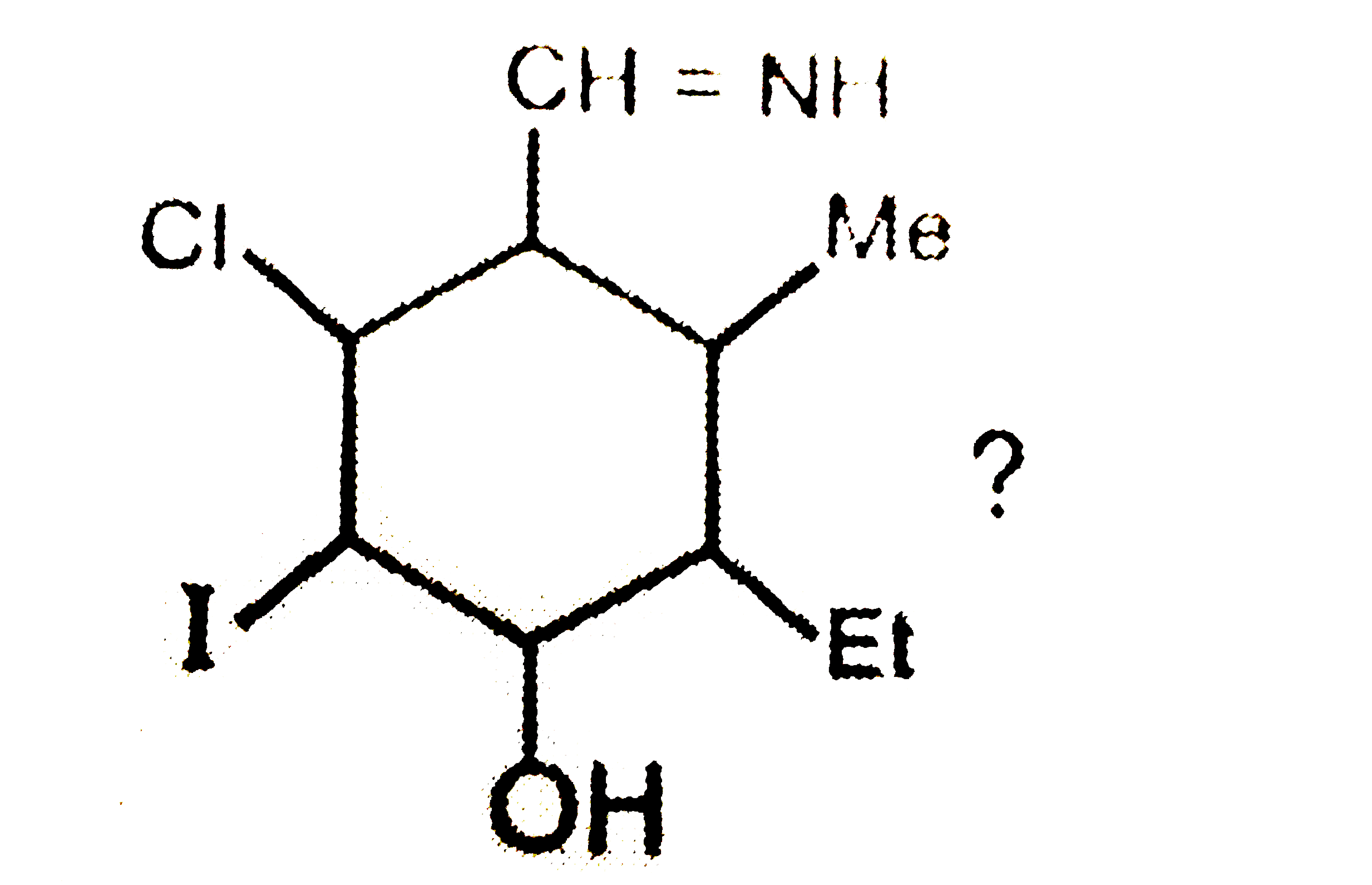
View SolutionHow many optically active stereoisomers are possoble for 2, 3 - butanediol ?
View Solution
