Identify the reaction order if the unit of rate constant iss−1.
To determine the reaction order from the unit of the rate constant, which is given as s−1, we can follow these steps:
1. Understand the Unit of Rate Constant (K):
The unit of the rate constant (K) is given as s−1, which indicates that it has the dimensions of inverse time.
2. General Expression for Rate Law:
The rate of a reaction can be expressed as:
\(
R = k (A)^n
\)
where:
- \( R \) is the rate of the reaction,
- \( k \) is the rate constant,
- \( (A) \) is the concentration of the reactant,
- \( n \) is the order of the reaction.
3. Identify Units of Rate (R):
The unit of the rate \( R \) is typically expressed in terms of concentration per time, which is:
\(
\text{Unit of } R = \text{mol L}^{-1} \text{s}^{-1}
\)
4. Relate Units of Rate and Rate Constant:
Rearranging the rate equation gives:
\(
k = \frac{R}{(A)^n}
\)
Thus, the unit of \( k \) can be expressed as:
\(
\text{Unit of } k = \frac{\text{Unit of } R}{\text{Unit of } (A)^n}
\)
5. Substituting Units:
Substituting the units we have:
\(
\text{Unit of } k = \frac{\text{mol L}^{-1} \text{s}^{-1}}{(\text{mol L}^{-1})^n}
\)
Simplifying this gives:
\(
\text{Unit of } k = \text{mol L}^{-1} \text{s}^{-1} \times \text{(mol L}^{-1})^{-n} = \text{mol L}^{-1} \text{s}^{-1} \times \text{mol}^{-n} \text{L}^n
\)
6. Equating Units:
This can be simplified to:
\(
\text{Unit of } k = \text{mol}^{1-n} \text{L}^{-1+n} \text{s}^{-1}
\)
Since we know that the unit of \( k \) is \( s^{-1} \), we can equate:
\(
\text{mol}^{1-n} \text{L}^{-1+n} \text{s}^{-1} = \text{s}^{-1}
\)
7. Setting Up Equations:
From the above equation, we can derive two conditions:
- For the concentration term: \( 1 - n = 0 \) (implying \( n = 1 \))
- For the time term: This is already satisfied as both sides have \( s^{-1} \).
8. Conclusion:
Therefore, the order of the reaction \( n \) is 1, indicating that it is a first-order reaction.
The unit of the rate constant (K) is given as s−1, which indicates that it has the dimensions of inverse time.
The rate of a reaction can be expressed as:
\(
R = k (A)^n
\)
where:
- \( R \) is the rate of the reaction,
- \( k \) is the rate constant,
- \( (A) \) is the concentration of the reactant,
- \( n \) is the order of the reaction.
The unit of the rate \( R \) is typically expressed in terms of concentration per time, which is:
\(
\text{Unit of } R = \text{mol L}^{-1} \text{s}^{-1}
\)
Rearranging the rate equation gives:
\(
k = \frac{R}{(A)^n}
\)
Thus, the unit of \( k \) can be expressed as:
\(
\text{Unit of } k = \frac{\text{Unit of } R}{\text{Unit of } (A)^n}
\)
Substituting the units we have:
\(
\text{Unit of } k = \frac{\text{mol L}^{-1} \text{s}^{-1}}{(\text{mol L}^{-1})^n}
\)
Simplifying this gives:
\(
\text{Unit of } k = \text{mol L}^{-1} \text{s}^{-1} \times \text{(mol L}^{-1})^{-n} = \text{mol L}^{-1} \text{s}^{-1} \times \text{mol}^{-n} \text{L}^n
\)
This can be simplified to:
\(
\text{Unit of } k = \text{mol}^{1-n} \text{L}^{-1+n} \text{s}^{-1}
\)
Since we know that the unit of \( k \) is \( s^{-1} \), we can equate:
\(
\text{mol}^{1-n} \text{L}^{-1+n} \text{s}^{-1} = \text{s}^{-1}
\)
From the above equation, we can derive two conditions:
- For the concentration term: \( 1 - n = 0 \) (implying \( n = 1 \))
- For the time term: This is already satisfied as both sides have \( s^{-1} \).
Therefore, the order of the reaction \( n \) is 1, indicating that it is a first-order reaction.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - CHEMICAL KINETICSEXERCISE - EXERCISE (PART- I (OBJECTIVE QUESRTIONS)A.FILL IN THE BLANKS)54videos
CHEMICAL KINETICS
- BOOK - ICSECHAPTER - CHEMICAL KINETICSEXERCISE - EXERCISE (PART- I (OBJECTIVE QUESRTIONS)B.COMPLE THE FOLLOWING STATEMENTS BY SELECTING THE CORRECT ALTERNATIVE FROM THE CHOICES GIVEN )105videos
CHEMICAL KINETICS
- BOOK - ICSECHAPTER - CHEMICAL KINETICSEXERCISE - PROBLEM165videos
CHEMICAL KINETICS
- BOOK - ICSECHAPTER - BIOMOLECULESEXERCISE - ISC EXAMINATION QUSTIONS (PART-I)(DESCRIPTIVE QUESTIONS)21videos
BIOMOLECULES
- BOOK - ICSECHAPTER - CHEMISTRY IN EVERYDAY LIFEEXERCISE - EXERCISE (PART - II (DESCRIPTIVE QUESTIONS) (LONG ANSWER QUESTIONS))19videos
CHEMISTRY IN EVERYDAY LIFE
Similar Questions
Knowledge Check
- Question 1 - Select One
What will be the half-life of the first order reaction for which the value of rate constant is 200s−1?
A3.46×10−2xB3.46×10−3sC4.26×10−2sD4.26×10−3s
What do you understand by the order of a reaction ? Identify the reaction order from each of the following units of the reaction rate constant :
(i) L−1mols−1
(ii) Lmols−1View Solution(a) For a reaction ,A+B→Product the rate law is given rate =k[A]1[B]2 .What is the order of the reaction ?
(b) write the unit of rate constant k for the first order reactionView SolutionFor a general reaction X→Y, the plot of conc. Of X vx time is given in the figure. What is the order of the reaction and what are the units of rate constant?
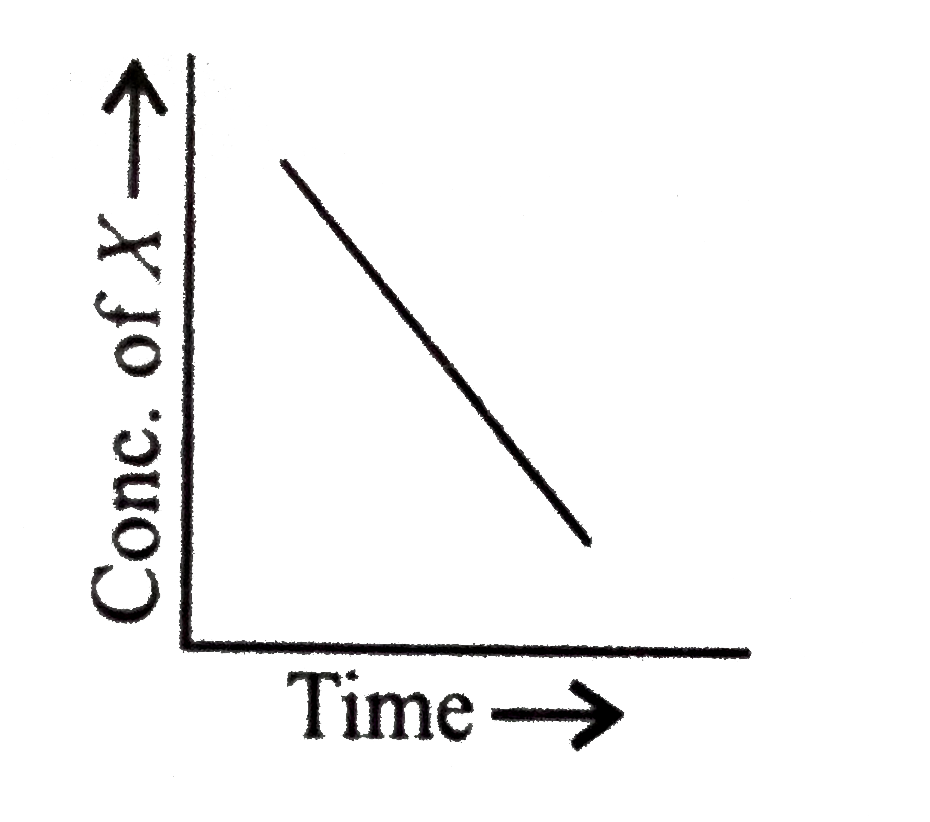 View Solution
View SolutionIdentify the order of reaction from each of the following units of rate constant (k) : (a)moleL−1sec−1 (b) Mol−1sec−1
View SolutionIdentify the order of the reaction from the rate constant value =3.2×10−5Lmol−1s−1.
View SolutionFor which order of the reaction, the units of the rate constant are independent of the concentration ?
View SolutionFor which order of the reaction, the units of the rate constant are independent of the concentration ?
View Solution
