In BX3 B - X distance is shorter than what is expected theoretically because (X = F, CI, Br, I)
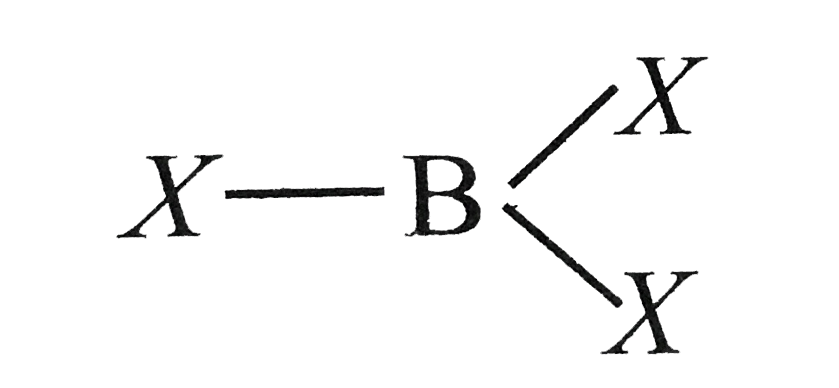
sp3 hybridisation of B is responsible for shorter B - X distance
B- X has a double bond character due to back-bonding
Dimerisation takes place in BX3 which is responsible for shorter B - X distance.
Due to large size, of X, B - X distance decreases.
The correct Answer is:B
Due to back-bonding B- X in BX3 has double bond character which because of resonance result in shorter B-X distance
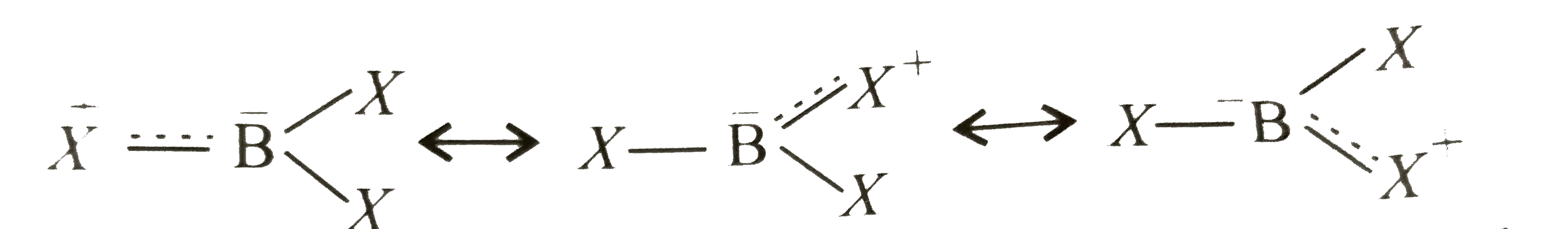
Topper's Solved these Questions
- THE P-BLOCK ELEMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THE P-BLOCK ELEMENTSEXERCISE - HIGHER ORDER THINKING SKILLS
9 Videos
- THE P-BLOCK ELEMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THE P-BLOCK ELEMENTSEXERCISE - EXEMPLAR PROBLEMS
15 Videos
- STRUCTURE OF ATOM BOOK - NCERT FINGERTPS ENGLISHCHAPTER - STRUCTURE OF ATOM EXERCISE - Assertion And Reason
15 Videos
- THE S-BLOCK ELEMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THE S-BLOCK ELEMENTSEXERCISE - Assertion And Reason
15 Videos
Similar Questions
Knowledge Check
If f(x)=3x3−2x2+x−2 , and i=√−1 then f(i) =
A−2i−4B4i-4C4iD−2i
A,B and C are parallel conductors of equal length carrying currents I,I and 2I respectively. Distance between A and B is x. Distance between B and C is also x. F1 is the force exerted by B on A and F2 is the force exerted by B on C choose the correct answer

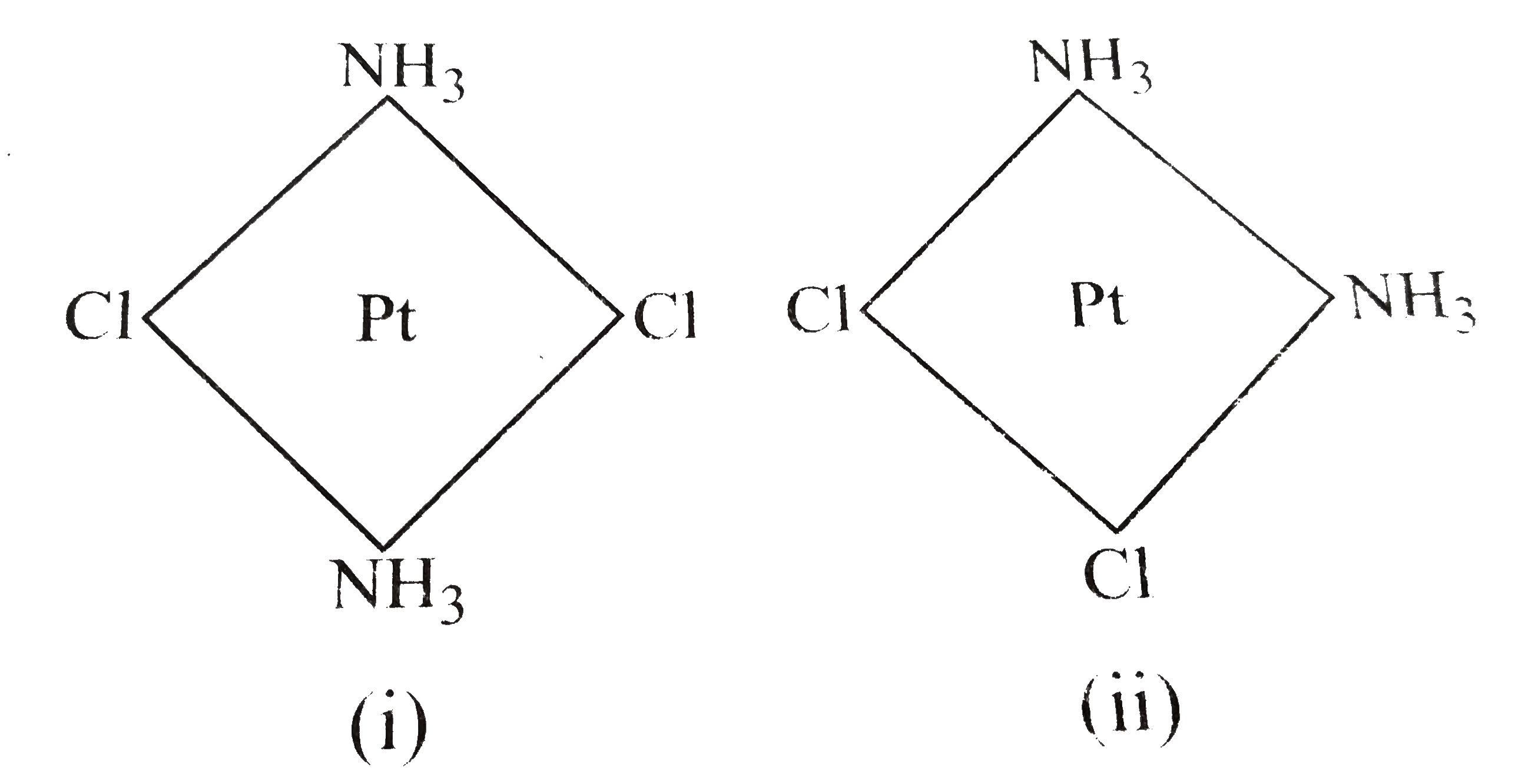
View SolutionThe Pt−CI distance is 2.32A in several crystaline compounds
What is the CI−CI distance in structure (i) and in structure (ii)
 .
.
View SolutionLet f(x)=x3−3x+b and g(x)=x2+bx−3 where b is a real number. What is the sum of all possible values of b for which the equations f(x)= 0 and g(x) = 0 have a common root ?
View SolutionGive the decreasing order or reactivity for the elimination with strong bases of RX(X=F,CI,Br,and I).
b. How is this information important to decide between E2 and E1cB?
View SolutionIf f(x)={A+Bx2,x<13Ax−B+2,x≥1, then A and B, so that f(x) is differentiabl at x = 1, are
View Solution2x(3x+5)+3(3x+5)=ax2+bx+c
In the equation above, a, b, and c are constants. If the equation is true for all values of x, what is the value of b ?
View SolutionIf ax−c=bx+d, what is x in terms of a, b, c, and d?
View Solution
