In the decomposition of at a given temperature T, the energy of activation decreases from to by the use of a catalyst. How many times does the rate of the catalysed reaction increase?
The correct Answer is:D
To solve the problem of how many times the rate of the catalyzed reaction increases when the activation energy decreases from E1 to E2, we can use the Arrhenius equation, which relates the rate constant k of a reaction to the activation energy Ea and temperature T:
k=Ae−EaRT
Where:
- k is the rate constant,
- A is the pre-exponential factor (a constant),
- Ea is the activation energy,
- R is the universal gas constant,
- T is the temperature in Kelvin.
Step 1: Write the rate constants for both reactions
For the uncatalyzed reaction (with activation energy E1):
k1=Ae−E1RT
For the catalyzed reaction (with activation energy E2):
k2=Ae−E2RT
Step 2: Find the ratio of the rate constants
To determine how many times the rate of the reaction increases, we need to find the ratio of k2 to k1:
k2k1=Ae−E2RTAe−E1RT
Step 3: Simplify the expression
Since A cancels out, we have:
k2k1=e−E2RT⋅eE1RT=e−E2−E1RT
Step 4: Interpret the result
The increase in the rate of the reaction due to the catalyst can be expressed as:
k2k1=e−E1−E2RT
This means that the rate of the catalyzed reaction k2 is e−E1−E2RT times the rate of the uncatalyzed reaction k1.
Final Answer
The rate of the catalyzed reaction increases by a factor of e−E1−E2RT.
- k is the rate constant,
- A is the pre-exponential factor (a constant),
- Ea is the activation energy,
- R is the universal gas constant,
- T is the temperature in Kelvin.
For the uncatalyzed reaction (with activation energy E1):
k1=Ae−E1RT
k2=Ae−E2RT
To determine how many times the rate of the reaction increases, we need to find the ratio of k2 to k1:
k2k1=Ae−E2RTAe−E1RT
Since A cancels out, we have:
k2k1=e−E2RT⋅eE1RT=e−E2−E1RT
The increase in the rate of the reaction due to the catalyst can be expressed as:
k2k1=e−E1−E2RT
This means that the rate of the catalyzed reaction k2 is e−E1−E2RT times the rate of the uncatalyzed reaction k1.
The rate of the catalyzed reaction increases by a factor of e−E1−E2RT.
Topper's Solved these Questions
Similar Questions
In the decomposition of H2O2 at 300 K , the energy of activation was found to be 18 kcal/mole , while it decreased to 6 kcal/mole when the decomposition was carried out in the presence of a catalyst at 300 K . How many times is the catalysed reaction faster than the uncatalysed one ?
View SolutionIn the decomosition of H2O2 at 300K, the energy of activation was found to be 18kcal/mole while it decreased to 6 kcal/mole when the decomposition was carried out in the presence of a catalyst at 300K. How many times is the catalysed reaction faster than the uncatalysed one ?
View Solution
Knowledge Check
- Question 1 - Select One
Consider the following reactions at 300 K.
A → B (uncatalysed reaction)
Acatalyst−−−−→B (catalyst reaction)
The activation energy is lowered by 8.314KJ mol−1 for the catalysed reaction. How many times the rate of this catalysed reaction greater than that of uncatalysed reaction?(Given e3.33=20)A15 timesB38 timesC22 timesD28 times - Question 2 - Select One
Arrhenius studied the effect of temperature on the rate of a reaction and postulated that rate constant varies with temperture exponentially as K=A.e−Ea/RT. This method is generally used for finding the activation energy of reaction . Keeping temperature constant , effect of catalyst on activation energy has also been studied.
If on addition of catalyst to a reaction at 300 K, rate of reaction increase by e20 , then difference of activation energy in a absence in absence of catalyst and in presence of catalyst is -A15 kcal/moleB18 kcal/moleC12 kcal/moleD24 kcal/ mole - Question 3 - Select One
By 10 K increase in temperature, the rate of reaction becomes double. How many times the rate of reaction will be if the temperature is increased from 303 K to 353 K ?
A32B6C8D4
The activation energy of a reaction is 75.2 kJ mol−1 in the absence of a catalyst and 50.14 kJ mol−1 in the presence of a catalyst. How many times will the reaction grow in the presence of catalyst if the reaction proceeds at 25∘C ?
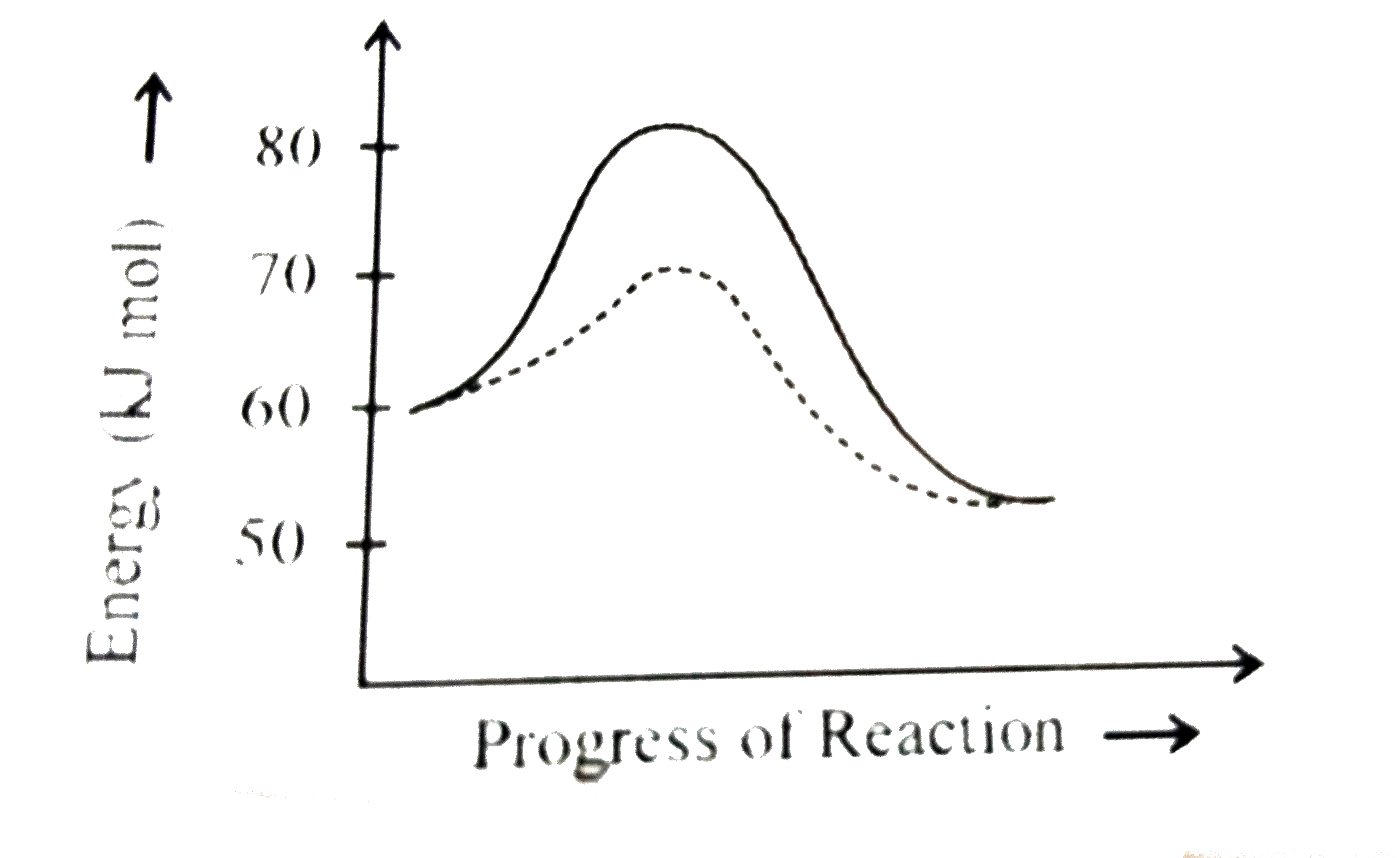
View SolutionFor a reaction A2+B2⇔2AB the figure shows the path of the reaction in absence and presence of a catalyst what will be the energy of activation for forward (Ef) and backward (Eb) reaction in presence of a catalyst and ΔH for the reaction gt the dotted curve is the path of reaction in presence of a catalyst .
 View Solution
View SolutionHow is activation energy of a reaction affected i) by using a catalyst ii) by increasing the temperature?
View SolutionAssertion: A catalyst increases the rate of a reaction.
Reason: In presence of a catalyst, the activation energy of the reaction decreases.View SolutionAssertion: A catalyst increases the rate of a reaction.
Reason: In presence of a catalyst, the activation energy of the reaction decreases.View Solution

