Doubtnut Promotions Sticky

Match the columns with the atomicity of gases to the heat capacity at constant pressure (Cp).
Match the columns with the atomicity of gases to the heat capacity at constant pressure (C(p)).

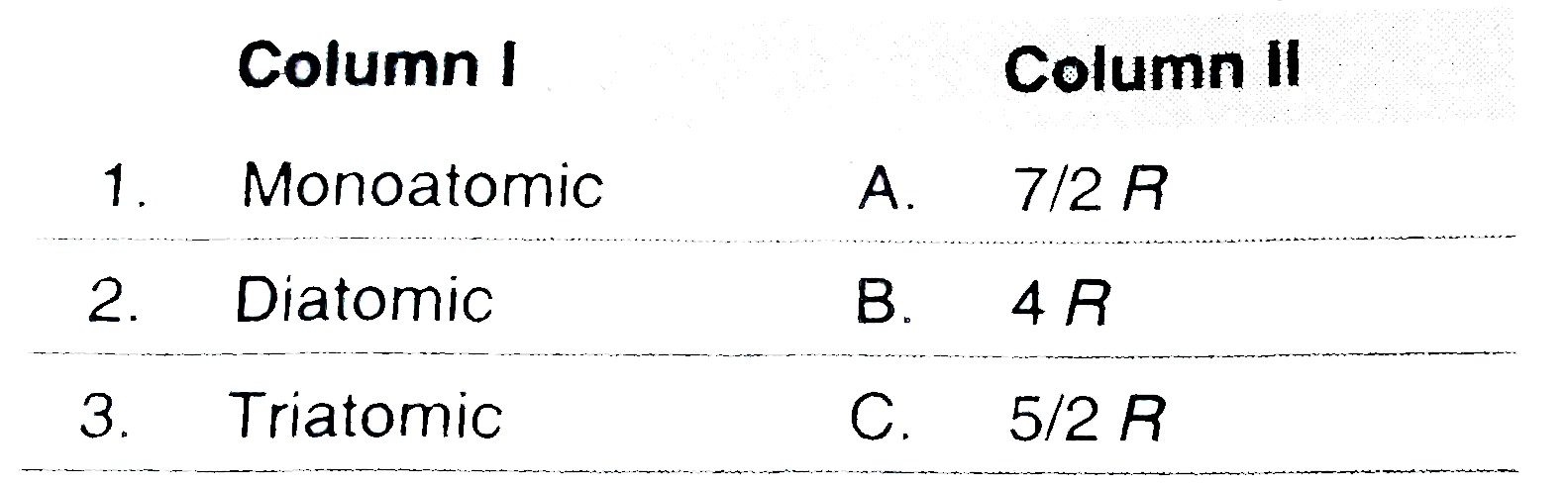
A

1-B,2-C,3-A

B

1-A,2-B,3-C

C

1-C,2-A,3-B

D

1-C,2-B,3-A

Text Solution
Verified by Experts

The correct Answer is:C

1-C, 2-A, 3-B.
For monoatomic gas, Cp=52R
for diatomic gas, Cp=72R
For triatomic gas, Cp=4R
as Cp−CV=R

Doubtnut Promotions Banner
|
Updated on:21/07/2023
Class 12CHEMISTRYTHERMODYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    For an ideal monoatomic gas, the universal gas constant R is n times the molar heat capacity a constant pressure Cp.Here n is

    A0.67
    B1.4
    C0.4
    D1.67
  • Question 2 - Select One

    Specific heat at constant pressure CP of a gas

    Amore than the specific heat at constant volume (CV)
    Bless then the specific heat at constant volume (CV)
    Cequal to the specific heat at constant volume (CV)
    Dmay be more or less than specific heat at constant volume (CV)
  • Question 3 - Select One

    Molar heat capacity at constant P for a substance is equal to

    A(∂U/∂T)v
    B(∂H/∂T)v
    C(∂U/∂T)p
    D(∂H/∂T)p