Molar conductivity of NH4OH can be calculated by the equation.
Λ∘NH4OH=Λ∘Ba(OH)2+Λ∘NH4Cl−Λ∘BaCl2
Λ∘NH4OH=Λ∘BaCl2+Λ∘NH4Cl−Λ∘Ba(OH)2
Λ∘NH4OH=Λ∘Ba(OH)2+2Λ∘NH4Cl−Λ∘BaCl22
Λ∘NH4OH=Λ∘NH4Cl+Λ∘Ba(OH)22
The correct Answer is:C
To calculate the molar conductivity of NH4OH (ammonium hydroxide), we need to use the concept of limiting molar conductivity and the principles of Kohlrausch's law. Here’s a step-by-step solution:
Step 1: Understand Limiting Molar Conductivity
Limiting molar conductivity (Λ0) of an electrolyte is defined as the conductivity of a solution divided by the molarity of the solution as the concentration approaches zero. For weak electrolytes like NH4OH, we can express its limiting molar conductivity in terms of its constituent ions.
Step 2: Identify the Ions in NH4OH
The dissociation of NH4OH in water can be represented as:
NH4OH⇌NH+4+OH−
Thus, the limiting molar conductivity of NH4OH can be expressed as:
Λ0NH4OH=Λ0NH+4+Λ0OH−
Step 3: Use Kohlrausch's Law
According to Kohlrausch's law, the limiting molar conductivity of a weak electrolyte can also be expressed in terms of the limiting molar conductivities of other related electrolytes. We will use the conductivities of Ba(OH)2, NH4Cl, and BaCl2 to derive the expression for NH4OH.
1. For Ba(OH)2:
Ba(OH)2→Ba2++2OH−
Thus,
Λ0Ba(OH)2=Λ0Ba2++2Λ0OH−
2. For NH4Cl:
NH4Cl→NH+4+Cl−
Thus,
Λ0NH4Cl=Λ0NH+4+Λ0Cl−
3. For BaCl2:
BaCl2→Ba2++2Cl−
Thus,
Λ0BaCl2=Λ0Ba2++2Λ0Cl−
Step 4: Set Up the Equation
To find Λ0NH4OH, we can use the relationships derived from the above equations. We can manipulate the equations to express Λ0NH4OH in terms of the other conductivities.
Using the equations:
- Λ0Ba(OH)2=Λ0Ba2++2Λ0OH−
- Λ0NH4Cl=Λ0NH+4+Λ0Cl−
- Λ0BaCl2=Λ0Ba2++2Λ0Cl−
We can derive:
Λ0NH4OH=Λ0Ba(OH)2+Λ0NH4Cl−Λ0BaCl2
Step 5: Choose the Correct Option
Based on the derived equation, we can see that the correct option that represents the limiting molar conductivity of NH4OH is:
Λ0NH4OH=Λ0Ba(OH)2+Λ0NH4Cl−Λ0BaCl2
This corresponds to Option 1.
Final Answer
Thus, the molar conductivity of NH4OH can be calculated by the equation:
Λ0NH4OH=Λ0Ba(OH)2+Λ0NH4Cl−Λ0BaCl2
Limiting molar conductivity (Λ0) of an electrolyte is defined as the conductivity of a solution divided by the molarity of the solution as the concentration approaches zero. For weak electrolytes like NH4OH, we can express its limiting molar conductivity in terms of its constituent ions.
The dissociation of NH4OH in water can be represented as:
NH4OH⇌NH+4+OH−
Thus, the limiting molar conductivity of NH4OH can be expressed as:
Λ0NH4OH=Λ0NH+4+Λ0OH−
According to Kohlrausch's law, the limiting molar conductivity of a weak electrolyte can also be expressed in terms of the limiting molar conductivities of other related electrolytes. We will use the conductivities of Ba(OH)2, NH4Cl, and BaCl2 to derive the expression for NH4OH.
Ba(OH)2→Ba2++2OH−
Thus,
Λ0Ba(OH)2=Λ0Ba2++2Λ0OH−
NH4Cl→NH+4+Cl−
Thus,
Λ0NH4Cl=Λ0NH+4+Λ0Cl−
BaCl2→Ba2++2Cl−
Thus,
Λ0BaCl2=Λ0Ba2++2Λ0Cl−
To find Λ0NH4OH, we can use the relationships derived from the above equations. We can manipulate the equations to express Λ0NH4OH in terms of the other conductivities.
- Λ0Ba(OH)2=Λ0Ba2++2Λ0OH−
- Λ0NH4Cl=Λ0NH+4+Λ0Cl−
- Λ0BaCl2=Λ0Ba2++2Λ0Cl−
Λ0NH4OH=Λ0Ba(OH)2+Λ0NH4Cl−Λ0BaCl2
Based on the derived equation, we can see that the correct option that represents the limiting molar conductivity of NH4OH is:
Λ0NH4OH=Λ0Ba(OH)2+Λ0NH4Cl−Λ0BaCl2
This corresponds to Option 1.
Thus, the molar conductivity of NH4OH can be calculated by the equation:
Λ0NH4OH=Λ0Ba(OH)2+Λ0NH4Cl−Λ0BaCl2
Topper's Solved these Questions
- ELECTROCHEMISTRYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROCHEMISTRYEXERCISE - HOTS
10 Videos
- ELECTROCHEMISTRYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROCHEMISTRYEXERCISE - EXEMPLAR PROBLEMS
17 Videos
- COORDINATION COMPOUNDS BOOK - NCERT FINGERTPS ENGLISHCHAPTER - COORDINATION COMPOUNDS EXERCISE - Assertion And Reason
15 Videos
- GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTSEXERCISE - Assertion And Reason
15 Videos
Similar Questions
The molar conductivity of NH4Cl at infinite dilution is 149.7ohm−cm2mol−1 and ionic conductances of hydroxyl ions and chloride ions are 198 and 76.3 respectively. Calculate the molar conductivity of NH4OH at infinite dilution.
View SolutionCalculate molar conductivity of HCOOH at infinite dilution, if equivalent conductivity of H2SO4=x1, Al2(SO4)3=x2, (HCOO)3Al=x3
View Solution(a) Apply Kohlrausch law of independent migration of ions, write the expression to determine the limiting molar conductivity of calcium chloride.
(b) Given are the conductivity and molar conductivity of NaCI solutions at 298 K at different concentrations :
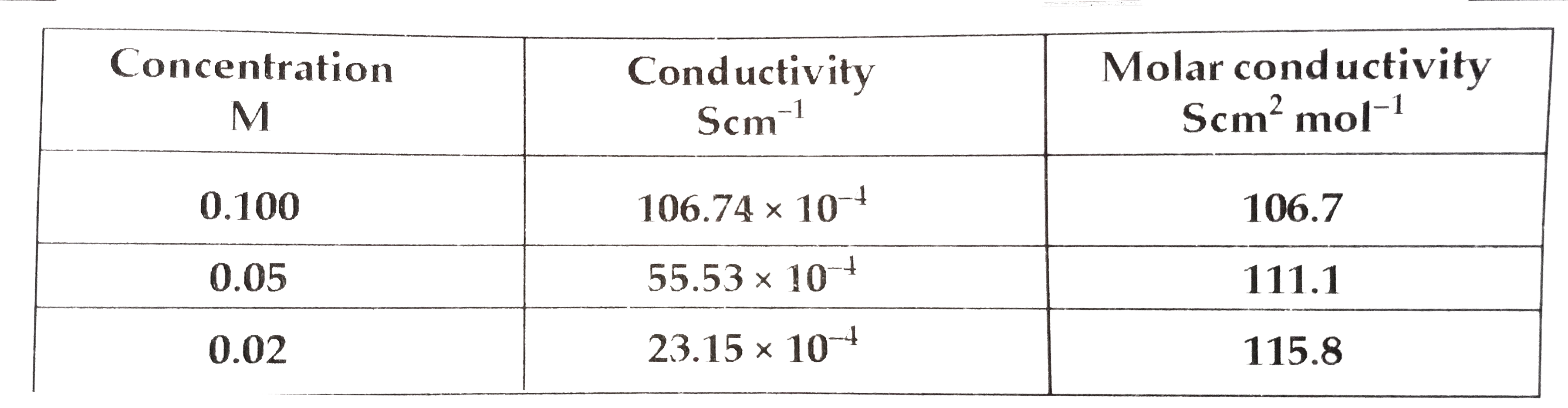
Compare the variation of conductivity and molar conductivity of NaCI solutions on dilution. Give reason.
(c) 0.1 M KCI solution offered a resistance of 100 ohms in conductivity cell at 298 K. If the cell constant of the cell is 1.29cm−1, calculate the molar conductivity of KCI solution.
View SolutionThe heat of neutralization of (i) CHCl2–COOH by NaOH is 12830 cal, (ii) HCl by NaOH is 13680 cal and (iii) NH4OH by HCl is 12270 cal. What is the heat of neutralization of dichloro acetic acid by NH4OH? Calculate the heats of ionization of dichloro acetic acid and NH4OH.
View SolutionCalculate limiting molar conductivity of CaSO4 (limiting molar conductivity of Calcium and sulfate ions are 119.0 and 160.0 Scm2mol−1 respectively.
View Solution(a) Define the terms conductivity and molar conductivity for the solution of an electrolyte.
Comment on their variation with temperature
(b) The measured resistance of a conductance cell was 100 ohms. Calculate (i) the specific conductance and (ii) the molar conductance of the solution
(KCl=74.5gmol−1 and cell constant =1.25 cm−1)
View SolutionLimiting molar conductivity of NH4OH [i.e., Λ∘m(NH4OH)] is equal to:
View SolutionA 0.05 M NH4OH solution offers the resistance of 50 ohm to a conductivity cell at 298 K. If the cell constant is 0.50cm−1 and molar conductance of NH4OH at infinite dilution is 471.4ohm−1cm2mol−1, calculate :
Molar conductance
View SolutionA 0.05 M NH4OH solution offers the resistance of 50 ohm to a conductivity cell at 298 K. If the cell constant is 0.50cm−1 and molar conductance of NH4OH at infinite dilution is 471.4ohm−1cm2mol−1, calculate :
Specific conductance
View SolutionA 0.05 M NH4OH solution offers the resistance of 50 ohm to a conductivity cell at 298 K. If the cell constant is 0.50cm−1 and molar conductance of NH4OH at infinite dilution is 471.4ohm−1cm2mol−1, calculate :
Degree of dissociation
View Solution
