Pure (S)-2-butanol has a specific rotation of +13.52 degrees. A sample of 2-butanol prepared in the lab and purified by disillation has a calculated specific rotation of + 6.76 degrees. What can you conclude about the composition ?
50% (S), 50% impurity
50% (S),50% (R)
50% (S), 50% racemic
some other mixture
The correct Answer is:c
To analyze the composition of the 2-butanol sample based on its specific rotation, we can follow these steps:
Step 1: Understand Specific Rotation
Specific rotation ((α)) is a property of chiral compounds that indicates the degree to which they rotate plane-polarized light. It is expressed in degrees and is dependent on the concentration of the solution and the path length of the light through the sample.
Step 2: Identify the Pure Compound's Specific Rotation
The specific rotation of pure (S)-2-butanol is given as +13.52 degrees. This means that if we have a pure sample of (S)-2-butanol, it will rotate plane-polarized light by +13.52 degrees.
Step 3: Analyze the Sample's Specific Rotation
The sample of 2-butanol prepared in the lab has a specific rotation of +6.76 degrees. This value is less than that of the pure (S)-2-butanol, indicating that the sample is not purely (S)-2-butanol.
Step 4: Determine the Composition
The specific rotation of a mixture can be calculated using the formula:
(α)mix=(α)pure⋅(n)(n+m)
Where:
- (α)_{mix} = specific rotation of the mixture
- (α)_{pure} = specific rotation of the pure enantiomer
- n = moles of (S)-2-butanol
- m = moles of (R)-2-butanol (the enantiomer)
Since the specific rotation of the mixture is +6.76 degrees, which is closer to zero than +13.52 degrees, we can conclude that the sample contains a mixture of both (S)-2-butanol and (R)-2-butanol.
Step 5: Calculate the Ratio of Enantiomers
To find the ratio of (S)-2-butanol to (R)-2-butanol, we can use the following relationship:
Specific rotation of mixture=(n⋅+13.52)+(m⋅−13.52)n+m
Given that the specific rotation is +6.76 degrees, we can set up the equation and solve for the ratio of n to m.
Conclusion
The calculated specific rotation of +6.76 degrees indicates that the sample is a mixture of (S)-2-butanol and (R)-2-butanol. The presence of the (R)-enantiomer reduces the overall specific rotation compared to that of pure (S)-2-butanol.
Specific rotation ((α)) is a property of chiral compounds that indicates the degree to which they rotate plane-polarized light. It is expressed in degrees and is dependent on the concentration of the solution and the path length of the light through the sample.
The specific rotation of pure (S)-2-butanol is given as +13.52 degrees. This means that if we have a pure sample of (S)-2-butanol, it will rotate plane-polarized light by +13.52 degrees.
The sample of 2-butanol prepared in the lab has a specific rotation of +6.76 degrees. This value is less than that of the pure (S)-2-butanol, indicating that the sample is not purely (S)-2-butanol.
The specific rotation of a mixture can be calculated using the formula:
(α)mix=(α)pure⋅(n)(n+m)
Where:
- (α)_{mix} = specific rotation of the mixture
- (α)_{pure} = specific rotation of the pure enantiomer
- n = moles of (S)-2-butanol
- m = moles of (R)-2-butanol (the enantiomer)
To find the ratio of (S)-2-butanol to (R)-2-butanol, we can use the following relationship:
Specific rotation of mixture=(n⋅+13.52)+(m⋅−13.52)n+m
Given that the specific rotation is +6.76 degrees, we can set up the equation and solve for the ratio of n to m.
The calculated specific rotation of +6.76 degrees indicates that the sample is a mixture of (S)-2-butanol and (R)-2-butanol. The presence of the (R)-enantiomer reduces the overall specific rotation compared to that of pure (S)-2-butanol.
Topper's Solved these Questions
- BOOK - MS CHOUHANCHAPTER - ISOMERISM (STRUCTURAL & STEREOISOMERISM)EXERCISE - Level 1 (Q.1 To Q.30)13videos
ISOMERISM (STRUCTURAL & STEREOISOMERISM)
- BOOK - MS CHOUHANCHAPTER - ISOMERISM (STRUCTURAL & STEREOISOMERISM)EXERCISE - Level 1 (Q.31 To Q.60)9videos
ISOMERISM (STRUCTURAL & STEREOISOMERISM)
- BOOK - MS CHOUHANCHAPTER - GRIGNARD REAGENTSEXERCISE - Level 1 (Q.61 To Q.66)1videos
GRIGNARD REAGENTS
- BOOK - MS CHOUHANCHAPTER - NOMENCLATURE AND CONFORMATIONS OF ALKANES AND CYCLOALKANESEXERCISE - Additional Objective Questions (MCQ)1videos
NOMENCLATURE AND CONFORMATIONS OF ALKANES AND CYCLOALKANES
Similar Questions
Pure cholesterol has a specific rotation of -32. A sample of cholesterol prepared in the lab has a specific rotation of -8. The enatiomeric excess of the sample of chloresterol is x%. X is :
View Solution(R)-2-methyl-1-butanol has a specific rotation of +13.5∘. The specific rotation of 2-methyl-1-butanol containing 40% of the (S)-enantiomer is _____
View Solution
Knowledge Check
- Question 1 - Select One
Dextrororatory bytan−2−ol has a specific rotation [α]25D=+13.52∘. A sample of butan−2−ol shows a specific rotation [α]25D=+6.72∘. Calculate the enantiomers excess (or optical purity) of the sample?
A60%B50%C70%D80% - Question 2 - Select One
(+) - 2 -butanol has [θ]25λ=+13.9∘. A sample of 2-butanol containing both the enantiomers was found to have a specific rotation value of −3.5∘ under similar condition. The percentage of the (+) and (-) enantiomer present in the sample are, respectively:
A37.4%and62.6%B62.6%and37.4%C42.2%and57.8%D35.5%and64.5% - Question 3 - Select One
If solution of a compound (30g/100ml of solution ) has measured rotation of +15∘ in a 2dm long sample tube, the specific rotation of this compound is
A+50∘B+7.5∘C+15∘D+25∘
The observed rotation of 2 gram of a compound in 10ml of solution in polarimeter tube of 25cm long is +24.6∘. The specific rotation of the compound (in degree) is
View SolutionCholesterol, when isolated from natural sources, is obtained as a single enantiomer. The observed rotation α of a 0.3 g sample of cholesterol in 5mL of chloroform solution contained in a 10 cm polarimeter tube is −0.78∘. Calculate the specific rotation of cholesterol. A sample of synthetic cholesterol was prepared consisting entirely of (+)-cholesterol. This synthetic (+)-cholesterol was mixed with some natural (-)-cholesterol. The mixture had a specific rotation [α]20D of −13∘. What fraction of the mixture was (+)-cholesterol ?
View SolutionD(+) Glucose has melting point 146∘C and specific rotation [α]C6(25) is +122∘C. Another D(+) Glucose has melting 150∘C and specific rotation [α]25D is +18.7∘C. The two form have significantly different optical rotation but when an aqueous solution of either form is allowed to stand, it rotation changes. The specific rotation of one form decrease and rotation of other increases until both solution show the same value +52.7∘. The change in rotation towards an equilibrium value is called mutarotation.
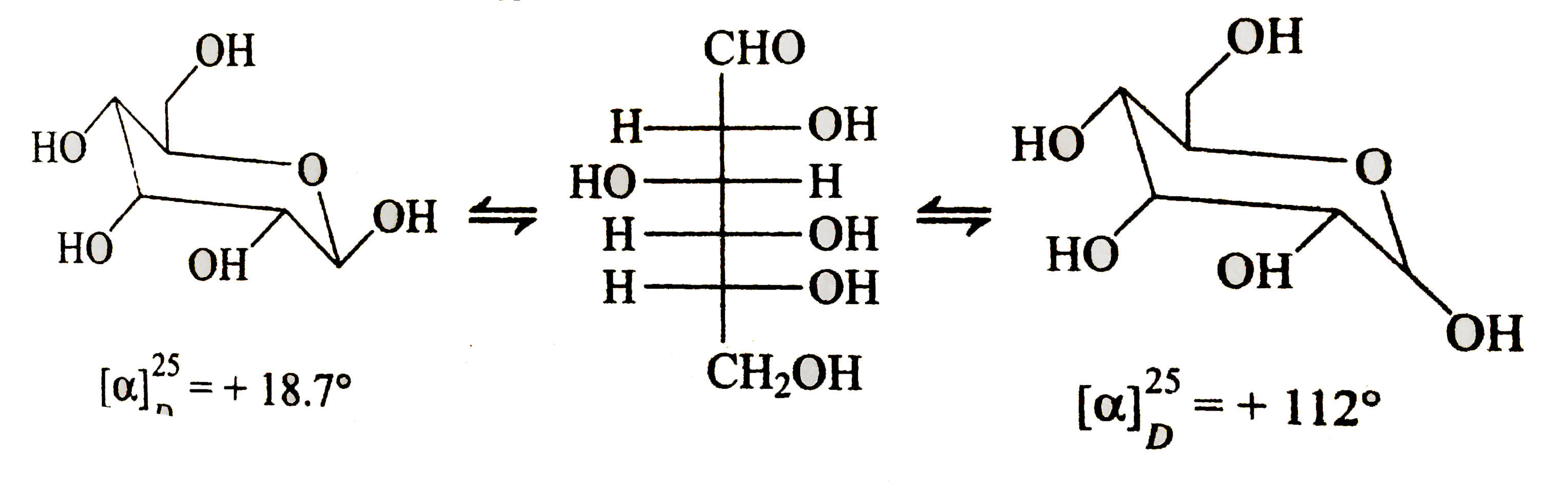
For mannose the mutarotation can be shown in brief as follow:
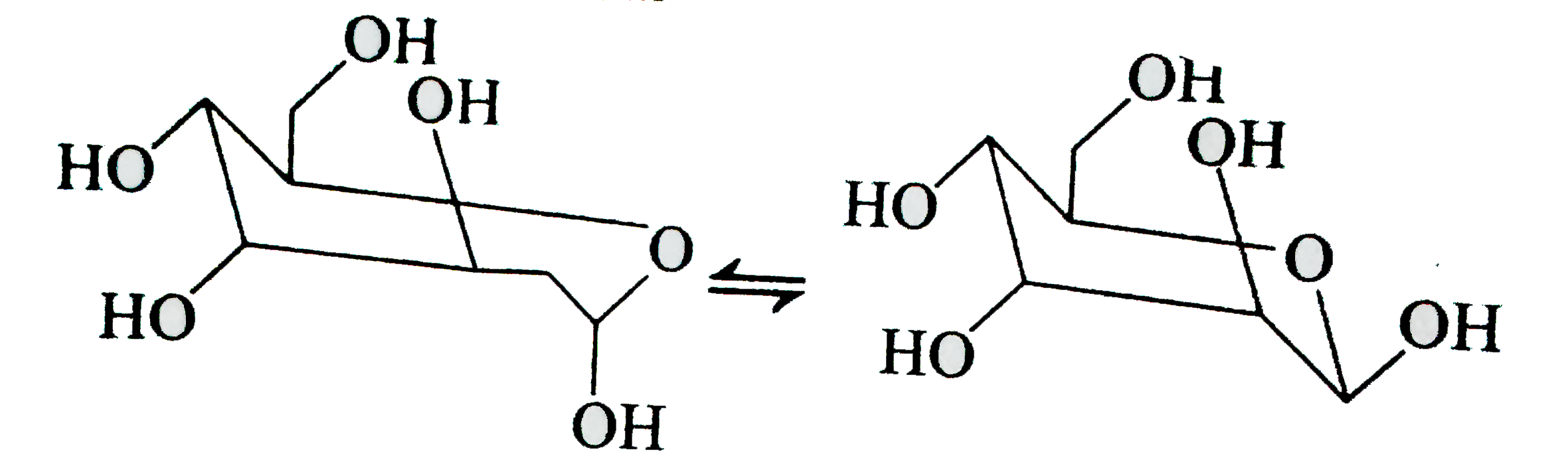 View Solution
View SolutionD(+) Glucose has melting point 146∘C and specific rotation [α]C6(25) is +122∘C. Another D(+) Glucose has melting 150∘C and specific rotation [α]25D is +18.7∘C. The two form have significantly different optical rotation but when an aqueous solution of either form is allowed to stand, it rotation changes. The specific rotation of one form decrease and rotation of other increases until both solution show the same value +52.7∘. The change in rotation towards an equilibrium value is called mutarotation.

What percentage of β-D-(+) glucopyrnsone found at equillibrium in the aqueous solution?View Solution(+)−Butan−2−ol has [α]20D=+13.9∘.a sample of butane2−ol contianing both the enantiomers was found to have a specific rotation value of −3.5∘ under similar conditions. The percentages of the (+) and (−) enantiomers present in the mixture are, respectively,
View Solution

