Sliver metal crystallizes in a cubic closet packed arrangement with the edge of the unit cell having a length . The distance between centers of two closet atoms is
A
pm
B
pm
C
pm
D
pm
Video Solution
Text Solution
The correct Answer is:1
Cubic closest packing uses a face centered cubic unit cell.Looking at any one face of the cube, we notice that the face atoms touch the corner atoms along the diagonal of the face but that corner atoms do not touch one another along the edges
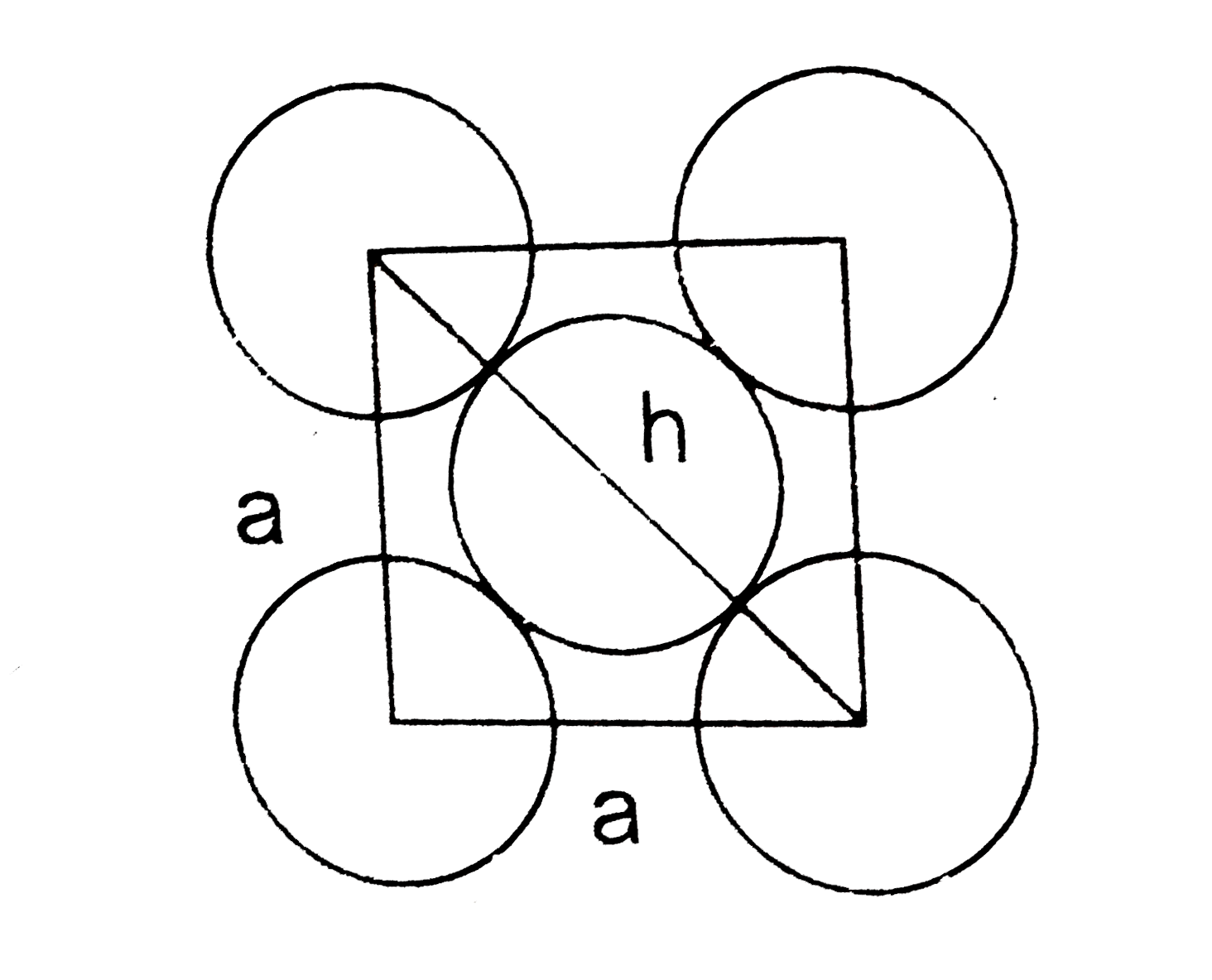
therefore the two atoms closest to each other are those along the face diagonal. One face of the face centered unit cell is shown above. with the atoms touching ,the nearnest neighbour atoms are the ones along the diagonal of the face of the cube , We may visualize the face as consisting of two righ isoscales triangle sharing a common hypotenuse , and having sides of lengths pm. the hypotenuse is equal to twice the centre to centre distance.The hypotaneous can be calculated from the pythagorean theorem .The length of the hypotaneous equals the square root of the sum of the squares of the sides
pm
|
Topper's Solved these Questions
- SOLID STATEBOOK - R SHARMACHAPTER - SOLID STATEEXERCISE - Follow Test 8
13 Videos
- SOLID STATEBOOK - R SHARMACHAPTER - SOLID STATEEXERCISE - Follow Test 9
7 Videos
- SOLID STATEBOOK - R SHARMACHAPTER - SOLID STATEEXERCISE - Follow Test 6
4 Videos
- POLYMERSBOOK - R SHARMACHAPTER - POLYMERSEXERCISE - All Questions
65 Videos
- SOLUTIONSBOOK - R SHARMACHAPTER - SOLUTIONSEXERCISE - ARCHIVES
42 Videos
Similar Questions
Knowledge Check
Silver metal crystallises in a cubic closed packed arrangement with edge length 404 pm .Thus radius of the silver atom is
A203.5 pmB138.8 pmC142.8 pmD407.0 pmA metal crystallize in a body centered cubic lattice (bcc) with the edge of the unit cell 5.2Å. The distance between the two nearest neighour is
A10.4ÅB4.5ÅC5.2ÅD9.0Åa metal crystallizes with a face-centered cubic lattice.The edge of the unit cell is 408 pm. The diameter of the metal atom is :
A288 pmB408 pmC144 pmD204 pm
a metal crystallizes with a face-centered cubic lattice.The edge of the unit cell is 408 pm. The diameter of the metal atom is :
View SolutionA metal crystallizes with a face-centered cubic lattice. The edge of the unit cell is 408 pm. The diameter of the metal atom is
View SolutionThe edge length of face centred cubic unit cell is 508 pm. The nearest distance between two atoms is :
View Solutiona metal crystallizes with a face-centered cubic lattice.The edge of the unit cell is 408 pm. The diameter of the metal atom is :
View SolutionA metal crystallizes with a face -centred cubic lattice.The edge of the unit cells is 408 pm.The dimeter of the metal atoms is
View Solution
