The correct starting material and product of different disintegration series is/are
Th232,Pb208
Np237,Bi209
U235,Pb206
U238,Pb206
The correct Answer is:A, D
To solve the question regarding the correct starting material and product of different disintegration series, we will analyze the various radioactive decay series and their end products.
1. Identify the Disintegration Series:
- There are several well-known disintegration series, including the Thorium series, Neptunium series, Uranium series, and Actinium series.
2. Thorium Series (4n):
- Starting Material: Thorium-232 (Th-232)
- End Product: Lead-208 (Pb-208)
- This series follows the decay of thorium through a series of alpha and beta decays until it stabilizes at lead.
3. Neptunium Series (4n + 1):
- Starting Material: Neptunium-237 (Np-237)
- End Product: Bismuth-209 (Bi-209)
- This series involves the decay of neptunium through several steps until it reaches bismuth.
4. Uranium Series (4n + 2):
- Starting Material: Uranium-238 (U-238)
- End Product: Lead-206 (Pb-206)
- The uranium series decays through multiple isotopes until it produces lead.
5. Actinium Series (4n + 3):
- Starting Material: Actinium-227 (Ac-227)
- End Product: Lead-207 (Pb-207)
- This series also involves a series of decays leading to the stable isotope of lead.
Summary of Results:
- Thorium Series: Th-232 → Pb-208
- Neptunium Series: Np-237 → Bi-209
- Uranium Series: U-238 → Pb-206
- Actinium Series: Ac-227 → Pb-207
Final Answer:
The correct starting material and product of different disintegration series are:
- Thorium Series: Thorium (Th) to Lead (Pb)
- Neptunium Series: Neptunium (Np) to Bismuth (Bi)
- There are several well-known disintegration series, including the Thorium series, Neptunium series, Uranium series, and Actinium series.
- Starting Material: Thorium-232 (Th-232)
- End Product: Lead-208 (Pb-208)
- This series follows the decay of thorium through a series of alpha and beta decays until it stabilizes at lead.
- Starting Material: Neptunium-237 (Np-237)
- End Product: Bismuth-209 (Bi-209)
- This series involves the decay of neptunium through several steps until it reaches bismuth.
- Starting Material: Uranium-238 (U-238)
- End Product: Lead-206 (Pb-206)
- The uranium series decays through multiple isotopes until it produces lead.
- Starting Material: Actinium-227 (Ac-227)
- End Product: Lead-207 (Pb-207)
- This series also involves a series of decays leading to the stable isotope of lead.
- Thorium Series: Th-232 → Pb-208
- Neptunium Series: Np-237 → Bi-209
- Uranium Series: U-238 → Pb-206
- Actinium Series: Ac-227 → Pb-207
The correct starting material and product of different disintegration series are:
- Thorium Series: Thorium (Th) to Lead (Pb)
- Neptunium Series: Neptunium (Np) to Bismuth (Bi)
Topper's Solved these Questions
- NUCLEAR CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Exercises Single Correct
80 Videos
- NUCLEAR CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Exercises Assertion-Reasoning
14 Videos
- NUCLEAR CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Exercises Link Comprehension
30 Videos
- NCERT BASED EXERCISEBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NCERT BASED EXERCISEEXERCISE - Nuclear Chemistry (NCERT Exercise)
29 Videos
- ORGANIC COMPOUNDS WITH FUNCTIONAL GROUPBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ORGANIC COMPOUNDS WITH FUNCTIONAL GROUPEXERCISE - Archives Analytical And Descriptive
24 Videos
Similar Questions
The end product of (4n+2) disintegration series is
View SolutionThe starting material of PCTFEis:
View SolutionThe end product of 4n series is
View Solution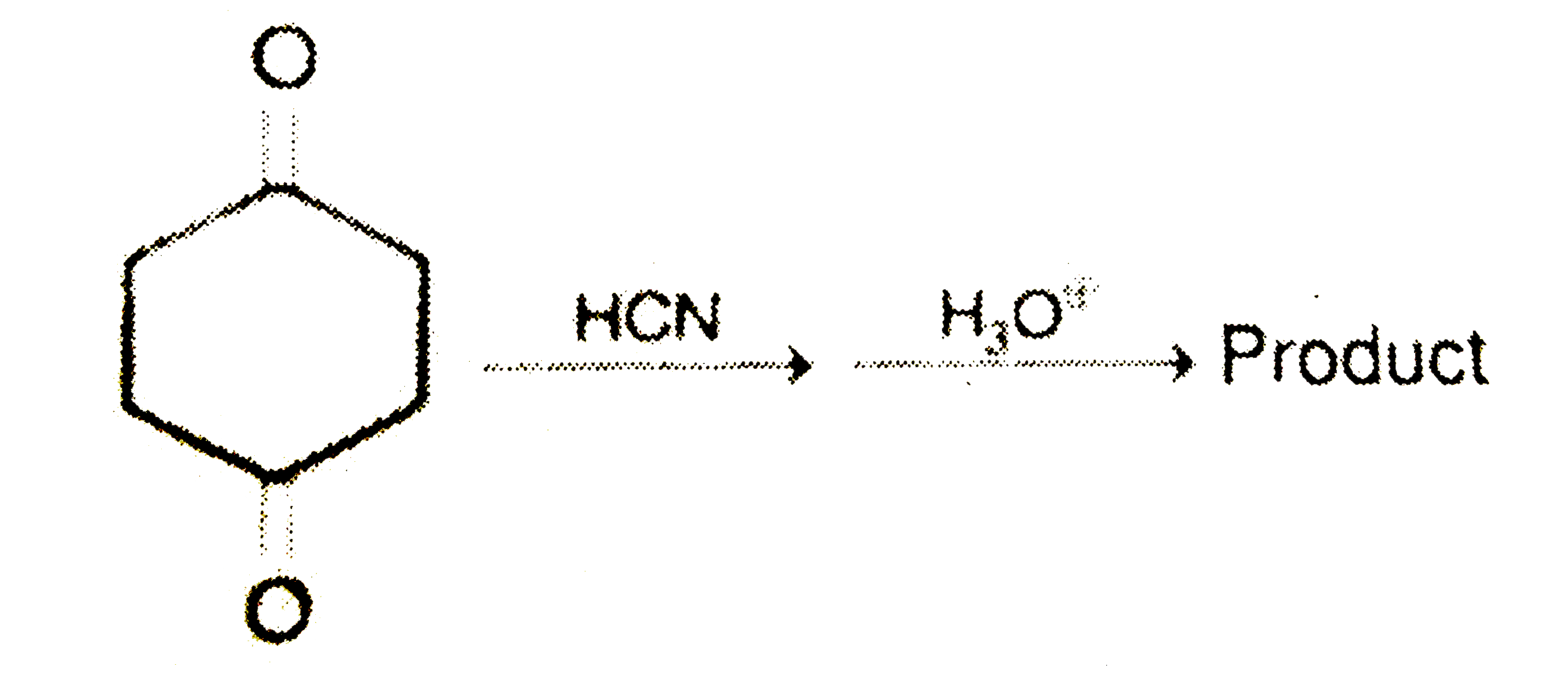 Product
Product
The correct statements about products is/are
View SolutionRate limiting material in biogas products is
View SolutionThe correct sequence in biogas production is
View SolutionThe starting material used in Solvay's process are
View Solution
The correct name of the product obtained is
View SolutionWhat is the end product of natural radioactive series?
View SolutionThe decay constant of the end product of a radioactive series is
View Solution