The energy released on combustion of 1 mole of C(s) with gaseous oxygen is
ΔH∘combustion
ΔH∘formation of CO2
Both are true
None is true
The correct Answer is:B
To determine the energy released on the combustion of 1 mole of solid carbon (C) with gaseous oxygen (O₂), we can follow these steps:
Step 1: Write the balanced chemical equation for the combustion of carbon.
The combustion of solid carbon (C) in the presence of oxygen (O₂) produces carbon dioxide (CO₂). The balanced equation for this reaction is:
C(s)+O2(g)→CO2(g)
Step 2: Identify the enthalpy change associated with the reaction.
The enthalpy change for the combustion of carbon is represented as ΔH_combustion. This value indicates the amount of energy released when one mole of carbon is completely combusted.
Step 3: Look up the standard enthalpy of formation for CO₂.
The standard enthalpy of formation (ΔH_f) for CO₂ is a known value. For CO₂, it is approximately:
ΔHf(CO2)=−393.5kJ/mol
This value indicates that when 1 mole of CO₂ is formed from its elements (carbon and oxygen), 393.5 kJ of energy is released.
Step 4: Relate the enthalpy of combustion to the enthalpy of formation.
The enthalpy of combustion of carbon can be expressed as the negative of the enthalpy of formation of CO₂:
ΔHcombustion=−ΔHf(CO2)
Step 5: Calculate the energy released.
Substituting the value from Step 3 into the equation gives:
ΔHcombustion=−(−393.5kJ/mol)=393.5kJ/mol
Thus, the energy released on the combustion of 1 mole of solid carbon with gaseous oxygen is approximately 393.5 kJ.
Final Answer:
The energy released on combustion of 1 mole of C(s) with gaseous oxygen is 393.5 kJ.
---
The combustion of solid carbon (C) in the presence of oxygen (O₂) produces carbon dioxide (CO₂). The balanced equation for this reaction is:
The enthalpy change for the combustion of carbon is represented as ΔH_combustion. This value indicates the amount of energy released when one mole of carbon is completely combusted.
The standard enthalpy of formation (ΔH_f) for CO₂ is a known value. For CO₂, it is approximately:
The enthalpy of combustion of carbon can be expressed as the negative of the enthalpy of formation of CO₂:
Substituting the value from Step 3 into the equation gives:
The energy released on combustion of 1 mole of C(s) with gaseous oxygen is 393.5 kJ.
Topper's Solved these Questions
- THERMODYNAMICS AND THERMOCHEMISTRY BOOK - ERRORLESS CHAPTER - THERMODYNAMICS AND THERMOCHEMISTRY EXERCISE - ORDINARY THINKING (Objective Questions) Bond energy
15 Videos
- THERMODYNAMICS AND THERMOCHEMISTRY BOOK - ERRORLESS CHAPTER - THERMODYNAMICS AND THERMOCHEMISTRY EXERCISE - ORDINARY THINKING (Objective Questions) Free energy and Work function
57 Videos
- THERMODYNAMICS AND THERMOCHEMISTRY BOOK - ERRORLESS CHAPTER - THERMODYNAMICS AND THERMOCHEMISTRY EXERCISE - ORDINARY THINKING (Objective Questions) First law of thermodynamics and Hess law
100 Videos
- SOME BASIC CONCEPTS OF CHEMISTRYBOOK - ERRORLESS CHAPTER - SOME BASIC CONCEPTS OF CHEMISTRYEXERCISE - JEE Section (Matrix Match type questions)
3 Videos
Similar Questions
Knowledge Check
The amount of energy released during complete combustion of 1 gm of substances is its
Aspecific valueBreaction energyCphysiological valueDcalorific fuel valueThe CORRECT decreasing order of energy released when an electron is added to neutral gaseous halogens is _________.
AF>ClBr>IBCl>Br>F>ICF>Br>Cl>IDCl>FBr>IHow much usable energy is available during oxidative combustion of 1 gm mole of glucose in the body ?
A686000 calB304000 calC277400 calD686 cal.
How much usable energy is available during oxidative combustion of 1 gm mole of glucose in the body?
View SolutionThe energy released when an electron is added to a neutral gaseous atom would be highest if the element belongs to
View SolutionCombustion of most fuels releases:
View SolutionIn the complete combustion of hydrocarbon (CnH2n+2) the number of oxygen molecules required per mole of hydrocarbon is
View SolutionThe enthalpy of gas phase trimerzation of one mole of gaseous formaldehyde in (KJ/mole)
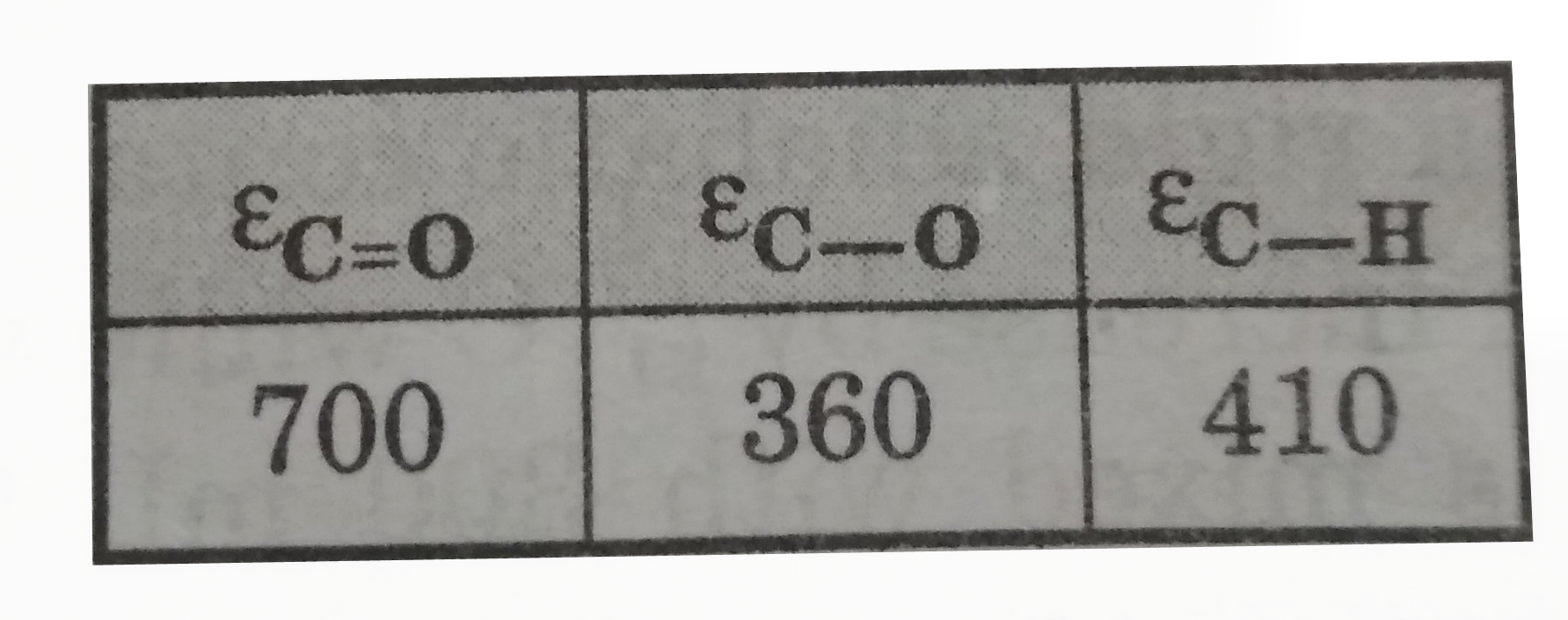
Bond energies (kj/mole)
Given:
εc=oεc−oεc−H700360410
View Solution
