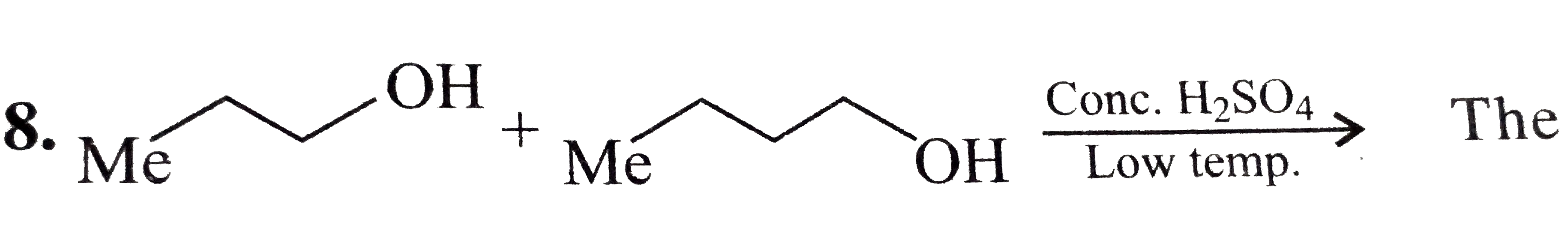
 The propduct (s) are:
The propduct (s) are:
Butyl propyl ether
Dipropyl ether
Dibutyl ether
Propene + But -2-ene (cis and trans) + Butene
The correct Answer is:A, B, C
A non-symmetrical ether is not usually prepared by heating a mixture of R'OH in acid. A mixture of three ethers R−−−O−−−R,R−−−−O−−−R' , and R'−−−O−−−R' is obtained. So the products are: Pr−OH+n−BuOH→PrBu(a)+PrOPr(b)+BuOBu(c)
Topper's Solved these Questions
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ALCOHOL,PHENOL AND ETHERSEXERCISE - Exercises Single Correct72videos
ALCOHOL,PHENOL AND ETHERS
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ALCOHOL,PHENOL AND ETHERSEXERCISE - Exercises Assertion-Reasoning5videos
ALCOHOL,PHENOL AND ETHERS
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ALCOHOL,PHENOL AND ETHERSEXERCISE - Exercises Linked Comprehension60videos
ALCOHOL,PHENOL AND ETHERS
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ALIPHATIC AND AROMATIC ALDEHYDES AND KETONESEXERCISE - Archives Subjective26videos
ALIPHATIC AND AROMATIC ALDEHYDES AND KETONES
Similar Questions
Compound (S) that on hydrogenation product (S) optically inactive compound (s) is/are
View SolutionThe nitrogen oxide (s) that contain (s) N−N bonds (s) is (are).
View SolutionIf c2=a2+b2, then prove that 4s(s−a)(s−b)(s−c)=a2b2
View SolutionFor the reaction,Fe(s)+S(s)→FeS(s) the expression for equilibrium constant is
View SolutionIf S=a+b+c then prove that SS−a+SS−b+SS−c>92 where a,b & c are distinct positive reals.
View SolutionS8 ring of both rhombic sulphur and monoclinic sulphur has crown shaped structure . The S- S -S bond angle in S8 ring is
View SolutionAssertion (A) :Angular momentum of 1s,2s,3s, ets all have spectrical shape
Reason (R ) : 1s,2s,3s, ets all have spherical shapeView SolutionSO2−3+S⋆boil−−→SS⋆O2−3,SS⋆O2−3+2H+→H2SO3+S⋆
The above reaction sequence proves:View SolutionIf s1,s2,s3 denote the sum of n terms of 3 arithmetic series whose first terms are unity and their common difference are in H.P., Prove that n=2s3s1−s1s2−s2s3s1−2s2+s3
View SolutionIf S1,S2,S3 be respectively the sums of n,2n,3n terms of a G.P., then prove that (S1)2+(S2)2=S1(S2+S3) .
View Solution
