The reaction between A and B is first order with respect to A and zero order with respect to B. Fill in the blanks in the following table:
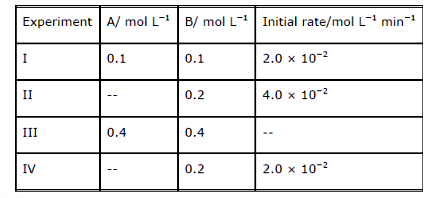
The given reaction is of the first order with respect to A and of zero order with respect to B .
Therefore , the rate of the reaction is given by ,
Rate = k[A]1[B]0
⇒ Rate = k [A]
From experiment l , we obtain
2.0×10−2molL−1min−1=k(0.1molL−1)
⇒k=0.2min−1
From experiment ll , we obtain
4.0×10−2molL−1min−1=0.2min−1[A]
⇒[A]=0.2molL−1
From experiment lll , we obtain
Rate = 0.2−1min×0.4molL−1
=0.08molL−1min−1
From experiment IV , we obtain
2.0×10−2molL−1min−1=0.2min−1[A]
⇒[A]=0.1molL−1
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - NCERTCHAPTER - CHEMICAL KINETICSEXERCISE - Exercise
1 Videos
- CHEMICAL KINETICSBOOK - NCERTCHAPTER - CHEMICAL KINETICSEXERCISE - SOLVED EXAMPLE
1 Videos
- BIOMOLECULESBOOK - NCERTCHAPTER - BIOMOLECULESEXERCISE - Exercise
33 Videos
- CHEMISTRY IN EVERYDAY LIFEBOOK - NCERTCHAPTER - CHEMISTRY IN EVERYDAY LIFEEXERCISE - Exercise
32 Videos
Similar Questions
The reaction between A and B is first order with respect to A and zero with respect to B. Fill in tha blanks in the following table:
Experiment[A]/M[B]/MInitial rate/M minI.0.10.12.0×10−2II.....0.24.0×10−2II.0.40.4.......IV.....0.22.0×10−2
View SolutionThe reaction between A and B is first order with respect to A and zero with respect to B. Fill in tha blanks in the following table:
Experiment[A]/M[B]/MInitial rate/M minI.0.10.12.0×10−2II.....0.24.0×10−2II.0.40.4.......IV.....0.22.0×10−2
View Solution
Knowledge Check
The reaction v1A+v2B→ products is first order with respect to A and zero-order with respect to B. If the reaction is started with [A0]and[B0], then the integrated rate expression of this reaction would be:
Aln[A]0[A]0−x=k1tBln[A]0[A]0−v1x=v1k1tCln[A]0[A]0−v1x=k1tDln[A]0[A]0−v1x=−v1k1tFor the raction A+B→ products, it is found that order of A is 2 and the order of B is 3. In the rate expression when the concentration of both A and B are doubled the rate will increases by a factor
A12B16C32D10A reaction of first order with respect to reactant A and second order with respect to reactant B. the rate law for the reaction is given by
ARate=k[A][B]2BRate =[A][B]2CRate =[A]2[B]DRate =k[A]0[B]2
Two coins are tossed, then fill in the blanks in the following table respectively:
View SolutionFrom the following data for the reaction between A and B
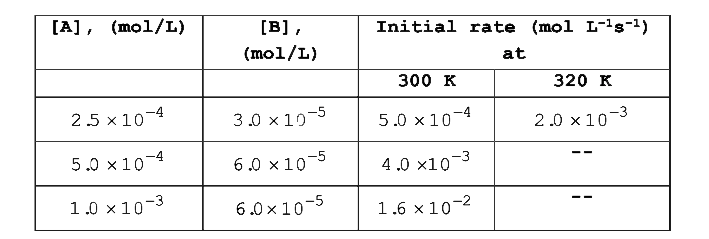
Calculate:
(i) the order of the reaction with respect to A and with respect to B
(ii) the rate constant at 300 K
(iii) the pre-exponential factor.
View SolutionFor a non-equilibrium process A+B→ Produts the rate is first order with respect to A and second order with respect to B. If 1.0 Mole each of A and B are introduced into a one liter vessel and the intial rate is 1.0×10−2mol L−1s−1, the rate when half of the reaction have been eonsumed is:
View SolutionFill in the balanks in the following table which treats reaction of a compound A with a compound B , that is of the first order with respect to A and zero order with respect to B :
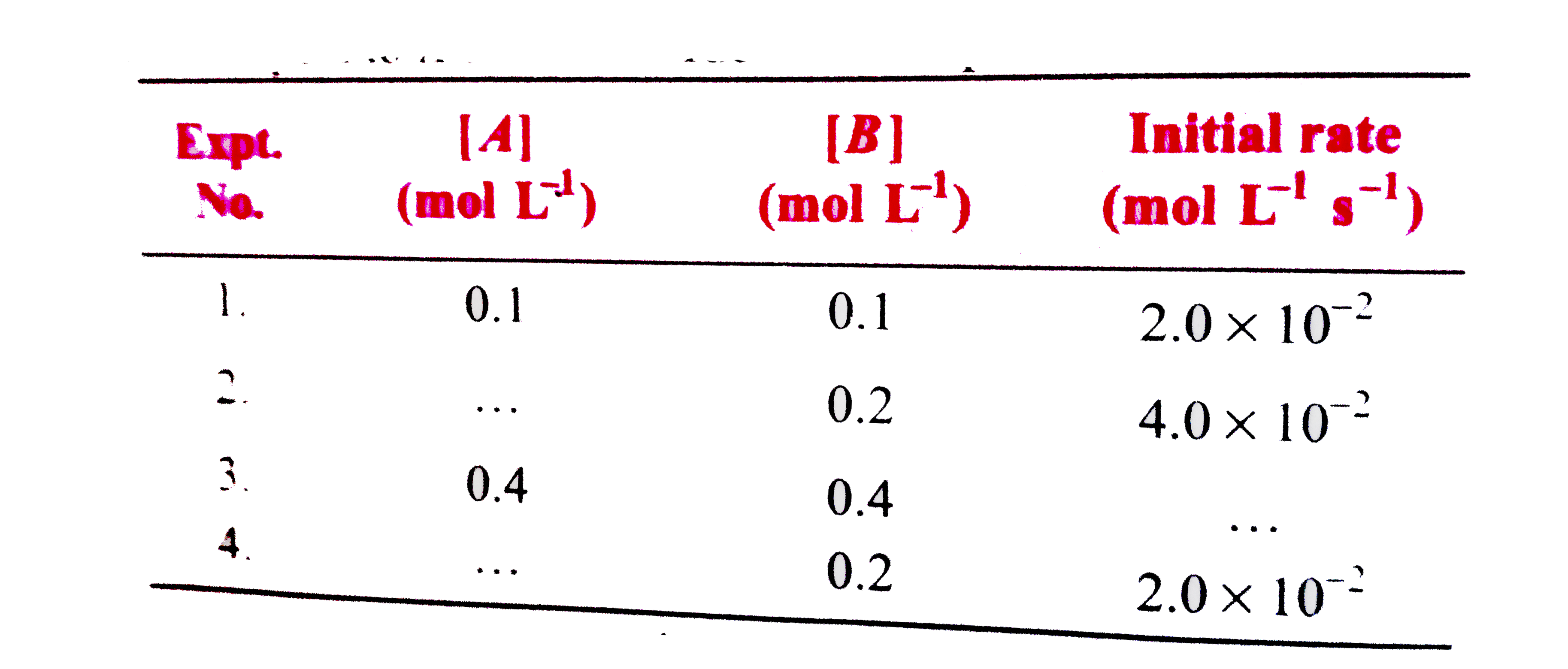
View SolutionFill in the blanks in the following table which treats a reaction of a compound A with a compound B, that is the first order with respect to A and zero order with respect to B.

View Solution
