Unit of visocity of liquids is .........
To determine the unit of viscosity of liquids, we can follow these steps:
Step 1: Understand Viscosity
Viscosity is defined as the resistance of a liquid to flow. It quantifies how much a fluid resists deformation or flow when a force is applied.
Hint: Remember that viscosity relates to how "thick" or "thin" a liquid is in terms of flow.
Step 2: Formula for Viscosity
The formula for viscosity (η) can be derived from the relationship between the applied force (F), the area of contact (A), and the velocity gradient (du/dz). The equation can be expressed as:
F∝A⋅dudz
Where:
- F = applied force
- A = area of contact
- du/dz = velocity gradient (change in velocity with distance)
Hint: Think about how force, area, and velocity gradient interact when a liquid flows.
Step 3: Introduce the Viscosity Coefficient
To remove the proportionality, we introduce a constant (η), which is the coefficient of viscosity:
F=η⋅A⋅dudz
Hint: The coefficient of viscosity (η) is what we are trying to find the units for.
Step 4: Analyze the Units
To find the units of viscosity, we rearrange the formula:
η=F⋅dzA⋅du
Now, substituting the units:
- Force (F) is measured in Newtons (N).
- Distance (dz) is measured in meters (m).
- Area (A) is measured in square meters (m²).
- Velocity (du) is measured in meters per second (m/s).
Thus, the units of viscosity become:
η=N⋅mm²⋅(m/s)
Step 5: Simplify the Units
Now, simplifying the units:
η=N⋅mm²⋅sm=N⋅sm²
Since 1 Newton (N) is equivalent to 1 kg·m/s², we can substitute:
η=kg⋅m/s²⋅sm²=kgm⋅s
Step 6: Final Units of Viscosity
The SI unit of viscosity can also be expressed in terms of Pascal seconds (Pa·s):
1Pa=1Nm²=1kgm⋅s²
Thus, the unit of viscosity is:
1Pa⋅s=1N⋅m−2⋅s
Final Answer: The unit of viscosity of liquids is Pascal second (Pa·s) or Newton meter squared per second (N·m²/s).
---
Viscosity is defined as the resistance of a liquid to flow. It quantifies how much a fluid resists deformation or flow when a force is applied.
The formula for viscosity (η) can be derived from the relationship between the applied force (F), the area of contact (A), and the velocity gradient (du/dz). The equation can be expressed as:
F∝A⋅dudz
Where:
- F = applied force
- A = area of contact
- du/dz = velocity gradient (change in velocity with distance)
To remove the proportionality, we introduce a constant (η), which is the coefficient of viscosity:
F=η⋅A⋅dudz
To find the units of viscosity, we rearrange the formula:
η=F⋅dzA⋅du
- Force (F) is measured in Newtons (N).
- Distance (dz) is measured in meters (m).
- Area (A) is measured in square meters (m²).
- Velocity (du) is measured in meters per second (m/s).
η=N⋅mm²⋅(m/s)
Now, simplifying the units:
η=N⋅mm²⋅sm=N⋅sm²
η=kg⋅m/s²⋅sm²=kgm⋅s
The SI unit of viscosity can also be expressed in terms of Pascal seconds (Pa·s):
1Pa=1Nm²=1kgm⋅s²
Thus, the unit of viscosity is:
1Pa⋅s=1N⋅m−2⋅s
Topper's Solved these Questions
- STATES OF MATTERBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - STATES OF MATTEREXERCISE - Exercises (Ture False)
25 Videos
- STATES OF MATTERBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - STATES OF MATTEREXERCISE - Ex 5.4
32 Videos
- SOME BASIC CONCEPTS AND MOLE CONCEPTBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOME BASIC CONCEPTS AND MOLE CONCEPTEXERCISE - Archives Subjective
11 Videos
- STOICHIOMETRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - STOICHIOMETRYEXERCISE - Archives Subjective
33 Videos
Similar Questions
Viscosity of liquids
View SolutionA liquid has
View SolutionDefine coeffiecient of viscosity of a liquid. Write down its dimensional formula and MKS unit.
View SolutionThe coefficient of viscosity of a liquid of σ=1.0CGS units as determined by measuring the terminal velocity of a spherical solid ball of density p=1.5CGS units inside the liquid. If the ball of radius 1.00cm attain terminal velocity 1cm/s, then the viscosity (in CGS units) of the liquid as calculated from the observations of the experimental is: [Take g=10m/s2 ]
View SolutionComplete the following sentences:
(a) Pressure at a depth h in a liquid of density ρ is …......
(b) Pressure is …... In all direction about a point in a liquid.
(c ) Pressure at all points at the same depth is ….
(d) Pressure at a point inside a liquid is ....... to its depth.
(e ) Pressure of a liquid at a given depth is ....... to the density of liquid.
View SolutionIn case of a liquid
View SolutionWhen a liquid flows in a tube, there is relative motion between adjacent layers of the liquid. This force is called the viscous force which tends to oppose the relative motion between the layers of the liquid. Newton was the first person to study the factors that govern the viscous force in a liquid. According to Newton’s law of viscous flow, the magnitude of the viscous force on a certain layer of a liquid is given by
F=−ηAdvdx
where A is the area of the layer dvdx is the velocity gradient at the layer and η is the coefficient of viscosity of the liquid.
A river is 5 m deep. The velocity of water on its surface is 2ms−1 If the coefficient of viscosity of water is 10−3Nsm−2 , the viscous force per unit area is :
View SolutionIn a thermometer, the commonly used liquid is...........
View SolutionPressure exerted by a liquid is ..................... proportional to the density of liquid.
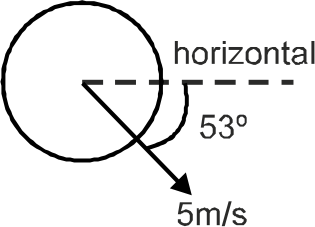
View SolutionAn external force 6N is applied on a sphere of radius R = 10 cm of mass 1 kg and the sphere moves in a liquid with a constant velocity 5 m/s making 530 with the horizontal.The coefficient of viscosity of the liquid is 20/(6π), in S.I units. (Take g=10m/s2)
View Solution
