Doubtnut Promotions Sticky

Write the values of n and l for 3p orbital.

Video Solution
Doubtnut Promotions Banner
Text Solution
Generated By DoubtnutGPT

To find the values of the principal quantum number (n) and the azimuthal quantum number (l) for the 3p orbital, we can follow these steps:

Step 1: Identify the Principal Quantum Number (n)
The principal quantum number (n) indicates the energy level or shell in which the electron resides. In the case of the 3p orbital, the number "3" indicates that the electron is in the third energy level.

Value of n:
- n = 3

Step 2: Identify the Azimuthal Quantum Number (l)
The azimuthal quantum number (l) defines the shape of the orbital and is associated with the subshell. For different types of orbitals, the values of l are as follows:
- s orbital: l = 0
- p orbital: l = 1
- d orbital: l = 2
- f orbital: l = 3

Since we are dealing with a p orbital in the 3p subshell, the value of l for a p orbital is 1.

Value of l:
- l = 1

Final Answer
Thus, for the 3p orbital:
- n = 3
- l = 1

---

|
Updated on:7/8/2024
Class 11CHEMISTRYSTRUCTURE OF ATOM

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    In an orbital electrons are filled according to Aufbau principle, Pauli's exclusion principle and Hund's rule of maximum muliplicity .
    According to Aufbau principal the orbital are filled in order to their increasing energies . In the order of increase of energy of orbitals cna be calculated from (n+l) rule. Lower the value of (n+l) for an orbital the lowest energy hence orbital are filled in order of increasing (n+l) value. If two orbitals have same (n+l) value, the orbital with lower value of 'n' has lower energy hence it is filled first.
    According to Pauli's exclusion principle, an orbital can have maximum two electrons with opposite spin.
    According to Hunds rule pairing of electron in degenerate orbitals of the same sub shell does not take place until each orbital belonging to that sub shell has got one electron each i.e., singly occupied.
    The electron identified by quantum number n & l
    (i) n = 4, l = 1 (ii) n = 4, l = 0
    ( iii) n = 3, l = 2 (iv) n = 3, l = 1
    can be placed in order of increasing energy from the lowest to the highest as

    A(iv)<(ii)<(iii)<(i)
    B(ii)<(iv)<(i)<(iii)
    C(i)<(iii)<(ii)<(iv)
    D(iii)<(i)<(iv)<(ii)
  • Question 2 - Select One

    In an orbital electrons are filled according to Aufbau principle, Pauli's exclusion principle and Hund's rule of maximum muliplicity .
    According to Aufbau principal the orbital are filled in order to their increasing energies . In the order of increase of energy of orbitals cna be calculated from (n+l) rule. Lower the value of (n+l) for an orbital the lowest energy hence orbital are filled in order of increasing (n+l) value. If two orbitals have same (n+l) value, the orbital with lower value of 'n' has lower energy hence it is filled first.
    According to Pauli's exclusion principle, an orbital can have maximum two electrons with opposite spin.
    According to Hunds rule pairing of electron in degenerate orbitals of the same sub shell does not take place until each orbital belonging to that sub shell has got one electron each i.e., singly occupied.
    If n=4, how many element are possible?

    A20
    B14
    C32
    D16
  • Question 3 - Select One

    For an orbital the graph between ψ(r) and r (distance from nucles) is :
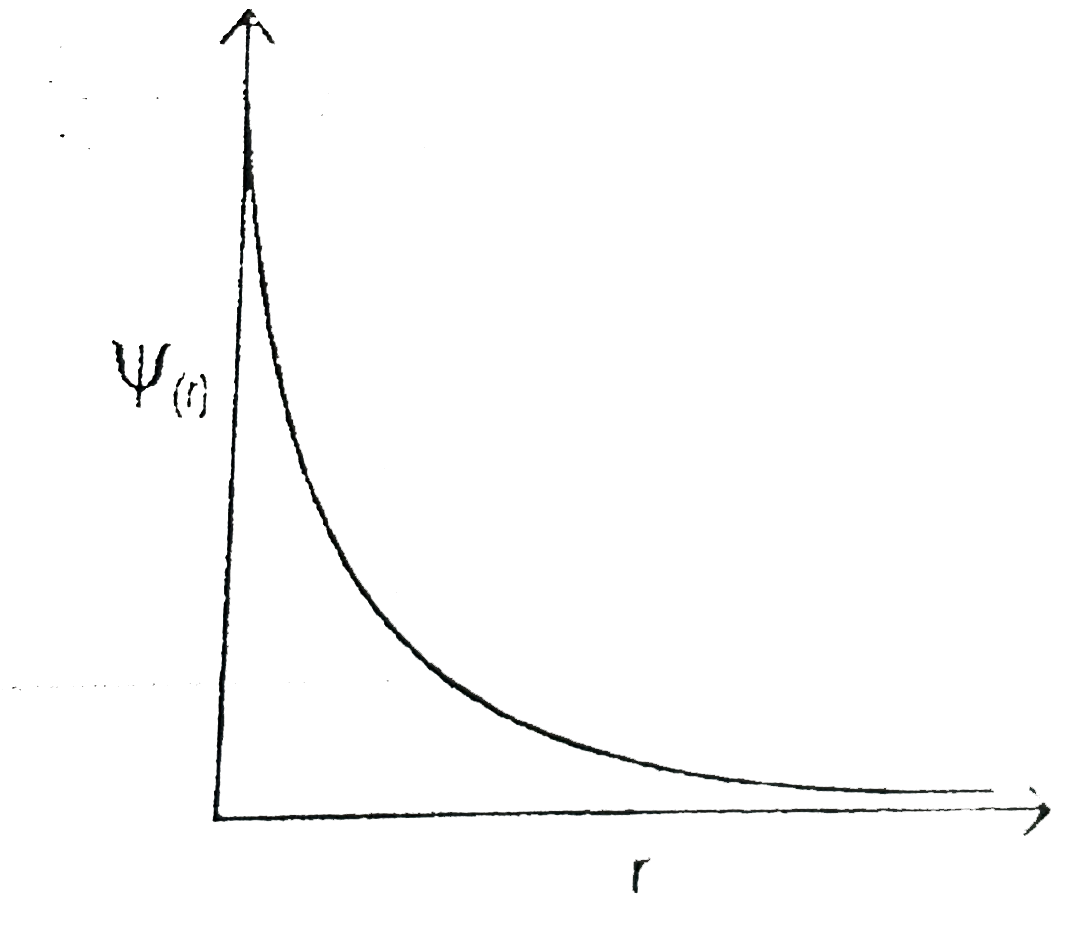
    ocr_image
    Find the value of n+l of the orbital.

    A1
    B2
    C3
    DNone of these