1 kg of water having a volume of becomes of steam when boiled at a pressure of one atmosphere. The heat of vapourization at this pressure is 540 kcal/kg. Calculate the increase in internal energy and the work done in expansion.
To solve the problem step by step, we will calculate the work done during the expansion and the increase in internal energy using the first law of thermodynamics.
Step 1: Convert the heat of vaporization to joules
The heat of vaporization is given as 540kcal/kg. We need to convert this to joules.
Heat of vaporization=540kcal/kg×4184J/kcal=2.26×106J/kg
Step 2: Calculate the total heat absorbed by the water
Since we have 1 kg of water, the total heat absorbed Q is:
Q=2.26×106J
Step 3: Calculate the change in volume
The initial volume of water is Vi=10−3m3 and the final volume of steam is Vf=1.67m3. The change in volume ΔV is:
ΔV=Vf−Vi=1.67−10−3=1.669m3
Step 4: Calculate the work done in expansion
The work done W during the expansion at constant pressure is given by:
W=PΔV
Where P=1.013×105Pa (pressure in pascals).
Substituting the values:
W=1.013×105Pa×1.669m3=1.69×105J
Step 5: Apply the first law of thermodynamics
According to the first law of thermodynamics, the change in internal energy ΔU is given by:
ΔU=Q−W
Substituting the values we calculated:
ΔU=2.26×106J−1.69×105J
Calculating this gives:
ΔU=2.26×106J−0.169×106J=2.091×106J
Final Results
- Work Done (W): 1.69×105J
- Increase in Internal Energy (ΔU): 2.091×106J
The heat of vaporization is given as 540kcal/kg. We need to convert this to joules.
Since we have 1 kg of water, the total heat absorbed Q is:
The initial volume of water is Vi=10−3m3 and the final volume of steam is Vf=1.67m3. The change in volume ΔV is:
The work done W during the expansion at constant pressure is given by:
Where P=1.013×105Pa (pressure in pascals).
According to the first law of thermodynamics, the change in internal energy ΔU is given by:
- Work Done (W): 1.69×105J
- Increase in Internal Energy (ΔU): 2.091×106J
Topper's Solved these Questions
- INTERNAL ENERGY Book:ICSEChapter:INTERNAL ENERGY Exercise:SELECTED PROBLEMS (FROM ISOTHERMAL AND ADIABATIC PROCESSES )
Explore 10 Videos
- INTERNAL ENERGY Book:ICSEChapter:INTERNAL ENERGY Exercise:SELECTED PROBLEMS (FROM WORK DONE AND INDICATOR DIAGRAM )
Explore 13 Videos
- INTERNAL ENERGY Book:ICSEChapter:INTERNAL ENERGY Exercise:VERY SHORT ANSWER QUESTIONS
Explore 17 Videos
- GRAVITATIONBook:ICSEChapter:GRAVITATIONExercise:FROM THE HUBBLE TELESCOP
Explore 2 Videos
- MOTION IN FLUIDS Book:ICSEChapter:MOTION IN FLUIDS Exercise:SELECTED PROBLEMS (FROM POISEUILLE.S FORMULA)
Explore 19 Videos
Similar Questions
At 1 atmospheric pressure, 1.000 g of water having a volume of 1.000cm3 becomes 1671 cm3 of steam when boiled. The heat of vaporization of water at 1 atmosphere is 539cal/g . What is the change in internal energy during the process ?
View SolutionOne gram of water (1cm3) becomes 1671cm3 of steam when boiled at a constant pressure of 1 atm (1.013×105Pa). The heat of vaporization at this pressure is Lv=2.256×106J/kg. Compute (a) the work done by the water when it vaporizes and (b) its increase in internal energy.
View SolutionOne gram of water (1cm3) becomes 1671cm3 of steam when boiled at a constant pressure of 1 atm (1.013×105Pa). The heat of vaporization at this pressure is Lv=2.256×106J/kg. Compute (a) the work done by the water when it vaporizes and (b) its increase in internal energy.
View SolutionAt 1 atmospheric pressure, 1000 g of water having a volume of 1000cm3 becomes 1.097cm3 of ice on freezing. The heat of fusion of water at 1 atmosphere is 80.0cal/g. What is the change in internal energy during the process?
View SolutionA gas occupying a volume of 10−2m3. at a pressure of 5 atmospheres expands isothermally to a pressure of 1 atmosphere. Calculate the work done.
View SolutionThe volume of a monatomic ideal gas increases linearly with pressure, as shown in Fig. Calculate (a) increase in internal energy, (b) work done by the gas and (c ) heat supplied to the gas.
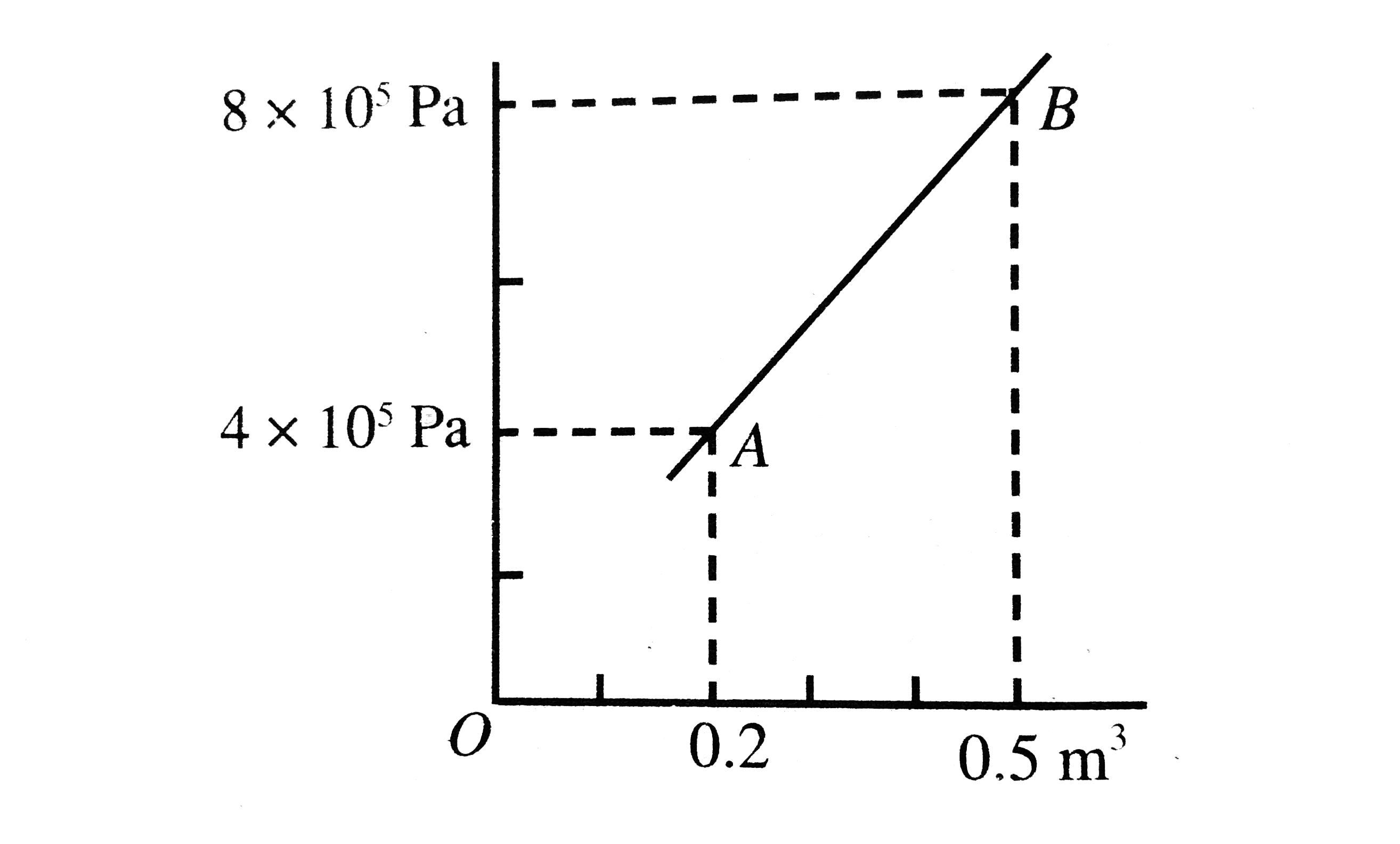
View SolutionA gas expands from 75 liter to 125 liter at a constant pressure of 4 atmosphere. If one atmospheric pressure is 1.0x105Pa(Nm−2), calculate the work done by the gas during this expansion.
View SolutionAn ideal gas of volume 1 litre and at a pressure 8 atm undergoes an adiabatic expansion until its pressure drops to 1 atm and volume increase to 4 liters. Calculate the work done in the process (y = 1.5).
View Solution1 g of water at 100∘C is completely converted into steam at 100∘C. 1g of steam occupies a volume of 1650cc. (Neglect the volume of 1g of water at 100∘C). At the pressure of 105N/m2, latent heat of steam is 540 cal/g (1 Calorie=4.2 joules). The increase in the internal energy in joules is
View Solution540 calories of heat convert 1 cubic centimeter of water at 100oC into 1671 cubic centimeter of steam at 100oC at a pressure of one atmosphere. Then the work done against the atmospheric pressure is nearly
View Solution