^57Codecays→^57Fe byβ+ -emission.The resulting ^57Feis∈itsexcitedstateandcomes→thegroundstatebyemi∈ggamma−rays.Thehalf−lifeofbeta^+−decayis270daysandtˆofthegamma−emissionis10^(-8)s.Asamp≤of^57Co given 5.0×109gamma rays per second.How much time will elapse before the emission rate of gamma rays drops to 2.5×109 per second ?
The correct Answer is:A, B, D
According to the question , the emistion rate of γ−rays will drop to the half when the β + decays to half of its original amount and for this the sample would take 270 days
∴Thisrequiredtimeis270days.
Topper's Solved these Questions
Similar Questions
.12N beta decays to an ecited state of .12C, which subsequenctly decays to the ground state with the emission of a 4.43−MeV gamma ray. What is the maxium kinetic energy of the emitted beta particle?
View SolutionThe half-life of _92238U against alpha decay is 4.5×109 year. How much disintegration per second occurs in 1 g of _92238U?
View Solution
Knowledge Check
Uranium- 238 decays to thorium-234 with half-life 5×109yr. The resulting nucleus is in the excited state and hence further emits γ-rays to come to the ground state. It emits 20γ-rays per second. The emission rate will drop to 5γ-rays per second in
A1.25×109yrB1010yrC10−8yrD1.25×10−9sGold nucleus (19879Au) can decay into mercuyr nucleus (19880Hg8) by two decay schemes shown in figure below (i) it can emit a β -particle (β1) and come to ground state by either emiting one γ- ray (γ1) or emitting two γ- rays (γ3 and γ4) (ii) It can emit one β- particle (β2) and come to ground state by emitting γ2 ray. Atomic masses: 198Au=197.9652 amu and 198Hg=197.9662 amu. Given that 1 amu= 930MeV/c2. The energy levels of the nucleus are shown in figure below
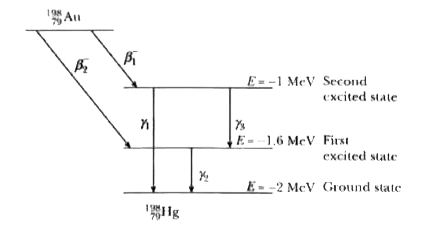
The wavelength of emitted γ - rays is the orderAλ2>λ3>λ1Bλ3>λ2>λ1Cλ1>λ2>λ3Dλ3=λ2=λ1Gold nucleus (19879Au) can decay into mercuyr nucleus (19880Hg8) by two decay schemes shown in figure below (i) it can emit a β -particle (β1) and come to ground state by either emiting one γ- ray (γ1) or emitting two γ- rays (γ3 and γ4) (ii) It can emit one β- particle (β2) and come to ground state by emitting γ2 ray. Atomic masses: 198Au=197.9652 amu and 198Hg=197.9662 amu. Given that 1 amu= 930MeV/c2. The energy levels of the nucleus are shown in figure below
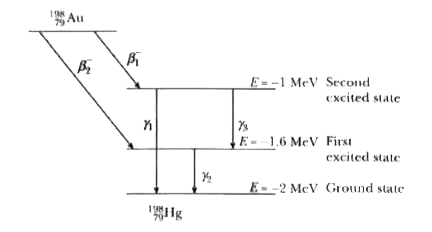
Whatis the maximum kinetic energy of emitted β2 particle?A1.44 MeVB0.59 MeVC1.86 MeVD1.46 MeV
A F32 radio nuclide with half-life T=14.3 days is produced in a reactor at a constant rate q=2×109 nuclei per second. How soon after the beginning of production of that radio nuclide will its activity be equal to R=109 disintegration per second?
View SolutionIf the average life time of an excited state of hydrogen is of the order of 10−8s, estimate how many whits an alectron makes when it is in the state n=2 and before it suffers a transition to staten=1(Bohrrediusa0=5.3×10−11m)?
View SolutionA sample of radon emitted initially 7×104α-particles per second. After some time, the emission rate became 2.1×104. If t12 for radon is 3.8 days, find the age of the sample
View SolutionGold nucleus (19879Au) can decay into mercuyr nucleus (19880Hg8) by two decay schemes shown in figure below (i) it can emit a β -particle (β1) and come to ground state by either emiting one γ- ray (γ1) or emitting two γ- rays (γ3 and γ4) (ii) It can emit one β- particle (β2) and come to ground state by emitting γ2 ray. Atomic masses: 198Au=197.9652 amu and 198Hg=197.9662 amu. Given that 1 amu= 930MeV/c2. The energy levels of the nucleus are shown in figure below
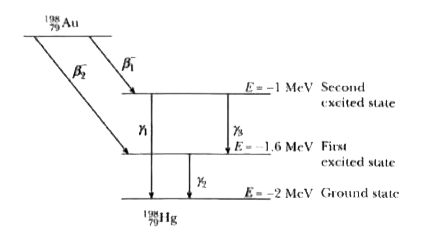
What is the maximum kinetic energy of emitted β1 parricle ?
View SolutionA .32P radionuclide with half - life T = 14.3 days is produced in a reactor at a constant rate q=2.7×109 nuclei per second. How soon after the beginning of production of that nuclide will its activity be equal to A=1.0×109dis./s?
View Solution
