A 40 cm wire having a mass of 3.2 g is stretched between two fixed supports 40.05 cm apart. In its fundamental mode, the wire vibrates at 220 Hz. If the area of cross section of the wire is 1.0mm2, find its Young modulus.
The correct Answer is:1.985xx10^11N/m^2
To find the Young's modulus of the wire, we will follow these steps:
Step 1: Calculate the Change in Length (ΔL)
The original length of the wire is given as 40 cm, and the stretched length is 40.05 cm.
ΔL=Stretched Length−Original Length=40.05cm−40cm=0.05cm=0.0005m
Step 2: Calculate the Strain (ε)
Strain is defined as the change in length divided by the original length.
Strain(ϵ)=ΔLL=0.0005m0.4m=0.00125
Step 3: Calculate the Mass per Unit Length (μ)
The mass of the wire is given as 3.2 g, which needs to be converted to kg.
Mass=3.2g=3.2×10−3kg
The length of the wire is 40 cm or 0.4 m. Thus, the mass per unit length (μ) is:
μ=MassL=3.2×10−3kg0.4m=8×10−3kg/m
Step 4: Calculate the Velocity (v)
The frequency of vibration is given as 220 Hz. The wavelength (λ) for the fundamental mode is given by:
λ=2L=2×0.4m=0.8m
Now, we can calculate the velocity (v) using the formula:
v=f⋅λ=220Hz×0.8m=176m/s
Step 5: Calculate the Tension (T)
Using the relationship between velocity, tension, and mass per unit length:
v=√Tμ⟹T=μv2
Substituting the values:
T=(8×10−3kg/m)⋅(176m/s)2
Calculating v2:
v2=1762=30976m2/s2
Now substituting back:
T=8×10−3⋅30976=248.81N
Step 6: Calculate the Young's Modulus (Y)
Young's modulus is defined as:
Y=T/Aϵ
Where A is the area of cross-section. The area is given as 1.0mm2, which needs to be converted to m2:
A=1.0mm2=1.0×10−6m2
Now substituting the values into the Young's modulus formula:
Y=T/Aϵ=248.81N/(1.0×10−6m2)0.00125
Calculating:
Y=248.81×1060.00125=198928800N/m2≈1.99×1011N/m2
Final Answer
The Young's modulus of the wire is approximately:
Y≈1.99×1011N/m2
---
The original length of the wire is given as 40 cm, and the stretched length is 40.05 cm.
Strain is defined as the change in length divided by the original length.
The mass of the wire is given as 3.2 g, which needs to be converted to kg.
The frequency of vibration is given as 220 Hz. The wavelength (λ) for the fundamental mode is given by:
Using the relationship between velocity, tension, and mass per unit length:
Young's modulus is defined as:
The Young's modulus of the wire is approximately:
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Question for short Answer7videos
WAVE MOTION AND WAVES ON A STRING
- BOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Objective -29videos
WAVE MOTION AND WAVES ON A STRING
- BOOK - HC VERMA ENGLISHCHAPTER - THE FORCESEXERCISE - Questions for short Answer9videos
THE FORCES
- BOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Question for Short Answer16videos
WORK AND ENERGY
Similar Questions
A 40 cm long wire having a mass 3.1 gm and area of cross section 1mm2 is stretched between the support 40.05 cm apart. In its fundamental mode , it vibrates with a frequency 1000/64Hz. Find the young's modulus of the wire in the form X×108N−m2 and fill value of X.
View SolutionFigure shows an aluminium wire of length 60cm joined to a steel wire of length 80cm and stretched between two fixed supports. The tension produced is 40N. The cross-sectional area of the steel wire is 1.0mm2 and that of the aluminimum wire is 3.0mm2 The minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node is 10P (in Hz) the find P. Given density of aluminimum is 2.6g/cm3 and that of steel is 7.8g/cm3.
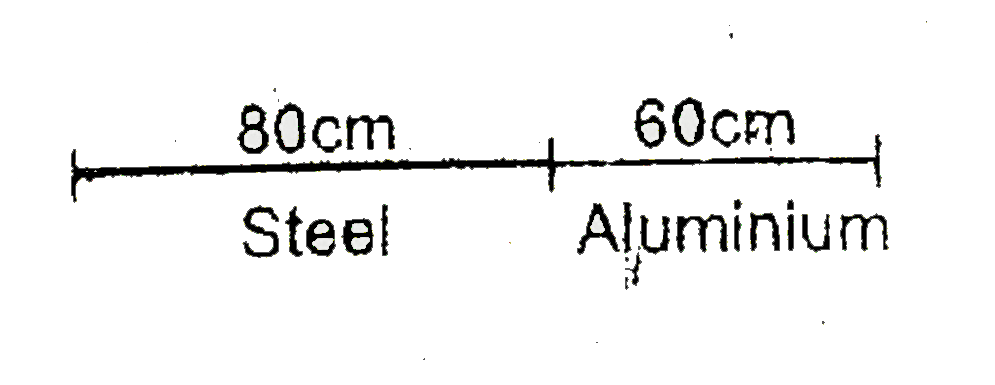 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A wire is stretched between two rigid supports vibrates in its fundamental mode with a frequency of 50 Hz. The mass of the wire is 30 g and its linear density is 4×10−2 kg m s−1. The speed of the transverse wave at the string is
A25ms−1B50ms−1C75ms−1D100ms−1
A steel wire of length 1m and density 8000kg/m3 is stretched tightly between two rigid supports . When vibrating in its fundamental mode , its frequency is 200Hz.
a. What is the velocity of transverse wave along this wire ?
b. What is the longitudinal stress in the wire ?
c. If the maximum acceleration of the wire is 880m/s2, what is the amplitude of vibration at the midpoint ?View SolutionWhen a wire of length 10m is subjected to a force of 100N along its length, the lateral strain produced is 0.01×10−3. The Poisson's ratio was found to be 0.4. If the area of cross-section of wire is 0.025m2, its Young's modulus is
View SolutionA wire having a linear density of 0.05g/cmis stretched between two rigid supports with a tension of 450N. It is observed that the wire resonates at a frequency of 420 Hz. The next higher frequency at which the same wire resonates is 490 Hz. Find the length of the wire.
View SolutionA wire having a linear density of 0.05g/cmis stretched between two rigid supports with a tension of 450N. It is observed that the wire resonates at a frequency of 420Hz. The next higher frequency at which the same wire resonates is 490Hz. Find the length of the wire.
View SolutionA wire having a linear mass density 5.0×103kg/m is stretched between two rigid supports with tension of 450 N. The wire resonates at a frequency of 420Hz. The next higher frequency at which the same wire resonates is480N. The length of the wire is
View SolutionA wire with mass 40.0 g is stretched so that its ends are tied down at points 80.0 cm apart.The wire vibrates in its fundamental mode with frequency 60.0 Hz and with an amplitude at the antinodes of 0.300 cm.
(a) What is the speed of propagation of transverse wave in the wire?
Compute the tension in the wire.
(c) Find the maximum transverse velocity and acceleration of particles in the wire.View SolutionA 30.0-cm-long wire having a mass of 10.0 g is fixed at the two ends and is vibrated in its fundamental mode.A 50.0-cm-long closed organ pipe, placed with its open end near the wire, is set up into resonance in its fundamental mode by the vibrating wire. Find the tension in the wire. Speed of sound in air =340ms−1.
View Solution

