A ball is rolled off the edge of a horizontal table at a speed of 4m/second. It hits the ground after 0.4 second . Which statement given below is true
It hits the ground at a horizontal distance 1.6 m from the edge of the table
The speed with which it hits the ground is 4.0 m/second
Height of the table is 0.8m
It hits the ground at an angle of 60∘ to the horizontal
The correct Answer is:A, C
To solve the problem step by step, we need to analyze the motion of the ball after it rolls off the edge of the table.
Step 1: Determine the vertical distance (height of the table)
The ball is rolled off the table horizontally with an initial horizontal velocity of ux=4m/s. The time taken to hit the ground is t=0.4s.
In the vertical direction, the initial vertical velocity uy=0 (since it is rolled off horizontally). The only force acting on the ball in the vertical direction is gravity, which causes a downward acceleration a=g=10m/s2.
Using the second equation of motion:
S=ut+12at2
Substituting the values:
S=0⋅0.4+12⋅10⋅(0.4)2
S=0+12⋅10⋅0.16
S=5⋅0.16=0.8m
Thus, the height of the table is 0.8m.
Step 2: Calculate the horizontal distance traveled
In the horizontal direction, the horizontal velocity remains constant since there is no acceleration. The horizontal distance Sx can be calculated as:
Sx=ux⋅t
Substituting the values:
Sx=4m/s⋅0.4s=1.6m
Thus, the ball travels a horizontal distance of 1.6m before hitting the ground.
Step 3: Determine the final vertical velocity
To find the final vertical velocity vy just before hitting the ground, we can use the first equation of motion:
v2y=u2y+2aS
Substituting the values:
v2y=0+2⋅10⋅0.8
v2y=16⟹vy=√16=4m/s
Step 4: Calculate the resultant velocity just before hitting the ground
The resultant velocity v just before hitting the ground can be calculated using the Pythagorean theorem:
v=√v2x+v2y
Where vx=4m/s (horizontal velocity) and vy=4m/s (vertical velocity):
v=√(4)2+(4)2=√16+16=√32=4√2m/s≈5.66m/s
Step 5: Determine the angle of impact
The angle θ with respect to the horizontal can be found using:
tan(θ)=vyvx
Substituting the values:
tan(θ)=44=1⟹θ=45∘
Conclusion
Based on the calculations:
1. The height of the table is 0.8m.
2. The horizontal distance traveled is 1.6m.
3. The speed at which it hits the ground is approximately 5.66m/s.
4. The angle of impact is 45∘.
True Statements
- The ball hits the ground at a horizontal distance of 1.6m from the edge of the table.
- The height of the table is 0.8m.
The ball is rolled off the table horizontally with an initial horizontal velocity of ux=4m/s. The time taken to hit the ground is t=0.4s.
S=ut+12at2
Substituting the values:
S=0⋅0.4+12⋅10⋅(0.4)2
S=0+12⋅10⋅0.16
S=5⋅0.16=0.8m
Thus, the height of the table is 0.8m.
In the horizontal direction, the horizontal velocity remains constant since there is no acceleration. The horizontal distance Sx can be calculated as:
Sx=ux⋅t
Substituting the values:
Sx=4m/s⋅0.4s=1.6m
Thus, the ball travels a horizontal distance of 1.6m before hitting the ground.
To find the final vertical velocity vy just before hitting the ground, we can use the first equation of motion:
v2y=u2y+2aS
Substituting the values:
v2y=0+2⋅10⋅0.8
v2y=16⟹vy=√16=4m/s
The resultant velocity v just before hitting the ground can be calculated using the Pythagorean theorem:
v=√v2x+v2y
Where vx=4m/s (horizontal velocity) and vy=4m/s (vertical velocity):
v=√(4)2+(4)2=√16+16=√32=4√2m/s≈5.66m/s
The angle θ with respect to the horizontal can be found using:
tan(θ)=vyvx
Substituting the values:
tan(θ)=44=1⟹θ=45∘
Based on the calculations:
1. The height of the table is 0.8m.
2. The horizontal distance traveled is 1.6m.
3. The speed at which it hits the ground is approximately 5.66m/s.
4. The angle of impact is 45∘.
- The ball hits the ground at a horizontal distance of 1.6m from the edge of the table.
- The height of the table is 0.8m.
Topper's Solved these Questions
Similar Questions
A ball rolls off the edge of a horizontal table top 4 m high. If it strikes the floor at a point 5 m horizontally away from the edge of the table, what was its speed at the instant it left the table?
View SolutionA body projected up reaches a point A in its path at the end of 4^( th) second and reaches the ground after 5 seconds from the start.The height of A above the ground is (g=10ms^(-2)) in m
View Solution
Knowledge Check
A ball is rolled off the edge of a horizontally table at as speed of 4m/s. It hits the ground after 0.8s.
(i) it hits the ground at a horizontal distance 3.2m from the edge of the table
(ii) The speed with which it hits the ground is 4√5m/s
(iii) Height of the table is 3.2m
(iv) it hits the ground at an angle of tan−1(2) to the horizontallyA(i), (ii)B(ii), (iii), (iv)C(i), (ii), (iii)Dall option are correctA ball is rolled off along the edge of a horizontal table with velocity 4m/s. It hits the ground after time 0.4s. Which of the following are correct?
AThe height of the table is 0.8mBIt hits the ground at an angle of 60∘ with the verticalCIt covers a horizontal distance 1.6m from the tableDIt hits the ground with vertical velcotiy 4m/sA ball is rolled off along the edge of a horizontal table with velocity 4m/s. It hits the ground after time 0.4s. Which of the following are correct?
AThe height of the table is 0.8mBIt hits the ground at an angle of 60∘ with the verticalCIt covers a horizontal distance of 1.6m from the tableDIt hits the ground with vertical velocity 4m/s
A wet ball is projected horizontally at a speed of u=10m/s from the top of a tower h=31.25m high. Water drops detach from the ball at regular intervals of Δt=1.0s after the throw.
(a) How many drops will detach from the ball before it hits the ground.
(b) How far away the drops strike the ground from the point where the ball hits the ground?
View SolutionA small ball rolls horizontally off the edge of a tabletop that is 1.50 m high. It strikes the floor at a point 1.52 m horizontally from the table edge. (a) How long is the ball in the air? (b) What is its speed at the instant it leaves the table?
View SolutionA ball is rolled off along the edge of the table (horizontal ) with velocity 4ms−1 .If hits the ground after time 0.4 s. Which one of the following statements is wrong ? ( g= 10 ms^(-2)).
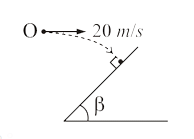
View SolutionA ball is thrown horizontally from a point O with speed 20 m/s as shown. Ball strikes the incline along normal after two seconds
The displacement of ball in two seconds will be:
View SolutionA ball is thrown horizontally with a speed of 20m/s from the top of a tower of height 100m
velocity with which the ball strikes the ground is .
View Solution
