Question

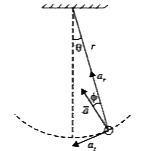
A ball is suspended by a string of length $0.75m$ and is made to oscillate in a vetical circle. The ball has a speed of $1m\text{/}s$ when $\theta ={10}^{\circ }$. What is the magnitude of the radical component of the acceleration at that instant? What is magnitude of the tangential acceleration? Find the magnitude and direction of the total acceleration when $\theta ={10}^{\circ }$.

Explanation

Length of the string `= r= 0.75 m`
Speed `= 1 m"/"s, theta = 10^(@)`
Radial component of acceleration `a_(r )= (v^2)/(r )= (1^2)/(0.75)= 1.33 ms^(-2)`
Tangential acceleration `a_(r )= g sin theta = 9.8 sin 10`
`=9.8 xx 0.1736= 1.70 ms^(-2)`
Total acceleration `veca= vec(a_r)+vec(a_t)`
Magnitude of `veca= sqrt(a_(r )^(2)+a_(t)^(2))= sqrt(1.33^(2)+1.7^(2))= 2.16 ms^(-2)`
Direction of the accleration is given by `phi`
`phi= tan^(-1)((a_t)/(a_r))= tan^(-1)((1.7)/(1.33))= 53^(@)`.