A ball is thrown upwards into air with a speed greater than its terminal speed. It lands at the same place from where it was thrown. Mark the correct statement (s).
It acquires terminal speed before it gets to the highest point of the trajectory.
Before reaching the highest point of trajectory, its speed is continuously decreasing.
During the entire flight, the force of air
The magnitude of net force experienced by the ball is maximum just after it is thrown.
The correct Answer is:B, C, D
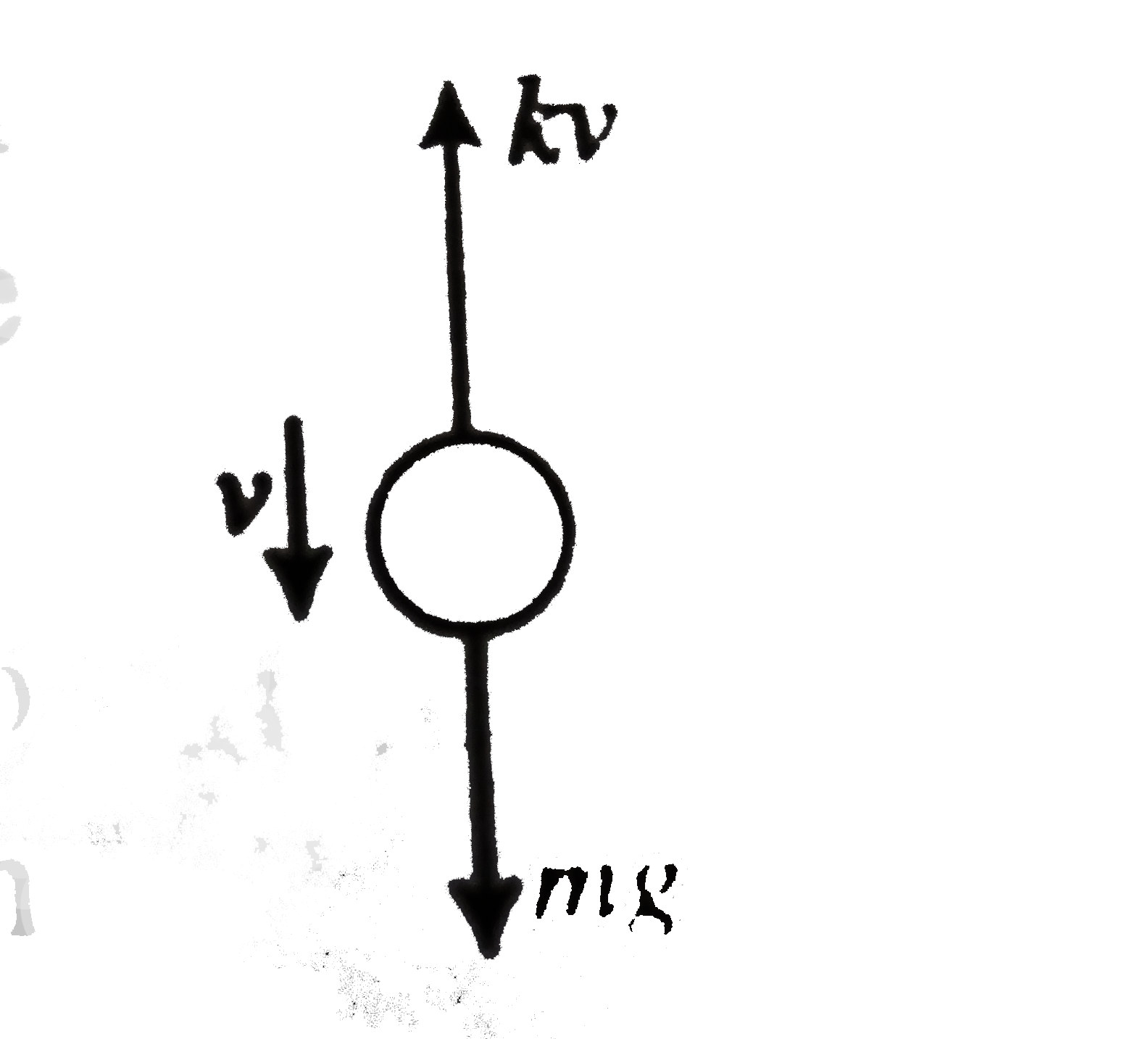
b,c,d. Initially, the FBD of ball would be as shown in figure.
Here, directions of velocity and acceleration are opposite, so particle is slowing down and moving
in upwards direction. At one instant its velocity
becomes zero, which would be the highest point of
trajectory. Then it starts falling down, for its descent
FBD would be as shown in figure.

During its descent, the ball will acquire the terminal speed (assuming ball is not striking ground before acquiring the terminal speed.)
As work has been done against the resistive force, so
speed of throwing is greater than speed with which
the particle lands.
hence, force of air friction is greatest (when speed is greatest) just after it is thrown.
Topper's Solved these Questions
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Linked Comprehension Type
35 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Integer Type
17 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICSCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Multiple Correct Answer Type
9 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE
10 Videos
Similar Questions
Knowledge Check
A ball is thrown upwards with a speed u from a height h above the ground.The time taken by the ball to hit the ground is
A√2h/gB√8h/gC√u2+2ghgDug+√2hgA ball of mass 1kg is thrown up with an initial speed of 4m/s. A second ball of mass 2kg is released from rest from some height as shown in Fig. Choose the correct statement (s).
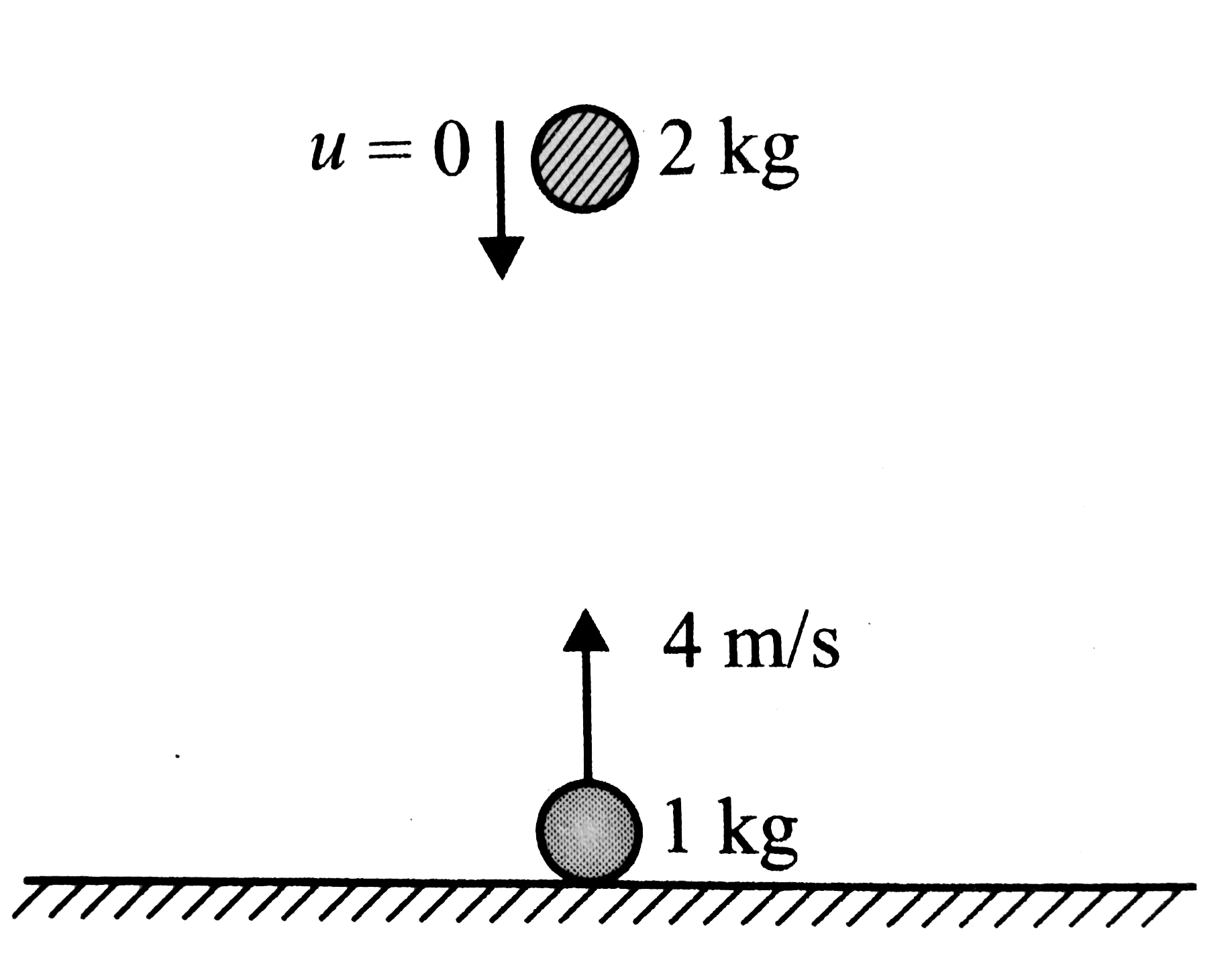 AThe centre of mass of the two balls comes down with acceleration g/3.BThe centre of mass first moves up and then comes down.CThe acceleration of the centre of mass is g downwards.DThe centre of mass of the two balls remains stationary.
AThe centre of mass of the two balls comes down with acceleration g/3.BThe centre of mass first moves up and then comes down.CThe acceleration of the centre of mass is g downwards.DThe centre of mass of the two balls remains stationary.A ball is thrown straight upward with a speed v from a point h meter above the ground. The time taken for the ball to strike the ground is
Avg[1+√1+2hgv2]Bvg[1−√1−2hgv2]Cvg[1−√1+2hgv2]Dvg[2+2hgv2]
A ball is thrown upwards with speed v from the top of a tower and it reaches the ground with speed 3v .The height of the tower is
View SolutionA ball is thrown vertically upwards with a speed of 9.8 m/s from the ground. Draw the x-t, v-t and a-t graph for its motion.
View SolutionA ball is thrown from a point on ground and it lands on same level at distance of
View SolutionA ball thrown upwards takes 4 s to reach the maximum height. Find the initial speed with which it was thrown.
View SolutionA stone is thrown upwards from the top of a tower with some initial speed and it reaches the ground in 16 seconds. Now it is thrown with the same initial speed downward and it reaches the ground in 9sec. In how much time will it reach the ground if the stone is allowed to fall freely under gravity from the same place ?
View Solution

