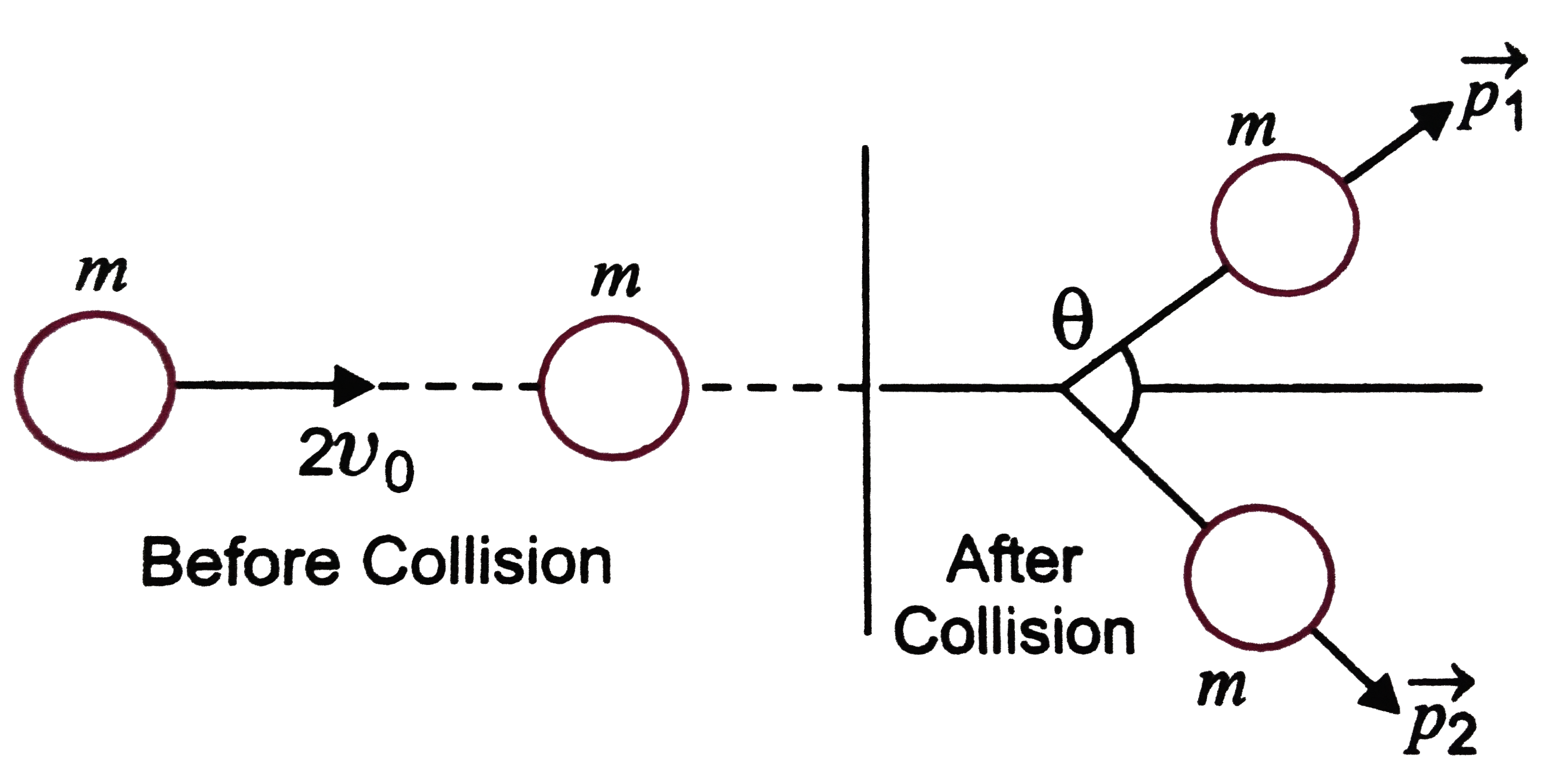
A ball of mass mm, moving with a speed 2υ0, collides inelasticaly (e>0) with an identical ball at rest. Show that (a) For head - on collision, both the balls move forward.
(b) For a genergcollision, the angle between the two velocities of scattered balls is less that 90∘.
(a) If υ1,υ2 are velocities of two balls after collision, then according to the principle of conservation of linear momentum.
m(2υ0)=mυ1+mυ2 or 2υ0=υ1+υ2 …(i)
By definition, e=υ2−υ1υ2+υ1=υ2−υ12υ0 ∴υ2=υ1+2υ0e ...(ii)
From (i), 2υ0=υ1+(υ1+2υ0e)
or =2(υ1+υ0e) or υ1=υ0(1−e)
As e<1,υ1 has the same sign sign as υ0. ∴ the ball moves forward after collision.
From (ii), υ2=υ0(1−e)+2υ0e=υ0(1+e)
Again, as e<1,υ2 has the same sign as υ0. Hence the other ball also moves forward after collision.
(b) Accordin to principle of conservation of linear momentum,
→p=→p1+→p2
For a general collision, some KE is lost
∴(p22m)>(p212m)+(p222m)
or p2>(p21+p22).
which is possible onely when θ<90∘. figure , which was to be proved.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Long Answer Question
24 Videos
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Advanced Problems For Competitions
20 Videos
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Very Short Anwer Question.
54 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - Assertion- Reason Type Questions
19 Videos
Similar Questions
A ball of mass m moving with velocity v collides head on elastically with another identical ball moving with velocity - V. After collision
View SolutionA ball of mass m moving with a speed u1 collides elasticity with another identical ball moving with velocity u2.
(a) Find the velocities of the balls after collision if the impact is direct.
(b) Find the angle between velocities after collision if they collide obliquely and u2=0
View Solution
Knowledge Check
A billiard ball moving with a speed of 5m/s collides with an identical ball, originally at rest. If the first ball stop dead after collision, then the second ball will move forward with a speed of:
A10ms−1B5ms−1C2.5ms−1D1.0ms−1A billiard ball moving with a speed of 5m/s collides with an identical ball, originally at rest. If the first ball stop dead after collision, then the second ball will move forward with a speed of:
A10m/sB5m/sC2.5m/sD1m/sA ball of mass m moving with a speed v makes a head on collision with an identical ball at rest. The kinetic energy after collision of the balls is three fourth of the original kinetic energy. The coefficient of restitution is
A12B13C1√2D1√3
A ball of mass m moving at a speed v makes a head on inelastic collision with an identical ball at rest. The kinetic energy of the balls after the collision is 34th of the original. Find the coefficient of restitution.


View SolutionA ball of mass m moving with a speed 2v0 collides head-on with an identical ball at rest. If e is the coefficient of restitution, then what will be the ratio of velocity of two balls after collision?
View SolutionA ball of mass M moving with speed v collides perfectly inelastically with another ball of mass m at rest. The magnitude of impulse imparted to the first ball is
View SolutionA ball of mass m is moving with velocity u and collides head on with another identical body at rest . What is the maximum possible loss of kinetic energy due to collision ?
View SolutionA ball of mass m moving with a velocity v undergoes an oblique elastic collision with another ball of the same mass m but at rest. After the collision if the two balls move with the same speeds , the angle between their directions of motion will be:
View Solution
