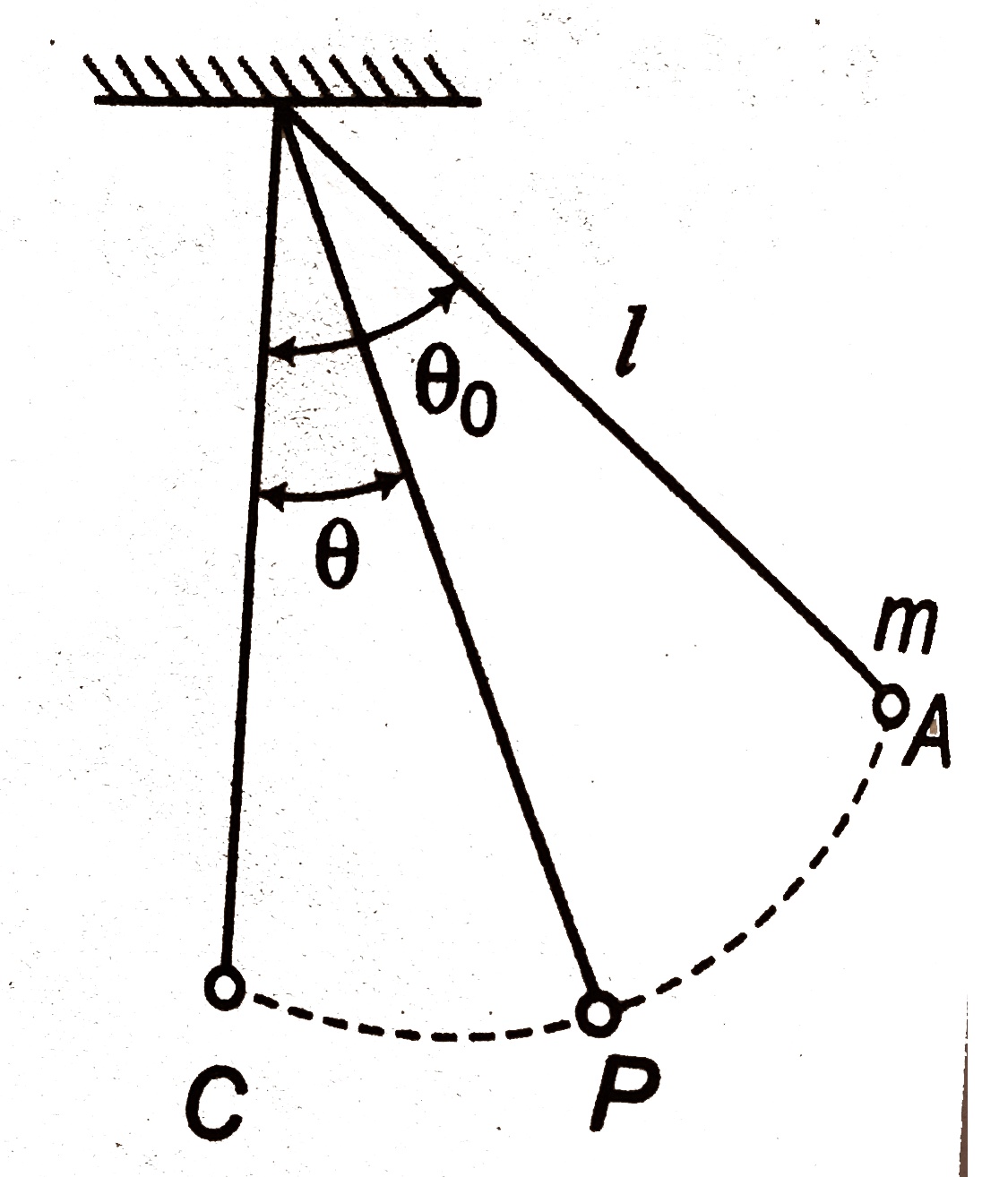
A ball of masws 'm' is released from point A where, Θ0=53∘ . Length of pendulium is 'l' . Find v,ar,at,a , T and Fnet at
(a) point A
(b) point C
(c) pont P where θ=37∘
(a) At pont A
v=0⇒ar=v2R=0 (R=l)
at=gsinθ0=gsin53∘=35g
a=at=45mg
Fnet=ma=45mg
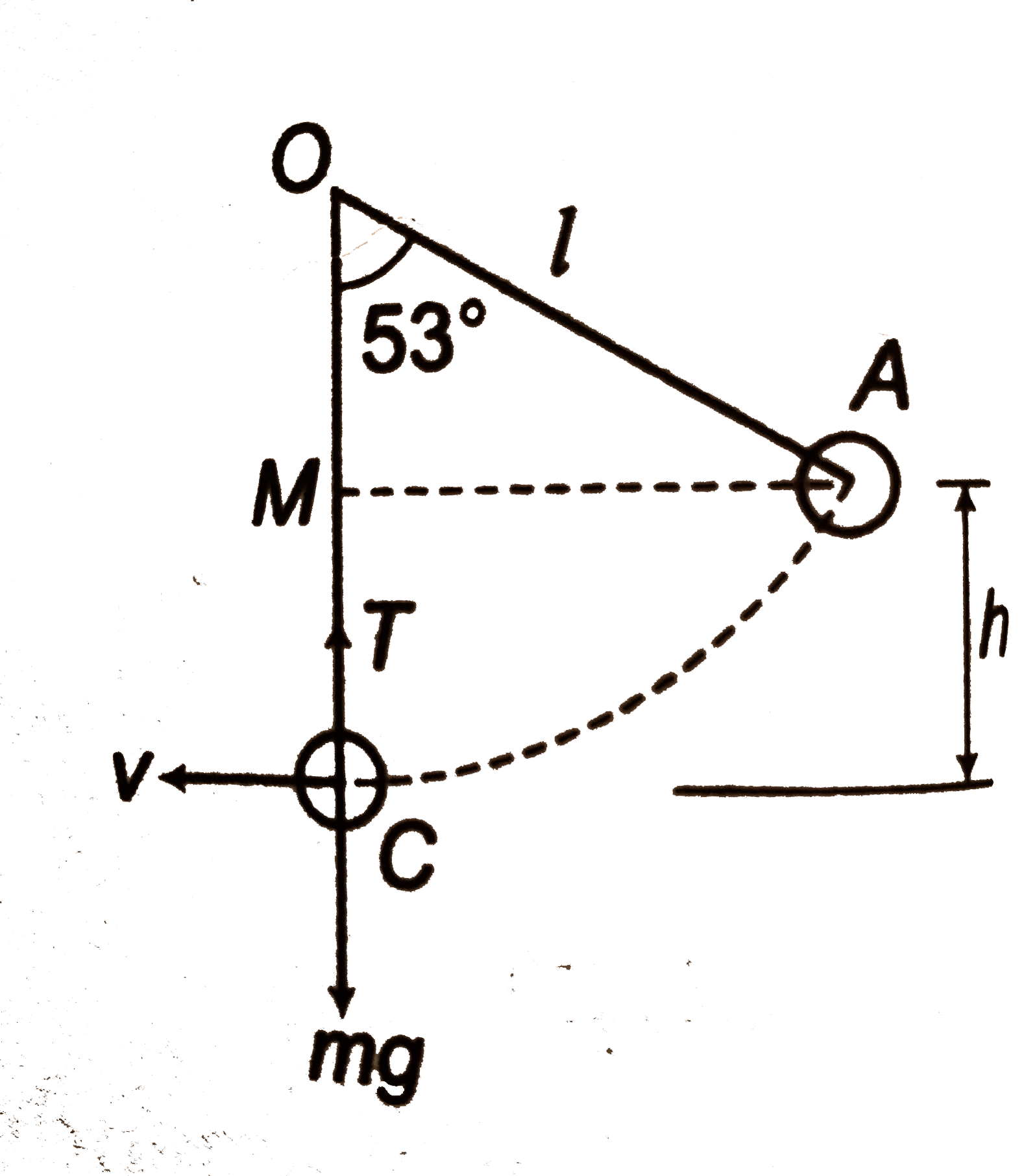
(b) At point C
h=OC−OM=l−lcos53∘
=l−35=25l
v=√2gh=√2g(25l)=√45gl
ar=v2R=(√45gl2)l=45g
at=0
a=ar=45g
T−mg=mv2R=45mg
T=95mg
Fnet=ma=45mg
(c) At point P
h=OM−ON−lcos37∘−lcos53∘
=45l−35l=l5
v=√2gh=√2g(l5)=√25gl
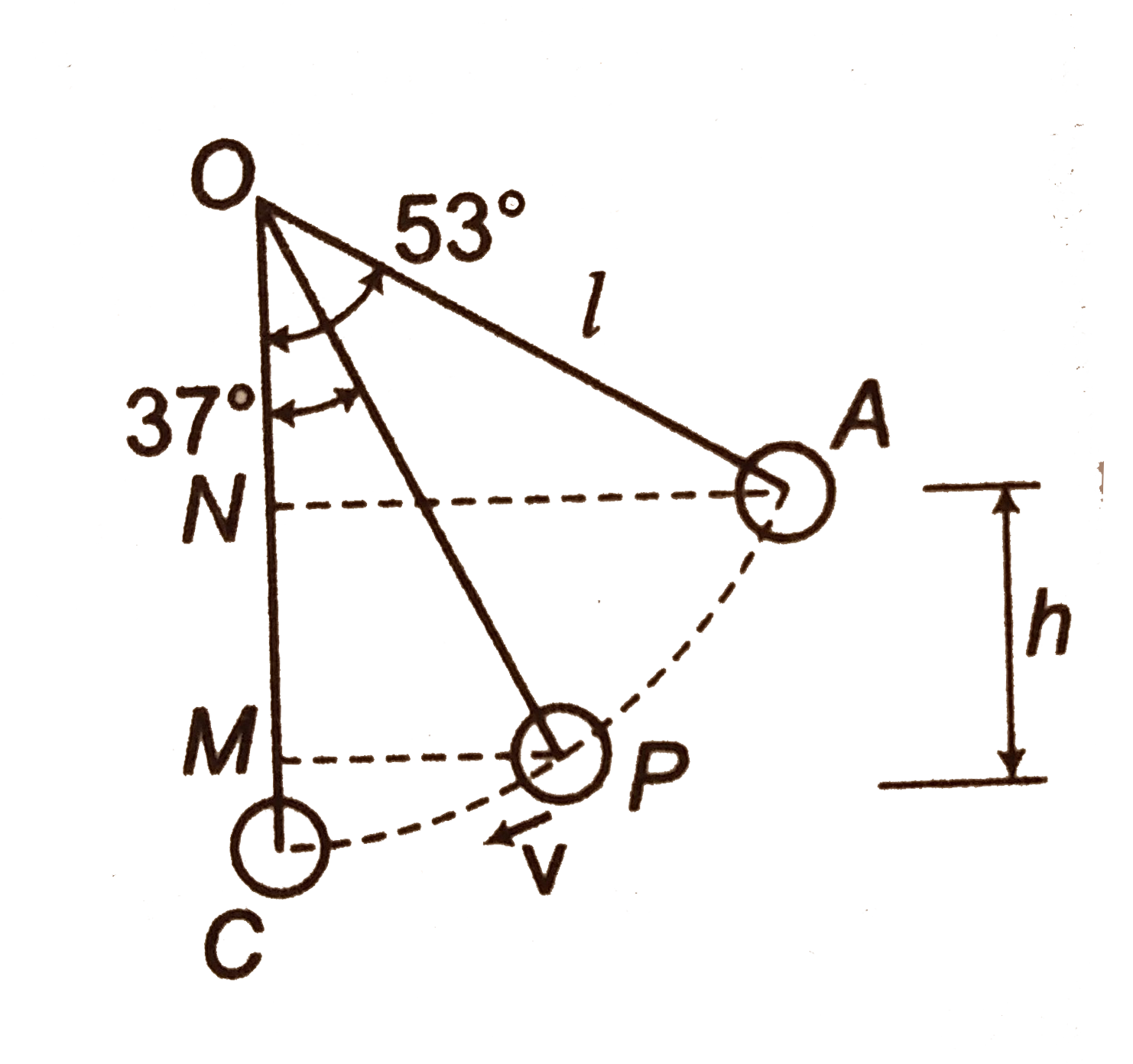
ar=v2R=(√25gl)l=25g
at=gsinθ=gsin37∘=35g
a=√a2r+a2t=√(25g)2+(35g)2=√135g
T−mgcosθ=mar
or T−mgcos37∘=m(25g)
T=65mg
Fnet=ma=√135mg
Topper's Solved these Questions
- CIRCULAR MOTIONBook:DC PANDEY ENGLISHChapter:CIRCULAR MOTIONExercise:Miscellaneous Examples
Explore 5 Videos
- CIRCULAR MOTIONBook:DC PANDEY ENGLISHChapter:CIRCULAR MOTIONExercise:Exercise 10.1
Explore 7 Videos
- CIRCULAR MOTIONBook:DC PANDEY ENGLISHChapter:CIRCULAR MOTIONExercise:Medical entrances s gallery
Explore 19 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONExercise:Level 2 Subjective
Explore 21 Videos
- COMMUNICATION SYSTEMBook:DC PANDEY ENGLISHChapter:COMMUNICATION SYSTEMExercise:Only One Option is Correct
Explore 27 Videos
Similar Questions
In the figure shown, u=√6gR(>√5gR)
Find h,v,ar,at,T and Fnet when
(a) θ=60∘
(b) θ=90∘
(c) θ=180∘
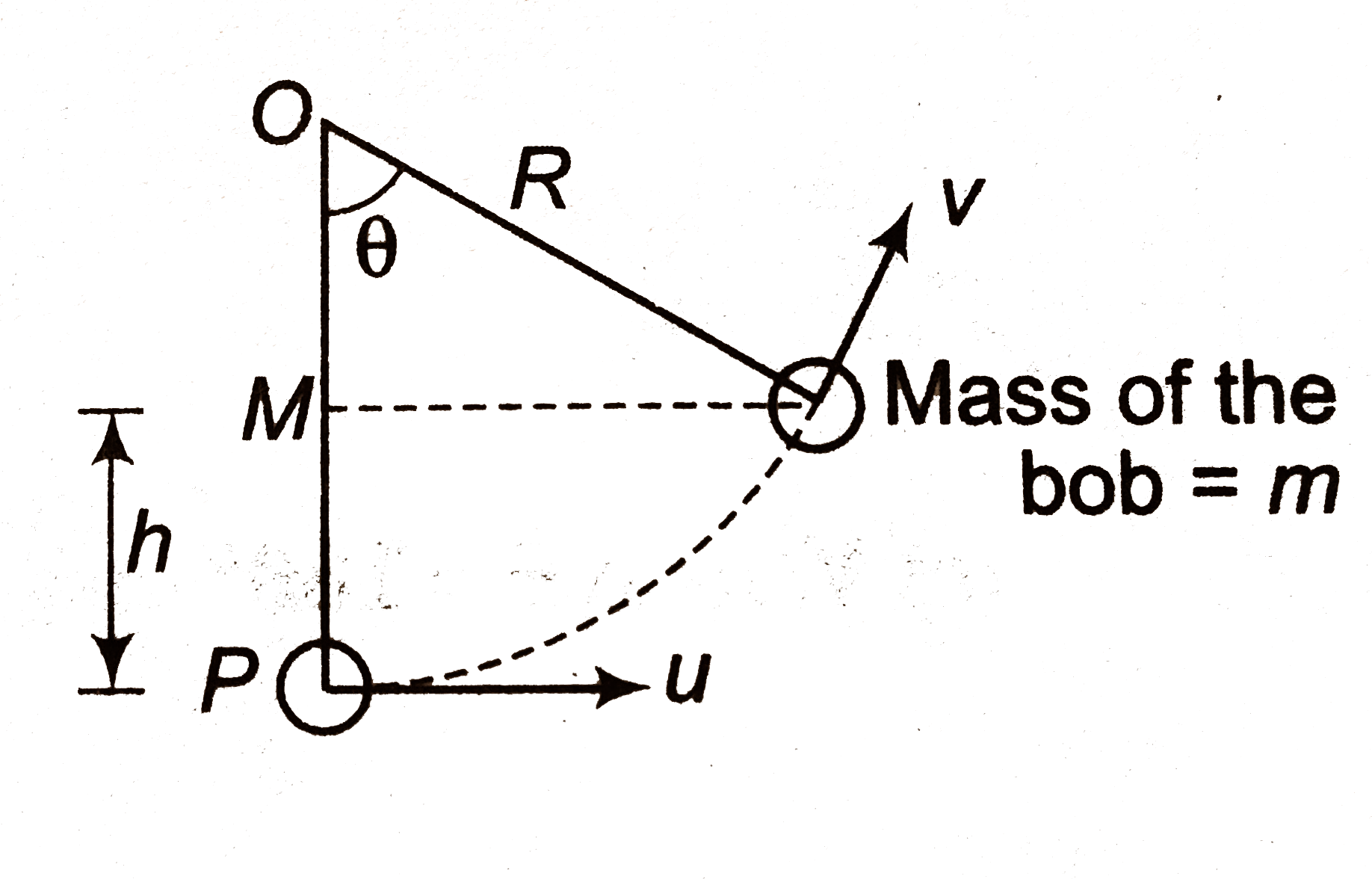
View SolutionA ball of mass 'm' is suspended from a point with a massless string of length l in form of a pendulum. This ball is given a horizontal velocity √4gl at bottom most point. When string makes an angle 60∘ from lower vertical, acat=p. write the value of p2. (g=10m/s2)
View SolutionA ball is released from point A. During its motion ball takes two seconds from B to C Find the time taken by ball from A to C.
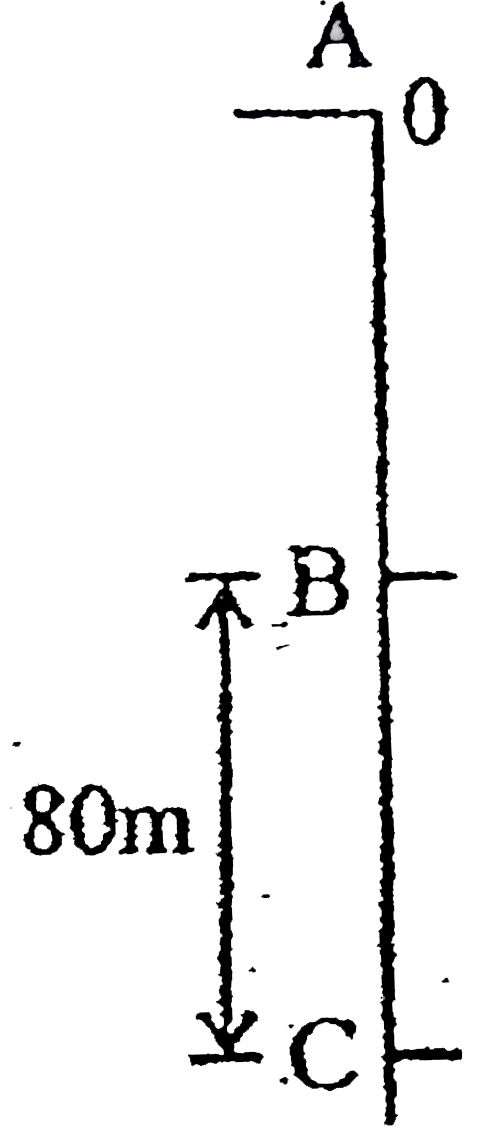 .
.
View SolutionA rigid rod of mass m and lengths l, is being rotated in horizontal plane about a vertical axis, passing through one end A. If TA,TBandTC are the tensions in rod at point A, mid point B and point C of rod repsectively, then
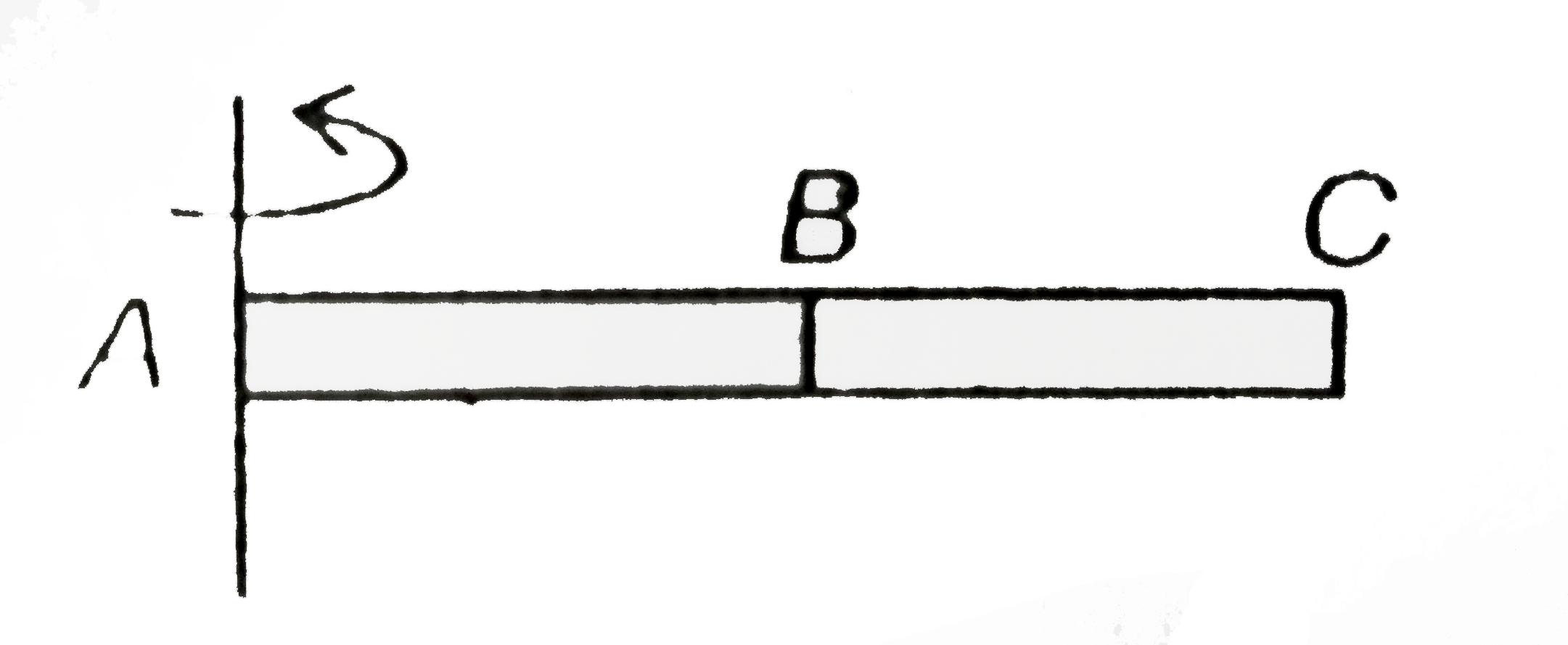
View SolutionA ball of mass 1g and charge 10−8C moves from a point A. Where potential is 600 volt to the point B where potential is zero. Velocity of the ball at the point B is 20cm/s. The velocity of the ball at the point A will be
View SolutionA ball is fired from point P, with an initial speed of 50ms−1 at an angle of 53∘, with the horizontal. At the same time, a long wall ABat200m from point P starts moving toward P with a constant speed of 10ms−1. Find
(a) the time when the ball collides with wall AB.
(b) the coordinate of point C, where the ball xollides, taking point P as origin.
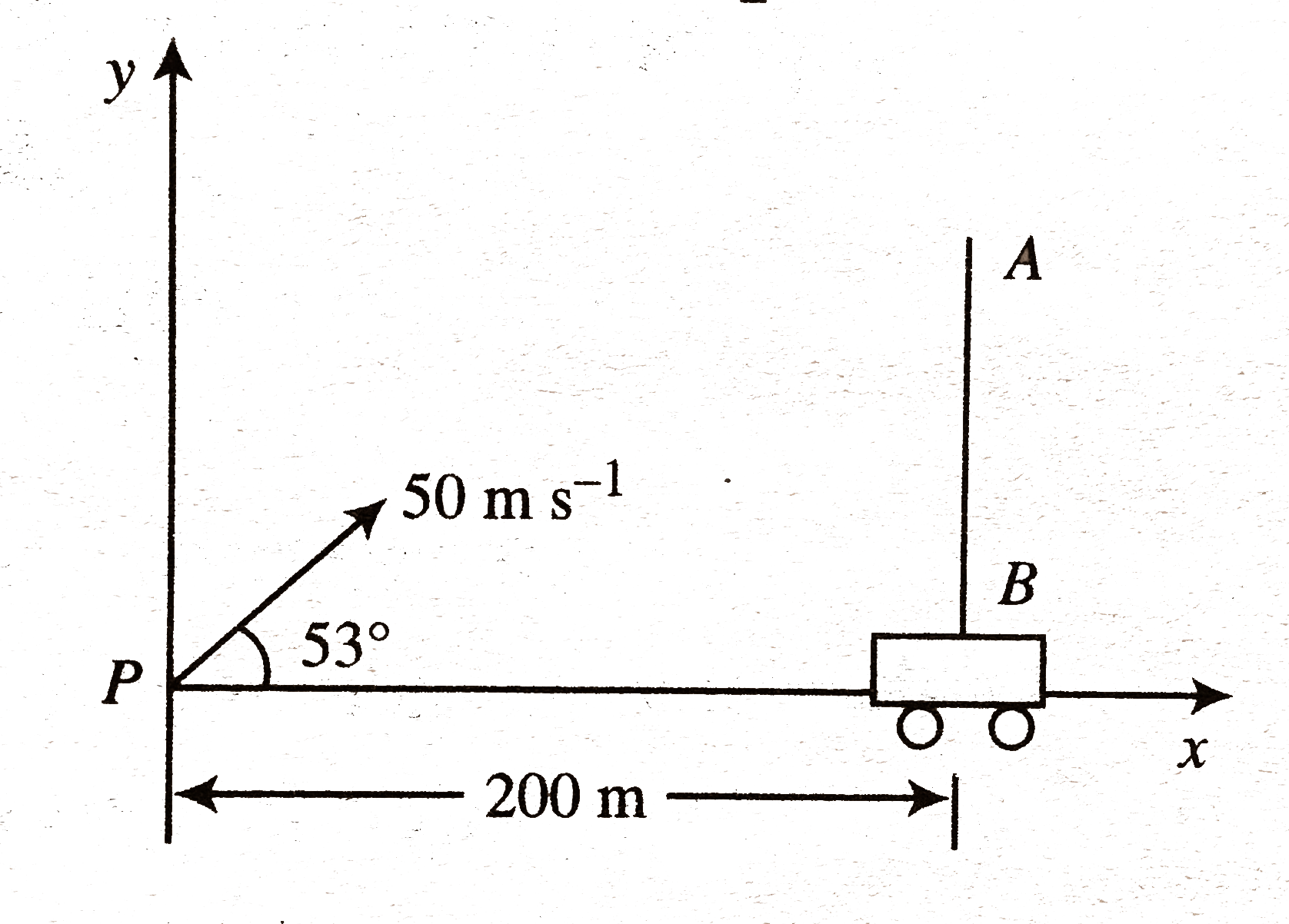 .
.
View SolutionFind the number points where f(θ)=∫1−1sinθdx1−2xcosθ+x2 is discontinous where θ∈[0,2π].
View SolutionTangents drawn from point P to the circle x2+y2=16 make the angles θ1 and θ2 with positive x-axis. Find the locus of point P such that (tanθ1−tanθ2)=c ( constant) .
View SolutionA pendulum bob swing from the point P when the ideal string of length l is horizontal. Find the
(A) Speed
(B) Acceleration of the bob
(C ) Power delivered by gravity at an angular position θ
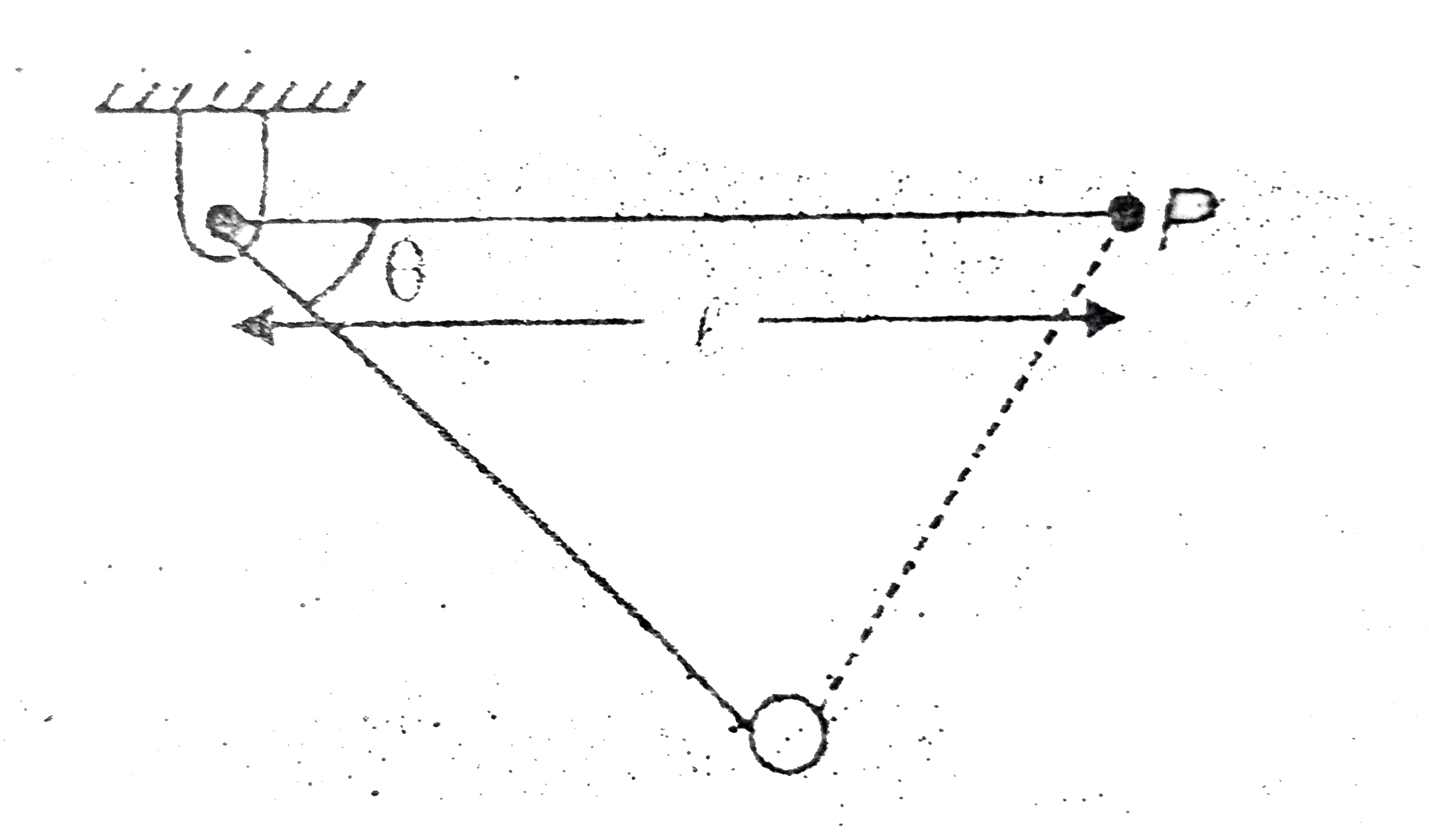
View SolutionFind all point of discontinuity of the function f(t)=1t2+t−2, where t=1x−1
View Solution