A baloon filled with helium rises against gravity increasing its potential energy. The speed of the baloon also increases as it rises. How do you reconcile this with the law of conservation of mechanical energy ? You can neglect viscous drag of air and assume that density of air is constant.
Let V= volume of helium (baloon), m= mass of baloon
ρHe= density of helium, ρair= density of air
Volume V of baloon displaces volume V of air.
∴ The baloon experience as upthrust =V(ρair−ρHe)g=ma=mdυdt ...(i)
Integrating both sides w.r.t. t, we get
v(ρair_ρHe)gt=mυ ...(ii)
From (i), a=V(ρair−ρHe)gm
If the baloon rises to a height h , then from
s=ut=12at2
h=0+12V(ρair−ρHe)gt2m ...(iii)
K.E. of baloon =12mυ2=(mυ)22m=(ρair−ρHe)2V2g2t22m ...using (ii)
=12mυ2=V(ρair−ρHe)g×[(ρair−ρHe)V2mgt2]
using (iii) , we get
12mυ2=V(ρair−ρHe)gh
12mυ2+VρHegh=Vρairgh,i.e., K.E. of baloon + P.E. of baloon = change in P.E. of air.
Hence we conclude that as the Helium baloon goes up, an equal volume of air comes down. Increase in P.E. and K.E. of baloon is at the cost of P.E. of air (that comes down).
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Advanced Problems For Competitions
20 Videos
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - NCERT EXERCISES WITH SOLUTIONS
30 Videos
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Short Answer Question
52 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - Assertion- Reason Type Questions
19 Videos
Similar Questions
Though the kinetic energy of electrons decreases with an increase in the distance from the nucleus, the potential energy of the electron increases. How do you account for this ?
View SolutionUnderline the correct alterntaive:
a) when a conservative force does positivie work on a body, the potential energy of the body increase/decreases/remains unaltered.
work done by a body against friction always results in a loss of its kinetic /potential energy.
c) The rate of change of total momentum of a many-particle system is proportional to the external force/ sum of the internal forces on the system.
d) In an inelastic collision of two bodies, the quantities which do not change after the collision are the total kinetic energy/total linear momentum/total enregy of the system of two bodies.
View Solution
Knowledge Check
Two stars bound together by gravity orbit othe because of their mutual attraction. Such a pair of stars is referred to as a binary star system. One type of binary system is that of a black hole and a companion star. The black hole is a star that has cullapsed on itself and is so missive that not even light rays can escape its gravitational pull therefore when describing the relative motion of a black hole and companion star, the motion of the black hole can be assumed negligible compared to that of the companion.
The orbit of the companion star is either elliptical with the black hole at one of the foci or circular with the black hole at the centre. The gravitational potential energy is given by U=−GmM/r where G is the universal gravitational constant, m is the mass of the companion star, M is the mass of the black hole, and r is the distance between the centre of the companion star and the centre of the black hole. Since the gravitational force is conservative. The companion star and the centre of the black hole, since the gravitational force is conservative the companion star's total mechanical energy is a constant. Because of the periodic nature of of orbit there is a simple relation between the average kinetic energy <K> of the companion star Two special points along the orbit are single out by astronomers. Parigee isthe point at which the companion star is closest to the black hole, and apogee is the point at which is the farthest from the black hole.
Q. For circular orbits the potential energy of the companion star is constant throughout the orbit. if the radius of the orbit doubles, what is the new value of the velocity of the companion star?Ait is 1/2 of the old valueBit is 1/√2 of the old valueCit is the same as old valueDit is double the old valueThe satellites when launched from the earth are not given the orbital velocity initially, a multistage rocket propeller carries the spacecraft up to its orbit and during each stage rocket has been fired to increase the velocity to acquire the desired velocity for a particular orbit. The last stage of the rocket brings the satellite in circular/elliptical (desired) orbit.
Consider a satellite of mass 150kg in a low circular orbit. In this orbit, we cannot neglect the effect of air drag. This air opposes the motion of satellite and hence the total mechanical energy of earth-satellite system decreases. That means the total energy becomes more negative and hence the orbital radius decreases which causes the increase in KE When the satellite comes in the low enough orbit, excessive thermal energy generation due to air friction may cause the satellite to burn up. Based on the above information, answer the following questions.
It has been mentioned in the passage that as r decreases, E decreases but K increases. The increase in K is [E= total mechanical energy, r= orbital radius, K= kinetic energy ]Adue to increase in gravitational PEBdue to decrease in gravitational PECdue to work done by air friction forceDboth (b) and (c)The satellites when launched from the earth are not given the orbital velocity initially, a multistage rocket propeller carries the spacecraft up to its orbit and during each stage rocket has been fired to increase the velocity to acquire the desired velocity for a particular orbit. The last stage of the rocket brings the satellite in circular/elliptical (desired) orbit.
Consider a satellite of mass 150kg in a low circular orbit. In this orbit, we cannot neglect the effect of air drag. This air opposes the motion of satellite and hence the total mechanical energy of earth-satellite system decreases. That means the total energy becomes more negative and hence the orbital radius decreases which causes the increase in KE When the satellite comes in the low enough orbit, excessive thermal energy generation due to air friction may cause the satellite to burn up. Based on the above information, answer the following questions.
If due to air drag, the orbital radius of the earth decreases from R to R−△R,△R<<R, then the expression for increase in the orbital velocity △v isA△r2√GMR3B−△R2√GM2C△R√GMR3D−△R√GMR3
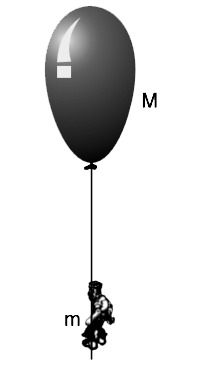
A hot air balloon (mass M) has a passenger (mass m) and is stationary in the mid air. The passenger climbs out and slides down a rope with constant velocity u relative to the balloon.
(a) Show that when the passenger is sliding down, there is no change in mechanical energy (kinetic + gravitational potential energy) of the system (Balloon + passenger). Calculate the speed of balloon.
(b) Calculate the power of the buoyancy force on the system when the man is sliding. For easy calculation, assume that volume of man is negligible compared to the balloon.
(c) If buoyancy force is doing positive work, where is this work done lost? You have proved that sum of kinetic and potential energy of the system remains constant
View SolutionUnderline the correct alterntaive:
a) when a conservative force does positivie work on a body, the potential energy of the body increase/decreases/remains unaltered.
work done by a body against friction always results in a loss of its kinetic /potential energy.
c) The rate of change of total momentum of a many-particle system is proportional to the external force/ sum of the internal forces on the system.
d) In an inelastic collision of two bodies, the quantities which do not change after the collision are the total kinetic energy/total linear momentum/total enregy of the system of two bodies.
View SolutionA gun fires a large number of bullets upward. Due to shaking of hands some bullets deviate as much as 1° from the vertical. The muzzle speed of the gun is 150m/s and the height of gun above the ground is negligible. The radius of the head of the person firing the gun is 10cm. You can assume that acceleration due to gravity is nearly constant for heights involved and its value is g=10m/s2. The gun fires 1000 bullets and they fall uniformly over a circle of radius r. Neglect air resistance. You can use the fact sinθ≅θ when θ is small.
(a) Find the approximate value of r. (b) What is the probability that a bullet will fall on the person’s head who is firing?
View SolutionAn accelration produces a narrow beam of protons, each having an initial speed of v0. The beam is directed towards an initially uncharges distant metal sphere of radius R and centered at point O. The initial path of the beam is parallel to the axis of the sphere at a distance of (R/2) from the axis, as indicated in the diagram.

The protons in the beam that collide with the sphere will cause it to becomes charged. The subsequentpotential field at the accelerator due to the sphere can be neglected. The angular momentum of a particle is defined in a similar way to the moment of a force. It is defined as the moment of its linear momentum, linear replacing the force. We may assume the angular momentum of a proton about point O to be conserved. Assume the mass of the proton as mP and the charge on it as e. Given that the potential of the sphere increases with time and eventually reaches a constant velue.
The total energy (E) of a proton in the beam travelling with seed v at a distance of r(r≥R) from point O. Assuming that the sphere has acquired an electrostatic charge Q is
View SolutionIf a body moves through a liquid or a gas then the fluid applies a force on the body which is called drag force. Direction of the drag force is always opposite to the motion of the body relative to the fluid. At low speeds of the body, drag frog (FP) is directly proportional to the speed.
FD=kv
What K is a proportionally constant and it depends upon the dimension of the body moving in air at relatively high speeds, the drag force applied by air an the body is proportional to v2
Where this proportionally constant K can be given by
K2ρCA
Where ρ is the density of air
C is another constant givig the drag property of air
A is area of cross-section of the body
Consider a case an object of mass m is released from a height h and it falls under gravity. As it's speed increases the drag force starts increasing on the object. Due to this at some instant, the object attains equilibrium. The speed attained by the body at this instant is called "terminal speed" of the body.
Assume that the drag force applied by air on the body follows the relation FD=kv,neglect the force by buoyancy applied by air on the body then answer the following questions.
What is the terminal speed of the object ?
View Solution
