A bead can slide on a smooth circular wire frame of radius r which is fixed in a vertical plane. The bead is displaced slighty from the highest point of the wire frame. The speed of the bead subsequently as a function of the angle θ made by the bead with the verticle line is
√2gr
√2gr(1−sinθ)
√2gr(1−cosθ)
2√gr
The correct Answer is:C
To solve the problem of finding the speed of the bead as a function of the angle θ made with the vertical line, we can follow these steps:
Step 1: Understand the Setup
The bead is on a smooth circular wire of radius r and is displaced slightly from the highest point. As it moves down, it loses potential energy and gains kinetic energy.
Step 2: Determine the Height h
When the bead is at an angle θ with the vertical, the height h of the bead above the lowest point of the circular path can be expressed as:
h=r(1−cosθ)
This equation comes from the geometry of the circle, where the vertical height from the top to the bead's position is given by the difference in the radius and the vertical component of the radius at angle θ.
Step 3: Apply Conservation of Energy
According to the principle of conservation of energy, the decrease in potential energy equals the increase in kinetic energy. The potential energy lost when the bead moves from the top to the height h is:
ΔPE=mgh=mg⋅r(1−cosθ)
The kinetic energy gained by the bead is:
KE=12mv2
Setting the decrease in potential energy equal to the increase in kinetic energy gives us:
mgr(1−cosθ)=12mv2
Step 4: Simplify the Equation
We can cancel m from both sides (assuming m≠0):
gr(1−cosθ)=12v2
Step 5: Solve for v
To find the speed v, we can rearrange the equation:
v2=2gr(1−cosθ)
Taking the square root of both sides gives us:
v=√2gr(1−cosθ)
Conclusion
Thus, the speed of the bead as a function of the angle θ is:
v=√2gr(1−cosθ)
The bead is on a smooth circular wire of radius r and is displaced slightly from the highest point. As it moves down, it loses potential energy and gains kinetic energy.
When the bead is at an angle θ with the vertical, the height h of the bead above the lowest point of the circular path can be expressed as:
h=r(1−cosθ)
This equation comes from the geometry of the circle, where the vertical height from the top to the bead's position is given by the difference in the radius and the vertical component of the radius at angle θ.
According to the principle of conservation of energy, the decrease in potential energy equals the increase in kinetic energy. The potential energy lost when the bead moves from the top to the height h is:
ΔPE=mgh=mg⋅r(1−cosθ)
The kinetic energy gained by the bead is:
KE=12mv2
Setting the decrease in potential energy equal to the increase in kinetic energy gives us:
mgr(1−cosθ)=12mv2
We can cancel m from both sides (assuming m≠0):
gr(1−cosθ)=12v2
To find the speed v, we can rearrange the equation:
v2=2gr(1−cosθ)
Taking the square root of both sides gives us:
v=√2gr(1−cosθ)
Thus, the speed of the bead as a function of the angle θ is:
v=√2gr(1−cosθ)
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRANCE SPECIAL QUESTIONS
16 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MATCH THE COLUMNS
7 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - CHECK POINT 6.3
10 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - E Integer Type Questions
11 Videos
Similar Questions
A small mass m starts from rest and slides down the smooth spherical surface of F. Assume zero potential energy at the top. Find
(a) the change in potential energy,
(b) the kinetic energy,
(c) the speed ot the mass as a function of the angle θ made by the radius through the mass with the vertical.
View SolutionTwo beads A and B of masses m1 and m2 respectively, are threaded on a smooth circular wire of radius a fixed in a vertical plane. B is stationary at the lowest point when A is gently dislodged from rest at the highest point. A collided with B at the lowest point. The impulse given to B due to collision is just great enough to carry it to the level of the centre of the circle while A is immediately brought to rest by the impact.
 What is the coefficient of restituting between the beads?
What is the coefficient of restituting between the beads?
View SolutionTwo beads A and B of masses m1 and m2 respectively, are threaded on a smooth circular wire of radius a fixed in a vertical plane. B is stationary at the lowest point when A is gently dislodged from rest at the highest point. A collided with B at the lowest point. The impulse given to B due to collision is just great enough to carry it to the level of the centre of the circle while A is immediately brought to rest by the impact.

If m2 again comes down and collides with m1 then after the collision
View SolutionTwo beads A and B of masses m1 and m2 respectively, are threaded on a smooth circular wire of radius a fixed in a vertical plane. B is stationary at the lowest point when A is gently dislodged from rest at the highest point. A collided with B at the lowest point. The impulse given to B due to collision is just great enough to carry it to the level of the centre of the circle while A is immediately brought to rest by the impact.

Find the ratio m1:m2
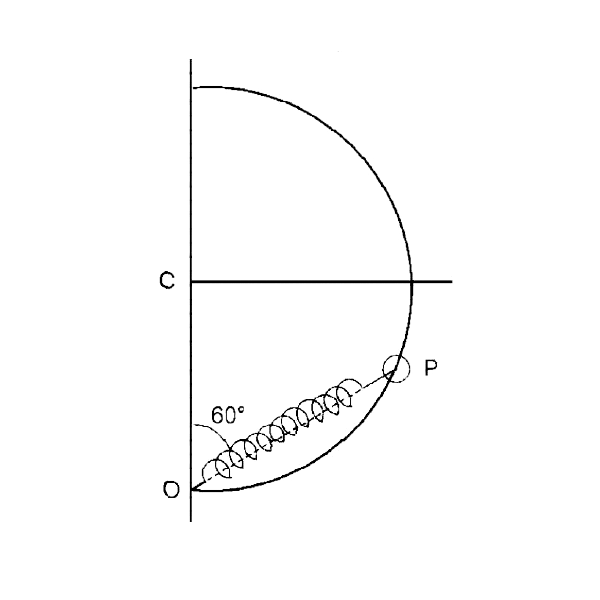
View SolutionA smooth semicircular wire-track of radius R is fixed in a vertical plane. One end of a massless spring of natural length 3R/4 is attached to the lowest point O of the wire-track. A small ring of mass m, which can slide on the track, is attached to the other end of the spring. The ring is held staionary at point P such that the spring makes an angle of 60∘ with the vertical. The spring constant K=mg/R. Consider the instant when the ring is released, and (i) draw the free body diagram of the ring, (ii) determine the tangential acceleration of the ring and the normal reaction.
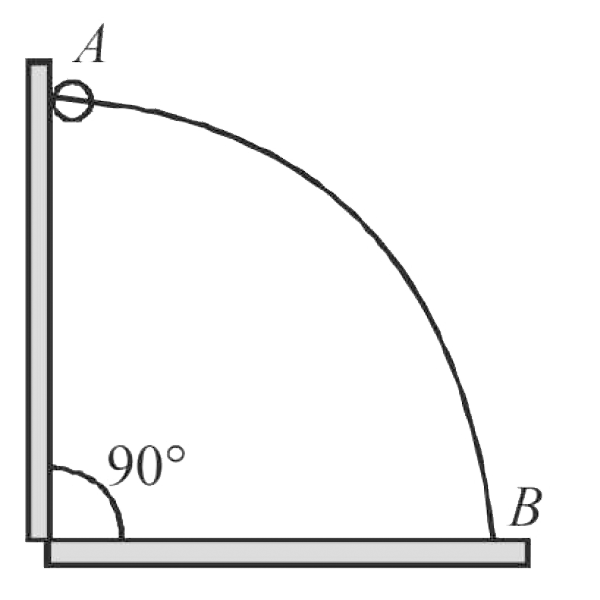
View SolutionA wire, which passes through the hole in a small bead, is bent in the form of quarter of a circle. The wire is fixed vertically on ground as shown in the figure. The bead is released from near the top of the wire and it slides along the wire without friction. As the bead moves from A to B, the force it applies on the wire is
View SolutionA particle is given an intial speed u inside a smooth spherical shell of radius R so that it is just able to complete the circle. Acceleration of the particle, when its velocity is vertical, is

View SolutionA smooth circular tube of radius R is fixed in a vertical plane. A particle is projectid from its lowest point with a velocity just sufficient to carry it to th ehigest point. Show that the time taken by the particle to rach the end of the horizontal diameter is (√Rg)In(1+√2) .
Hint : ∫secθ.dthη=In(secθ+tanθ)
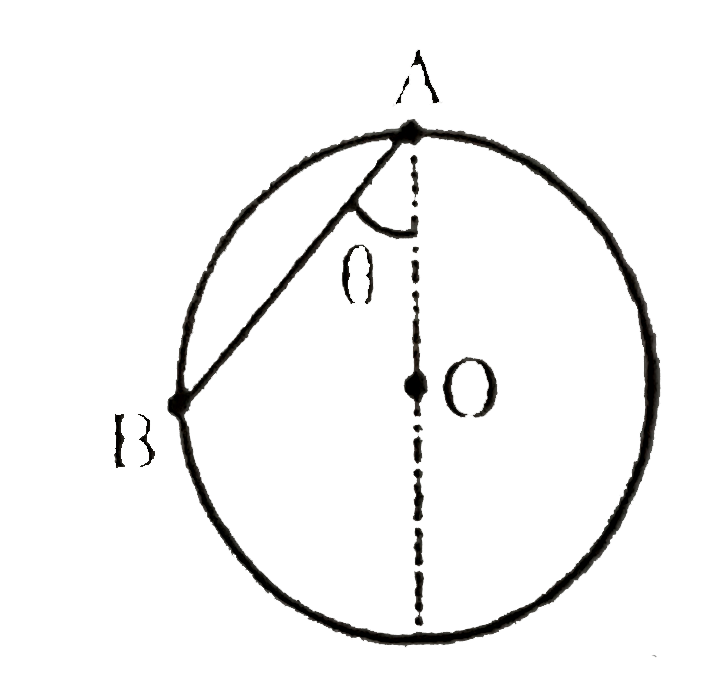
View SolutionA bead is free to slide down a smooth wire tightly stretched between points A and on a verticle circle of radius R. if the bead starts from rest at 'A', the highest point on the circle, its velocity when it arrives at B is :-
View SolutionA bead can slide on a smooth straight wire and a particle of mass m attached to the bead by a light string of length L. The particle is held in contact with the wire and with the string taut and is then let fall. If the bead has mass 2m then when the string makes an angle θ with the wire, the bead will have slipped a distance.

View Solution
