A binary star system consists of two stars A and B which have time period TAandTB,radiusRBa and mass MaandMB. Then
ifT_Agt T_B then R_A gt R_B
ifTA>TBthenMA>MB
(TATB)2=(RARB)3
TA=TB
The correct Answer is:D
To solve the problem regarding the binary star system consisting of two stars A and B, we need to analyze the relationship between their time periods, masses, and radii. Here’s a step-by-step solution:
Step 1: Understanding the System
In a binary star system, two stars orbit around their common center of mass. The gravitational force between the two stars provides the necessary centripetal force for their circular motion.
Step 2: Gravitational Force
The gravitational force F between the two stars can be expressed using Newton's law of gravitation:
F=GMAMBD2
where G is the gravitational constant, MA and MB are the masses of stars A and B, respectively, and D is the distance between the two stars.
Step 3: Centripetal Force
For a star to move in a circular path, the required centripetal force Fc is given by:
Fc=mω2r
where m is the mass of the star, ω is the angular velocity, and r is the radius of the circular path.
Step 4: Equating Forces
Since the gravitational force provides the centripetal force for both stars, we can set the gravitational force equal to the centripetal force for each star:
GMAMBD2=MAω2RA(for star A)
GMAMBD2=MBω2RB(for star B)
Step 5: Relationship Between Angular Velocity and Time Period
The angular velocity ω is related to the time period T by the formula:
ω=2πT
Thus, we can express the time periods for both stars:
TA=2πωAandTB=2πωB
Step 6: Conclusion
Since the angular velocities ωA and ωB are equal for both stars (as they orbit around the center of mass), we conclude that:
TA=TB
This means the time periods of both stars are the same.
Final Answer
Thus, the correct option is that the time periods of the two stars are equal:
TA=TB
In a binary star system, two stars orbit around their common center of mass. The gravitational force between the two stars provides the necessary centripetal force for their circular motion.
The gravitational force F between the two stars can be expressed using Newton's law of gravitation:
F=GMAMBD2
where G is the gravitational constant, MA and MB are the masses of stars A and B, respectively, and D is the distance between the two stars.
For a star to move in a circular path, the required centripetal force Fc is given by:
Fc=mω2r
where m is the mass of the star, ω is the angular velocity, and r is the radius of the circular path.
Since the gravitational force provides the centripetal force for both stars, we can set the gravitational force equal to the centripetal force for each star:
GMAMBD2=MAω2RA(for star A)
GMAMBD2=MBω2RB(for star B)
The angular velocity ω is related to the time period T by the formula:
ω=2πT
Thus, we can express the time periods for both stars:
TA=2πωAandTB=2πωB
Since the angular velocities ωA and ωB are equal for both stars (as they orbit around the center of mass), we conclude that:
TA=TB
This means the time periods of both stars are the same.
Thus, the correct option is that the time periods of the two stars are equal:
TA=TB
Topper's Solved these Questions
Similar Questions
A binary star system consists of two stars A and B which have time periods TA and TB radius RA and RB and masses mA and mB which of the three quantities are same for the stars. Justify.
View SolutionConsider a binary star system consisting of two stars of masses M1 and M2 separated by a distance of 30AU with a period of revolution equal to 30 years. If one of the two stars is 5 times farther from the centre of mass than the other, show that the masses of the two stars are 5 and 25 times that of the sun.
View Solution
Knowledge Check
- Question 1 - Select One
A binary star system consists of two stars. One star has twice the mass of the other. The star rotates about their common centre of mass. Which of the following statement is correct?
AStar having the smaller mass has twice angular momentum compared to heavier star.BBoth stars have same angular momentum about centre of mass.CBoth stars have same linear speed.DBoth the stars have same kinetic energy. - Question 2 - Select One
A binary star system consists of two stars of masses M1 and M2 revolving in circular orbits of radii R1 and R2 respectively. If their respective time periods are T1 and T2, then
AT1>T2 if R1>R2BT1>T2 if M1>M2CT1=T2DT1T2=(R1R2)3/2 - Question 3 - Select One
An isolated triple star systerm consists of two identical stars, each of mass m and f fixed star of mass M. They revolve around the central star in the same circular orbit to radius r. The orbiting stars are always at opposite ends of a diameter of the orbit. The time period of revolution of each star around the fixed is equal to:
 A4πr3/2√G(4M+m)B2πr3/2√GMC2πr3/2√G(M+m)D4πr3/2√G(M+m)
A4πr3/2√G(4M+m)B2πr3/2√GMC2πr3/2√G(M+m)D4πr3/2√G(M+m)
A certain triple-star system consists of two stars, each of mass m, revolving about a central star, mass M, in the same circular orbit. The two stars stay at opposite ends of a diameter of the circular orbit, see figure. Derive an expression for the period of revolution of the stars, the radius of the orbit is r.
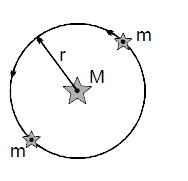 View Solution
View SolutionA binary star consists of two stars A(mass2.2Ms) and B (mass11Ms) where Ms is the mass of the sun, they are separted by distane d and are rotating about their center of mass, which is stationary. The ratio of the total angular momentum of the binary to the angular momentum of star B about the centre of mass is
View Solution_S01_033_Q01.png)
A triple star ststem consists of two stars each of mass m in the same circular orbit about central star with mass M=2×1033kg. The two outer stars always lie at opposite ends of a diameter of their common circular orbit the radius of the circular orbit is r=1011 m and the orbital period each star is 1.6×107s [take π2=10 and G=203×10−11Mn2kg−2]
Q. The mass m of the outer stars is:View Solution_S01_035_Q01.png)
A triple star ststem consists of two stars each of mass m in the same circular orbit about central star with mass M=2×1033kg. The two outer stars always lie at opposite ends of a diameter of their common circular orbit the radius of the circular orbit is r=1011 m and the orbital period each star is 1.6×107s [take π2=10 and G=203×10−11Mn2kg−2]
Q. The total machanical energy of the system isView Solution_S01_034_Q01.png)
A triple star ststem consists of two stars each of mass m in the same circular orbit about central star with mass M=2×1033kg. The two outer stars always lie at opposite ends of a diameter of their common circular orbit the radius of the circular orbit is r=1011 m and the orbital period each star is 1.6×107s [take π2=10 and G=203×10−11Mn2kg−2]
Q. The orbital velocity of each star isView Solution

