a block of weight 100 N is slowly slid up on a smooth incline of inclination by as person. Calculate the work done by the person in moving the block through a distance of 2.0 m, if the drivin force is a. parallel to the inclne and b. in the horizontla direction.
The correct Answer is:A, B
To solve the problem, we need to calculate the work done by a person in moving a block of weight 100 N up a smooth incline of inclination 37∘ through a distance of 2.0 m. We will consider two cases: (a) when the driving force is parallel to the incline, and (b) when the driving force is in the horizontal direction.
1. Identify the Forces Acting on the Block:
- The weight of the block W=100N.
- The angle of inclination θ=37∘.
- The component of the weight acting down the incline is Wsin(θ).
- The component of the weight acting perpendicular to the incline is Wcos(θ).
2. Calculate the Component of Weight Down the Incline:
Wsin(37∘)=100sin(37∘)
Using sin(37∘)≈0.6:
Wsin(37∘)=100×0.6=60N
3. Determine the Force Required to Move the Block Up:
- Since the block is moved slowly up the incline, the person must apply a force F that is slightly greater than 60N to overcome the component of weight acting down the incline. We can assume F≈60N for our calculations.
4. Calculate the Work Done:
- The work done Wd is given by:
Wd=F×d×cos(0∘)
where d=2m is the distance moved and cos(0∘)=1.
Wd=60N×2m×1=120J
Case (b): Driving Force in the Horizontal Direction
1. Identify the Forces Acting on the Block:
- The weight of the block remains W=100N.
- The horizontal force Fh is applied.
2. Calculate the Component of the Horizontal Force:
- The horizontal force has a component that acts along the incline:
Fhcos(37∘)=100sin(37∘)
Using cos(37∘)≈0.8:
Fhcos(37∘)=100×0.6=60N
Therefore,
Fh=60Ncos(37∘)=60N0.8=75N
3. Calculate the Work Done:
- The work done when applying the force horizontally is given by:
Wd=Fh×d×cos(53∘)
where d=2m and cos(53∘)≈0.6.
Wd=75N×2m×0.6=90J
Final Answers
- Work done when the force is parallel to the incline: 120J
- Work done when the force is horizontal: 90J
- The weight of the block W=100N.
- The angle of inclination θ=37∘.
- The component of the weight acting down the incline is Wsin(θ).
- The component of the weight acting perpendicular to the incline is Wcos(θ).
Wsin(37∘)=100sin(37∘)
Using sin(37∘)≈0.6:
Wsin(37∘)=100×0.6=60N
- Since the block is moved slowly up the incline, the person must apply a force F that is slightly greater than 60N to overcome the component of weight acting down the incline. We can assume F≈60N for our calculations.
- The work done Wd is given by:
Wd=F×d×cos(0∘)
where d=2m is the distance moved and cos(0∘)=1.
Wd=60N×2m×1=120J
- The weight of the block remains W=100N.
- The horizontal force Fh is applied.
- The horizontal force has a component that acts along the incline:
Fhcos(37∘)=100sin(37∘)
Using cos(37∘)≈0.8:
Fhcos(37∘)=100×0.6=60N
Therefore,
Fh=60Ncos(37∘)=60N0.8=75N
- The work done when applying the force horizontally is given by:
Wd=Fh×d×cos(53∘)
where d=2m and cos(53∘)≈0.6.
Wd=75N×2m×0.6=90J
- Work done when the force is parallel to the incline: 120J
- Work done when the force is horizontal: 90J
Topper's Solved these Questions
Similar Questions
A 2-kg body is sliding up an inclined plane of inclination 30∘. Find the work done by the force of gravity m moving the body through I m.
View SolutionA block of mass 250 g slides down an incline of inclination 370 with a uniform speed. Find the work done against the friction as the block slides through 1.0 m.
View Solution
Knowledge Check
How much work is done by a force of 10 N in moving an object through a distance of 1 m in the direction of the force ?
A20JB100JC15JD10JA block of mass 10kg is pushed up on a smooth incline plane of inclination 30∘, so that it has acceleration 2m/s2. The applied force is
A50 NB60 NC70 ND80 NA block of mass 20 kg is moving on a smooth surface by pulling through a light string of tension 10√2 N. Find the work done by string on the block for a displacement of 2 m along the surface.
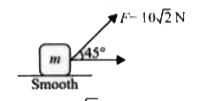 A10JB20√2JC20JD10√2J
A10JB20√2JC20JD10√2J
A block of mass 5.0 kg slides down an incline of inclination 300 and length 10 m. The work done by the force of gravity (in joule) is
View SolutionA block of weight 1 N rests on a inclined plane of inclination θ with the horizontal. The coefficient of friction between the block and the inclined plane is μ. The minimum force that has to be applied parallel to the inclined plane to make the body just move up the plane is
View SolutionA block of mass m is placed on an inclined plane which is moving with constant velocity v in horizontal direction as shown in figure. Then find out work done by the friction in time t if the block is at rest with respect to the incline plane.
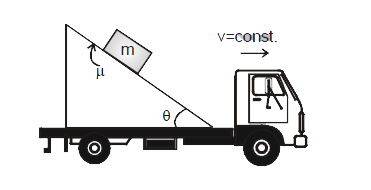
View SolutionA man of weight 60 kg f is carrying a load of 20 kg of on his head . He moves a distance of 25 m up an incline of 1 in 12 . Calculate the work done by him .
View SolutionA body is mass 300kg is moved through 10m along a smooth inclined plane of inclination angle 30∘. The work done in moving (in joules) is (g=9.8ms−2).
View Solution
